OPERATIONS WITH RADICALS

Addition, subtraction, multiplication and division of radical terms can be performed by laws of radicals. Let us see the rules one by one.

Addition and Subtraction of Radicals

Addition and subtraction of two or more radical terms can be performed with like radicands only. 

Like radicand means a number which is inside root sign must be same but the number outside the radical may be different.

For example,

5√2 + 3√2 = 8√2

Here 5√2 and 3√2 are like radical terms.

Multiplying and Dividing Radicals

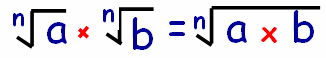
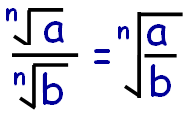
Whenever we have two or more radical terms which are multiplied with same index, then we can put only one radical and multiply the terms inside the radical. 

Whenever we have two or more radical terms which are dividing with same index, then we can put only one radical and divide the terms inside the radical. 

Problem 1 :

Simplify the following radical expression 

7√30 + 2√75 + 5√50 

Solution :

                    = 7 √30 + 2 √75 + 5 √50 

First we have to split the given numbers inside the radical as much as possible.

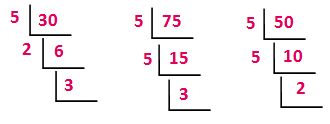
=  √(5 x 2 x 3) + √(5 x 5 x 3) + √(5 x 5 x 2)

Here we have to keep √30 as it is.

=  √30 + 5 √3 + 5 √2

Problem 2 :

Simplify the following √5 x √18

Solution :

  = √5 x √18

According to the laws of radical,

= √(5 x 18) ==> √(5 x 3 x 3)  ==> 3 √5

Problem 3 :

Simplify the following

∛7 x ∛8

Solution :

  =  ∛7 x ∛8

According to the laws of radical,

  = ∛(7 x 8) ==> ∛(7 x 2 x 2 x 2) ==> 2 ∛7 x 2 ==> 2 ∛14

Problem 4 :

Simplify the following 

3√35 ÷ 2√7

Solution :

  = 3√35 ÷ 2√7

According to the laws of radical,

  =  (3/2) √(35/7) ==> (3/2)√5

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.