ORDERING TRIANGLE SIDES AND ANGLES
Angle Side Relationship
In a triangle the sides opposite to the angles are in the same order as the angles and vice versa. If we order the three angles of a triangle from least to largest, the sides opposite to the angles will be in the same order.
Thus the side opposite to the largest angle will be the longest side. The same pattern can be applied for the angles when the sides are given, The angle opposite to the smallest side will be the smallest angle.
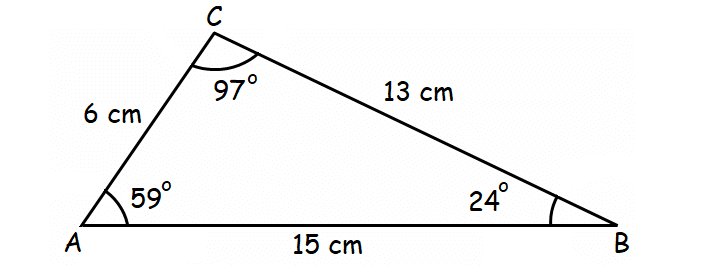
In the triangle above the smallest side is AC (= 6 cm) which is opposite to the smallest angle 24°, the next longer side is BC (= 13 cm) which is opposite to the next larger angle 24° and the longest side is AB (= 15 cm) which is opposite to 97°.
Ordering of the sides from shortest to longest :
AC, BC, AB (or) 6 cm, 13 cm, 15 cm
Ordering of the angles from smallest to largest :
∠B, ∠A, ∠C (or) 24°, 59°, 97°
Example 1 :
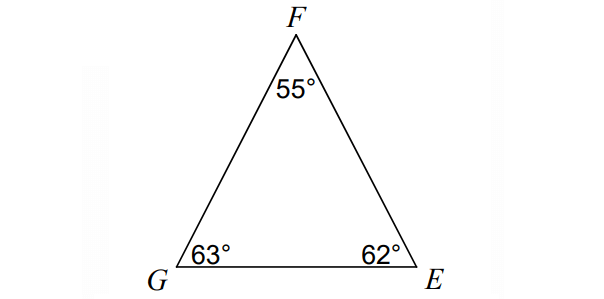
In ΔGEF below, order the sides from shortest to longest.
Answer :
Order the angles from smallest to largest :
55°, 62°, 63°
The side opposite to the smallest angle is the shortest side and the side opposite to the largest angle is the longest side.
side opposite to 55° ----> GE
side opposite to 62° ----> GF
side opposite to 63° ----> EF
Order of the sides from shortest to longest :
GE, GF, EF
Example 2 :
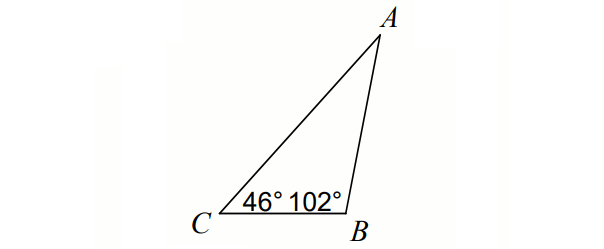
In ΔABC below, order the sides from longest to shortest.
Answer :
In ΔABC above,
m∠A + m∠B + m∠C = 180°
Substitute m∠B = 102° and m∠C = 46°.
m∠A + 102° + 46° = 180°
m∠A + 148° = 180°
Subtract 148° from each side.
m∠A = 32°
Order the angles from smallest to largest :
32°, 46°, 102°
side opposite to 102° ----> AC
side opposite to 46° ----> AB
side opposite to 32° ----> BC
Order of the sides from longest to shortest :
AC, AB, BC
Example 3 :
In ΔEFD below, order the sides from shortest to longest.
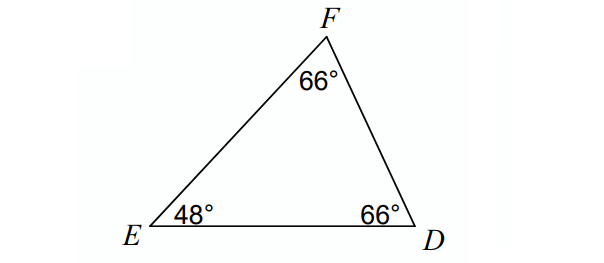
Answer :
m∠E = 48° and the remaining two angles m∠F and m∠D are equal, that is 66°.
side opposite to m∠E ----> FD
side opposite to m∠F ----> ED
side opposite to m∠D ----> EF
In ΔEFD above, the shortest side is FD and the remaining two sides ED and EF are equal in length.
Example 4 :
In ΔJKL below, order the angles from smallest to greatest.
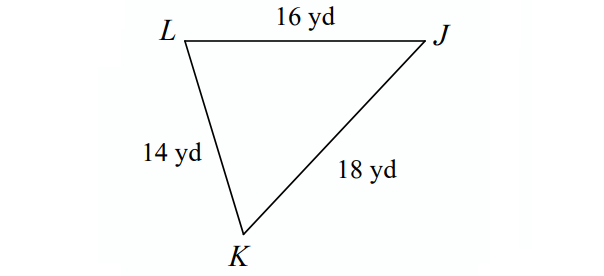
Answer :
Order the sides from shortest to longest :
14 yd, 16 yd, 18 yd
The angle opposite to the shortest side is the smallest angle and the side opposite to the longest side is the largest angle.
angle opposite to the side 14 yd long ----> ∠J
angle opposite to the side 16 yd long ----> ∠K
angle opposite to the side 18 yd long ----> ∠L
Order of the angles from smallest to largest :
∠J, ∠K, ∠L
Example 5 :
In ΔKLM below, KL = 18 cm, LM = 20 cm and KM = 13 cm. Order the angles from largest to smallest.
Answer :
Draw ΔKLM with the given measures.
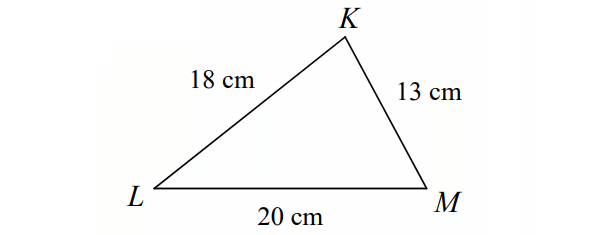
Order the sides from longest to shortest :
20 cm, 18 cm, 13 cm
angle opposite to the side 20 cm long ----> ∠K
angle opposite to the side 18 cm long ----> ∠M
angle opposite to the side 13 cm long ----> ∠L
Order of the angles from smallest to largest :
∠K, ∠M, ∠L
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Quadratic Equation Problems with Solutions (Part - 3)
Apr 21, 25 02:37 AM
Quadratic Equation Problems with Solutions (Part - 3) -
Digital SAT Math Problems and Solutions (Part - 147)
Apr 20, 25 08:38 AM
Digital SAT Math Problems and Solutions (Part - 147) -
Digital SAT Math Problems and Solutions (Part - 146)
Apr 18, 25 06:52 AM
Digital SAT Math Problems and Solutions (Part - 146)