PARALLELOGRAM WORKSHEET

Problem 1 :

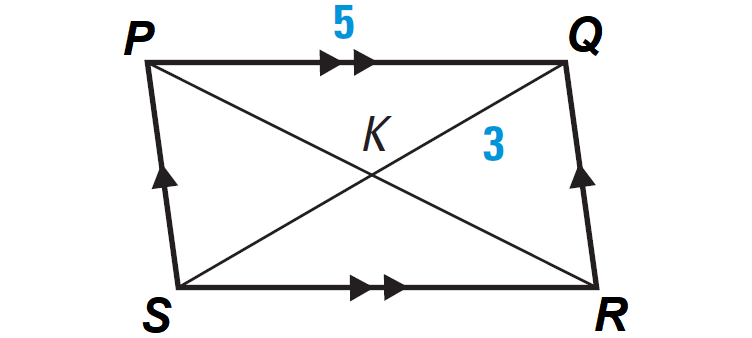
Find the lengths of SR and SK in the parallelogram shown below. Explain your reasoning.

Problem 2 :

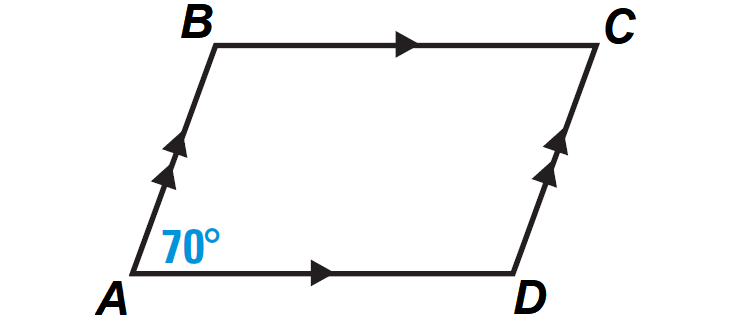
Find the measures of ∠C and ∠B in the parallelogram ABCD. 

Problem 3 :

Find the value of x in the parallelogram ABCD shown below. 

Problem 4 :

In the diagram shown below, ABCD and AEFG are parallelograms. Prove that m∠1  ≅  m∠3.

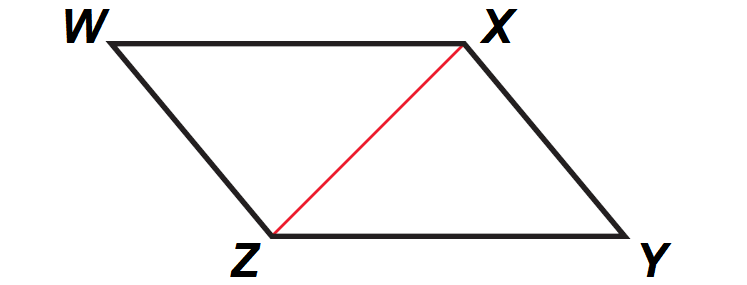
Problem 5 :

In the parallelogram ABCD shown below, prove that WX  ≅  YZ and WZ  ≅  XY. 

Answers

1. Answer :

Finding the length of SR :

By Theorem, opposite sides of a parallelogram are congruent.

So, we have

SR = PQ

From the diagram, PQ = 5.

SR = 5

Finding the length of SK :

By Theorem, diagonals of a parallelogram bisect each other.

So, we have

SK = QK

From the diagram, QK = 3.

SK = 3

2. Answer :

Finding the measure of ∠C :

By Theorem, opposite angles of a parallelogram are congruent.

So, we have

m∠C = m∠A

From the diagram, m∠A = 70°.

m∠C = 70°

Finding the measure of ∠B :

By Theorem, consecutive  angles of a parallelogram are supplementary.

So, we have

m∠A  +  m∠B = 180°

From the diagram, m∠A = 70°.

70° + m∠B = 180°

Subtract 70° from both sides.

 m∠B = 110°

3. Answer :

By Theorem, consecutive  angles of a parallelogram are supplementary.

So, we have

m∠D + m∠C = 180°

Substitute m∠D = 3x° and m∠C = 120°.

3x° + 120° = 180°

Subtract 120° from both sides.

3x° = 60°

3x = 60

Divide both sides by 3.

x = 20

4. Answer :

Plan : Show that both angles are congruent to m∠2. Then use the Transitive Property of Congruence.

5. Answer :

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.