PARTIAL FRACTION WITH CUBIC DENOMINATOR

Example 1 :

Resolve the following rational expressions into partial fractions.

(6x² - x + 1)/(x³ + x² + x + 1)

Solution :

Let us use synthetic division to factorize the cubic denominator.

6x² - x + 1  =  A(x² + 1) + (Bx + C)(x + 1)

6x² - x + 1  =  Ax² + A + Bx² + Bx + Cx + C

Equating the coefficient of x², x and constant terms

6  =  A + B  ----(1)

-1  =  B + C  ----(2)

1  =  A + C   ----(3)

(1) - (2)

A + B - (B + C)  =  6 + 1

A - C  =  7 ----(4)

(3) + (4)  ==>  A + C + A - C  =  1 + 7

2A  =  8

A  =  4

Hence the solution is

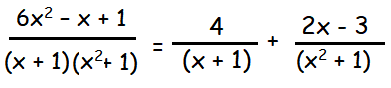
Example 2 :

Resolve the following rational expressions into partial fractions.

(2x² + 5x - 11)/(x² + 2x - 3)

Solution :

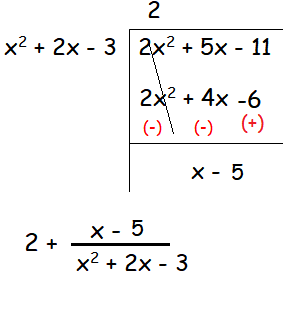
(2x² + 5x - 11)/(x² + 2x - 3) 

  =  2 + [(x - 5)/(x + 3)(x - 1)]

x - 5  =  A/(x + 3) + B(x - 1)

x - 5  =  A(x - 1) + B(x + 3)

Hence the solution is

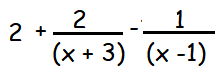
Example 3 :

Resolve the following rational expressions into partial fractions.

(7 + x)/(1 + x)(1 + x²)

Solution :

(7 + x)/(x + 1)(x² + 1)  =  A/(x + 1) + (Bx+C)/(x² + 1)

7 + x  =  A(x² + 1) + (Bx + C)(x + 1)

0  =  A + B  ---(1)

1  =  B + C  ---(2)

7  =  A + C ---(3)

(1) - (2)===>

A - C  =  -1  ---(4)

(3) + (4)  ==>

2A  =  6  ===> A  =  3

B  =  -3

C  =  A + 1  ==> 3 + 1 ==> 4

C  =  4

Hence the solution is

3/(x + 1) + (4 - 3x)/(x² + 1)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.