PERIMETERS AND AREAS OF SIMILAR FIGURES WORKSHEET

Problem 1 :

Pentagons ABCDE and LMNPQ shown below are similar.

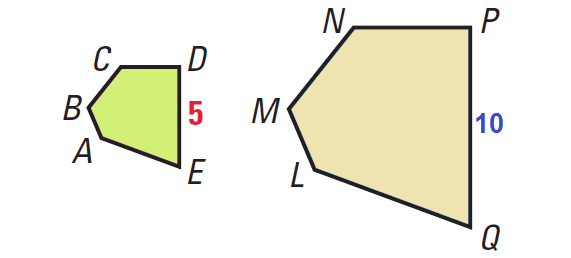
(a)  Find the ratio (red to blue) of the perimeters of the pentagons. 

(a) Find the ratio (red to blue) of the areas of the pentagons. 

Problem 2 :

The red and blue figures shown below are similar. Find the ratio (red to blue) of their perimeters and of their areas.

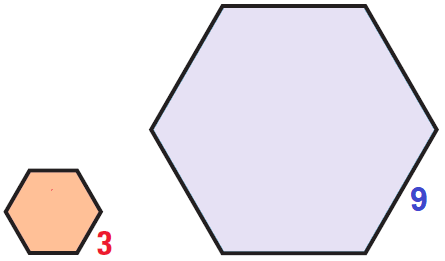
Problem 3 :

The red and blue figures shown below are similar. Find the ratio (red to blue) of their areas using the theorem and justify your answer. 

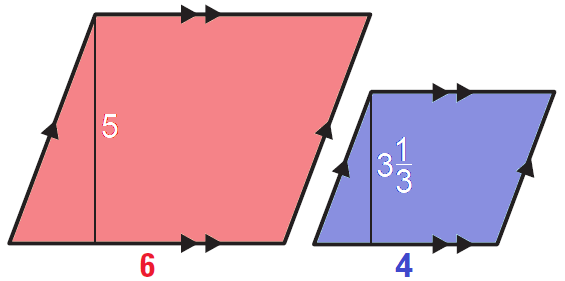
Problem 4 :

A trading pit at the Chicago Board of Trade is in the shape of a series of regular octagons. One octagon has a side length of about 14.25 feet and an area of about 980.4 square feet. Find the area of a smaller octagon that has a perimeter of about 76 feet.

Problem 5 :

Mr. Alex is buying photographic paper to print a photo in different sizes. An 8 inch by 10 inch sheet of the paper costs $0.42. What is a reasonable cost for a 16 inch by 20 inch sheet ?

Answers

1. Answer :

The ratio of the lengths of corresponding sides in the pentagons is 

=  5/10

=  1/2

=  1 : 2

Solution (a) : 

Because the ratio of the lengths of the corresponding sides is 1 : 2, the ratio of the perimeters is also 1 : 2. 

So, the perimeter of pentagon ABCDE is half the perimeter of pentagon LMNPQ. 

Solution (b) : 

The ratio of the lengths of the corresponding sides in the pentagons is 1 : 2. 

Using the Theorem, the ratio of the areas is 

=  1² : 2²

=  1 : 4

So, the area of pentagon ABCDE is one fourth the area of pentagon LMNPQ.

2. Answer : 

Ratio of the perimeters :

The ratio of the lengths of corresponding sides in the hexagon is 

=  3/9

=  1/3

=  1 : 3

Hence, the ratio of the perimeters is also 1 : 3. 

Ratio of the areas :

The ratio of the lengths of the corresponding sides in the pentagons is 1 : 3. 

Using the Theorem, the ratio of the areas is 

=  1² : 3²

=  1 : 9

3. Answer :

The ratio of the lengths of corresponding sides in the parallelograms is 

=  6/4

=  3/2

=  3 : 2

The ratio of the lengths of the corresponding sides in the  parallelograms is 3 : 2. 

Using the Theorem, the ratio of the areas is 

=  3² : 2²

=  9 : 4

Justification : 

Area of red parallelogram is 

=  base ⋅ height

=  6 ⋅ 5

=  30 square units

Area of blue parallelogram is 

=  base ⋅ height

=  4 ⋅ 3⅓

=  4 ⋅ 10/3

=  40/3 square units. 

Ratio of the areas of parallelograms is 

=  30 : 40/3

=  90 : 40

=  9 : 4

Hence, the ratio of the areas found  9 : 4 using Theorem is reasonable. 

4. Answer :

All regular octagons are similar because all corresponding angles are congruent and the corresponding side lengths are proportional.

Draw and label a sketch. 

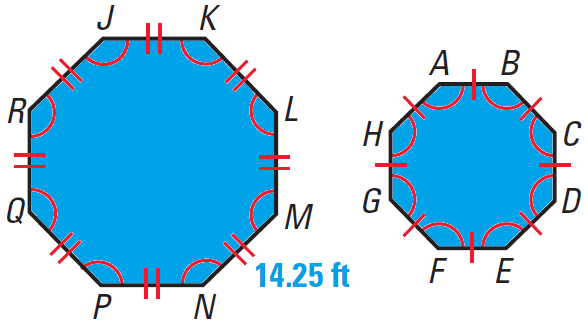
Find the ratio of the side lengths of the two octagons, which is the same as the ratio of their perimeters.

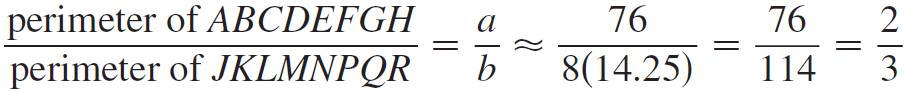
The ratio of the areas of the smaller octagon to the larger is 

=  a² : b²

=  2² : 3²

=  4 : 9

=  4 / 9

Calculate the area of the smaller octagon. Let A represent the area of the smaller octagon. 

Then, we have

A/980.4  =  4/9

Use cross product property. 

9A  =  4(980.4)

Simplify. 

9A  =  3921.6

Divide each side by 9. 

9A/9  =  3921.6/9

A  ≈  435.7

The area of the smaller octagon is about 435.7 square feet.

5. Answer :

The ratio of the lengths of the corresponding sides of the two rectangular pieces of paper is

=  8/16  or  10/20

=  1/2

=  1 : 2

The ratio of the areas of the pieces of paper is

=  1² : 2²

=  1 : 4

So, the area of larger piece of paper is four times the area of smaller piece of paper. 

Because the cost of the paper should be a function of its area, the larger piece of paper should cost about four times as much as the cost of smaller piece of paper.

Hence, the cost of larger piece of paper is 

=  4(0.42)

=  $1.68

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.