PERMUTATION WITH REPETITION EXAMPLE PROBLEMS

Question 1 :

How many ways can the product a²b³c⁴ be expressed without exponents?

Solution :

From the given question, we come to know that "a" is appearing 2 times the letter "b" is appearing 3 times and the letter "c" is appearing 4 times.

Total number of letters  =  2 + 3 + 4  =  9

Total number of ways  =  9!/2!3!4!

  =  9 ⋅ 8 ⋅ 7 ⋅ 6 ⋅ 5 ⋅ 4!/(2 ⋅ 1) (3 ⋅ 2 ⋅ 1)4!

  =  1260

Question 2 :

In how many ways 4 mathematics books, 3 physics books, 2 chemistry books and 1 biology book can be arranged on a shelf so that all books of the same subjects are together.

Solution :

Number of math books  =  4

Number of physics books  =  3

Number of chemistry books  =  2

Number of biology book =  1

Here we have to consider set of each subject books as one units respectively.

So, we have 4 units of books

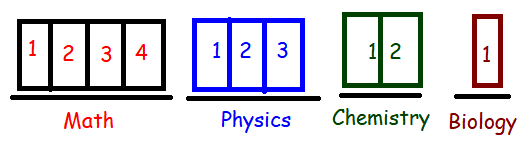
Number of arrangements in ordering math book  =  4!

Number of arrangements in ordering physics book  =  3!

Number of arrangements in ordering chemistry book  =  2!

Number of arrangements in ordering biology book  =  3!

Hence the total number of ways  =   4! ⋅  4! ⋅ 2! ⋅ 3!

  =  6912

Question 3 :

In how many ways can the letters of the word SUCCESS be arranged so that all Ss are together?

Solution :

Let us consider all "S" together as one unit.

"C" is repeating 2 times, both "E" and "U" is repeating once.

Total number of letters  =  5 (SSS as 1)

  =  5!/(2!)

  =  60

Hence the total number of words  =  60.

Question 4 :

A coin is tossed 8 times,

(i) How many different sequences of heads and tails are possible?

(ii) How many different sequences containing six heads and two tails are possible?

Solution :

(i)  Tossing 1 coin as 8 times and 8 coin as 1 time, both are equal.

So, the difference sequence of heads and tails  =  2⁸

(ii)  Total number of entities in each entry  =  8

Head is appearing 6 times, tail is appearing 2 times.

  =  8!/(2! 6!)

  =  (8 ⋅ 7)/2

  =  28

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.