PIECEWISE-DEFINED FUNCTIONS

Learning Objectives :

*  Understanding piecewise-defined functions.

* Defining piecewise function from a absolute value function

*  Graphing piecewise-defined functions.

*  Writing a piecewise-defined rule from a graph. 

Understanding Piecewise Defined Functions

In math, when we have functions, there is a same rule for all values of x. 

For example, in the function f(x)  =  x², we have the same rule x² for all values of x. 

That is, 

x  =  2  -----> 2²  =  4 

x  =  - 3  -----> (- 3)²  =  9 

x  =  0  -----> 0²  =  0 

But in piecewise-defined functions, we have different rule for different values of x. 

Example :

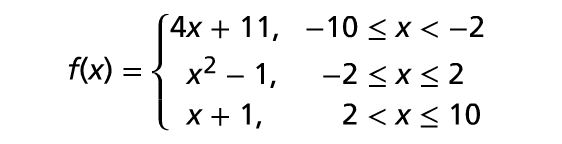
In the above piecewise-defined function, we have different rules for for different values of x. 

When x  =  - 5, the rule is 4x + 11.

Because  -5 lies in the interval [-10, -2) 

When x  =  0, the rule is x² - 1.

Because  -5 lies in the interval [-2, 2] 

When x  =  3, the rule is x + 1.

Because  3 lies in the interval (2, 10] 

Defining Piecewise Function from a Absolute Value Function

Example :

If f(x) = |x - 2|, then redefine f(x) as a piecewise function. 

Solution :

Take the stuff inside the absolute value and equate it to zero. 

x - 2  =  0 

x  =  2

From x  =  2, we can set three conditions as shown below. 

x < 2,  x = 2,  x > 2 

Case (i) :

When x  <  2,

(x - 2)  <  0

So, we have

f(x)  =  -  (x - 2)

f(x)  =  - x + 2

f(x)  =  2 - x

Case (ii) : 

When x  =  0,

(x - 2)  =  0

So, we have

f(x)  =  0

Case (iii) : 

When x  >  2,

(x - 2)  >  0

So, we have

f(x)  =  x - 2

Hence, the given absolute value function is redefined as piecewise function as shown below :   

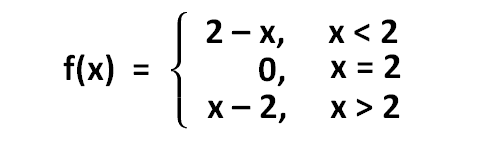
Graphing Piecewise-Defined Functions

Example : 

Graph the piecewise-defined function shown below :

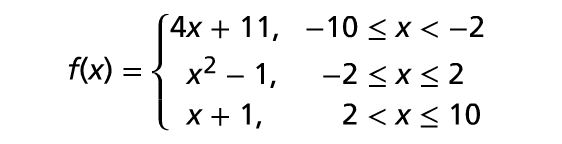
What are the domain and range ? Over what intervals is the function increasing or decreasing ?

Solution :

Step 1 :

Sketch the graph of y  =  4x + 11 for values of x between -10 and -2.

We can consider the following points to sketch the graph of y  =  4x + 11 : 

*  y = 4x + 11 is a linear equation. Then, its graph will be a straight line. 

*  y = 4x + 11 is in slope intercept form y = mx + b.

*  Comparing

y = 4x + 11 and y = mx + b 

we get a positive slope m = 4.

So, the graph of y = 4x + 11 is a rising line. 

Step 2 :

Sketch the graph of y = x² - 1 for values of x between -2 and 2.

We can consider the following points to sketch the graph of y = x² - 1 : 

*  y = x² - 1 is a quadratic equation. Then, its graph will be a parabola.  

*  The sign of x²  in y = x² - 1 is positive. So, the graph will be a open upward parabola. 

*  We can write y = x² - 1 in vertex form as shown below. 

y = (x - 0)² - 1

*  Comparing

y = (x - h)² + k  and  y = (x - 0)² - 1

we get the vertex (h, k)  =  (0, -1)

So, the graph of y = x² - 1 is a open upward parabola with the vertex (0, -1). 

Step 3 :

Sketch the graph of y  =  x + 1 for values of x between 2 and 10.

We can consider the following points to sketch the graph of y  =  x + 1 : 

*  y = x + 1 is a linear equation. Then, its graph will be a straight line. 

*  y = x + 1 is in slope intercept form y = mx + b.

*  Comparing

y = x + 1 and y = mx + b 

we get a positive slope m = 1.

So, the graph of y = x + 1 is a rising line. 

Graph :

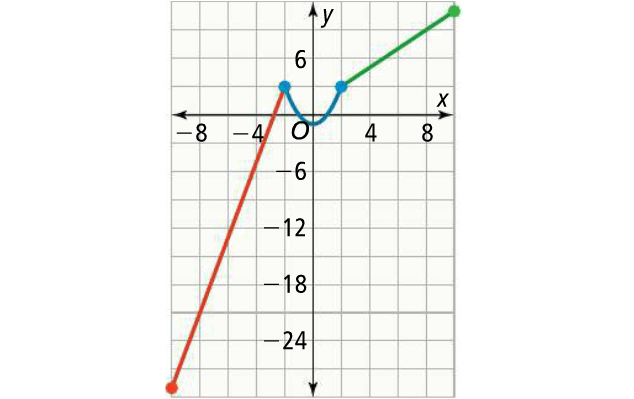
Domain and Range : 

To determine the range, calculate the y-values that correspond to the minimum and maximum x-values on the graph. 

For this graph, these values occur at the endpoints of the domain of the piecewise function,

-10 ≤ x ≤ 10

So, the domain is {x | -10 ≤ x ≤ 10}.

Evaluate y = 4x + 11 for x = -10 :

y  =  4(-10) + 11

y  =  - 40 + 11

y  =  - 29

Evaluate y = x + 1 for x = 10 :

y  =  10 + 1

y  =  11

So, the range is {y | -29 ≤ x ≤ 11}.

Increasing and Decreasing Intervals : 

The function is increasing when

- 10 < x < -2  and 0 < x < 10

The function is decreasing when

- 2 < x < 0

Writing a Piecewise-Defined Rule From a Graph

Example : 

What is the rule that describes the piecewise-defined function shown in the graph ? 

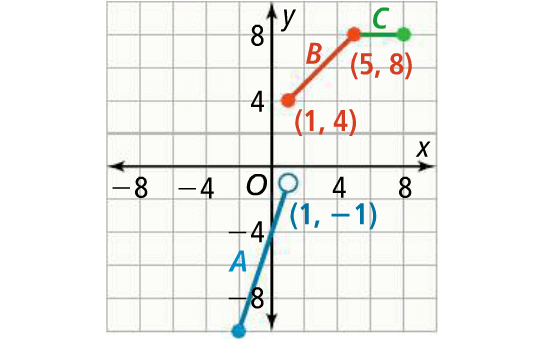
Solution :

Step 1 : 

Notice three separate linear pieces that make up the function. 

Step 2 : 

Determine the domain of each segment.

Step 3 : 

For each segment, use the graph to locate points on the line and to find the slope. 

Step 4 : 

We can use the slope-intercept form of a linear equation

f(x)  =  mx + b

to define the function of each segment. 

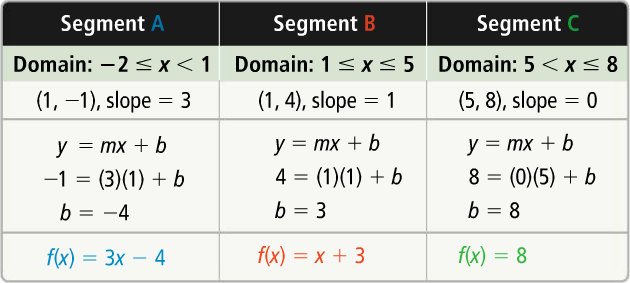
The rule for this function is :

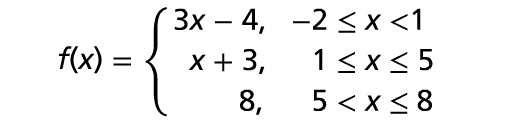
Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.