PRACTICE IN CLASSIFYING POLYNOMIALS

Based on the table given below answer the following questions

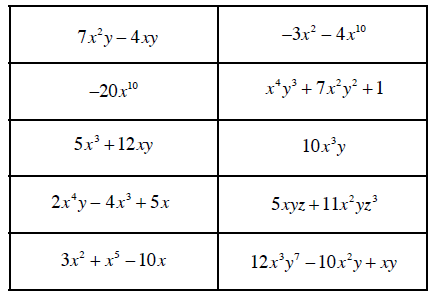
Solution :

By finding degree of the polynomial

Question 1 :

I’m a monomial of degree 4.

Solution :

What is monomial ?

An algebraic term which consists of only one term is known as monomial.

In 10x³y, the degree is 4.

Question 2 :

I am a trinomial of degree 5. My leading coefficient is 2.

Solution :

A polynomial with three terms is trinomial.

Leading coefficients are the numbers written in front of the variable with the largest exponent. 

2x⁴y-4x³+5x

Degree of the term x⁴y is 4+1  ==>  5

Question 3 :

I am a binomial. My leading coefficient is -4.

Solution :

Binomial will consists of 2 terms.

So, the answer is -3x²-4x¹⁰

Question 4 :

My degree is 10.

Solution :

The two polynomials those are having degree 10

-20x¹⁰ and 12x³y⁷-10x²y+xy

The answer is 12x³y⁷-10x²y+xy.

Question 5 :

I am a trinomial. I’m not in standard form.

Solution :

3x²+x⁵-10x

Question 6 :

I am a binomial of degree 3. My leading coefficient is 7.

Solution :

7x²y-4xy

Question 7 :

My leading coefficient is 1.

Solution :

x⁴y³+7x²y²+1

Question 8 :

I’m a monomial. My degree is not 4.

Solution :

We have two monomials -20x¹⁰ and 10x³y.

Degree of the first monomial is 10 and degree of the second monomial is 4.

Since we have to choose monomial which is not having the degree 4, we choose -20x^10.

Question 9 :

I’m in standard form. My leading coefficient is 5.

Solution :

5x³+12xy

Question 10 :

My degree is 6.

Solution :

5xyz + 11x²yz³

Degree of the term 11x²yz³  =  (2+1+3)  ==>  6

Subtract the following polynomials

(Write answers in descending order)

Question 11 :

(-x² + x - 4) - (3x² - 8x - 2)

Solution :

= (-x² + x - 4) - (3x² - 8x - 2)

= -x² + x - 4 - 3x² + 8x + 2

= -x² - 3x² + x + 8x - 4 + 2

= -4x² + 9x - 2

Question 12 :

The Valdez family is filling their swimming pool. The pool will hold 10m² + 2m - 16 gallons of water. If the tank truck has already delivered 7m² - 8m + 12 gallons, how many more gallons of water, in terms of m, must be delivered to fill the pool ? 

Solution :

The capacity of the tank = 10m² + 2m - 16

quantity of water delivered = 7m² - 8m + 12

Difference between them

= 10m² + 2m - 16 - (7m² - 8m + 12)

= 10m² + 2m - 16 - 7m² + 8m - 12

= 10m² - 7m² + 2m + 8m - 16 - 12

= 3m² + 10m - 28

Question 13 :

The perimeter of the triangle is 24x - 6. If two of the sides measure 5x - 7 and 2x + 5, then how long is the third side in terms of x ?

Solution :

Perimeter of the triangle = 24x - 6

The measures of two sides are 5x - 7 and 2x + 5

Let third side be the unknown.

5x - 7 + 2x + 5 + third side = 24x - 6

7x - 2 + third side = 24x - 6

third side = 24x - 6 - (7x - 2)

= 24x - 6 - 7x + 2

= 24x - 7x - 6 + 2

= 17x - 4

So, the length of the third side is 17x - 4.

Question 14 :

Which polynomial has a leading coefficient of 4 and a degree of 3?

a) 3x⁴ - 2x² + 4x - 7        b) 4 - x + 4x² + 5x³

c) 4x⁴ - 3x³ + 2x²        d) 2x + x² + 4x³

Solution :

Option a :

3x⁴ - 2x² + 4x - 7

Leading coefficient = 3

Degree = 4

Option b :

4 - x + 4x² + 5x³

Leading coefficient = 5

Degree = 3

Option c :

4x⁴ - 3x³ + 2x²

Leading coefficient = 4

Degree = 4

Option d :

2x + x² + 4x³

Leading coefficient = 4

Degree = 3

So, option d is correct.

Question 15 :

When 3x² + 7x - 6 + 2x³ is written in standard form, the leading coefficient is

a) 7     b) 2     c) 3     d) -6

Solution :

3x² + 7x - 6 + 2x³

Writing in standard form, we get

2x³ + 3x² + 7x - 6

Leading coefficient is 2.

Question 16 :

What is the degree of the polynomial 2x + x³ + 5x² ?

a) 1      b) 2     c) 3     d) 4

Solution :

2x + x³ + 5x² 

Writing in standard form, we get

x³ + 5x² + 2x

Leading coefficient is 1.

Question 17 :

What is the degree of the polynomial 2x - x² + 4x³ ?

a) 1    b) 2   c) 3    d) 4

Solution :

2x - x² + 4x³ 

Writing in standard form, we get

4x³ - x² + 2x

Leading coefficient is 4.

Question 18 :

Which statement is correct about the polynomial

3 x² + 5x - 2?

a) It is a third-degree polynomial with a constant term of -2.

b) It is a third-degree polynomial with a leading coefficient of 3.

c) It is a second-degree polynomial with a constant term of 2.

d) It is a second-degree polynomial with a leading coefficient of 3

Solution :

3x² + 5x - 2

Degree of the polynomial = 2

leading coefficient = 3

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.