PRACTICE PROBLEMS ON MATRICES FOR GRADE 10

Question 1 :

If –4 is a root of the equation x² + px −4 = 0 and if the equation x² + px +q = 0 has equal roots, find the values of p and q.

Solution :

Let p(x)  =  x² + px −4 

Since -4 is one of the root of the quadratic equation 

p(-4)  =  (-4)² + p(-4) −4 

  0  =  16 - 4p - 4

12 - 4p  =  0

4p  =  12

p  =  3

By applying the value in the first equation, we get 

 x² + px −4 = 0

 x² + 3x −4  =  0

(x - 1)(x + 4)  =  0

x = 1 and x = -4.

By applying the value of p in the second equation, we get

x² + 3x + q = 0

Since the above quadratic equation will have equal roots,

b² - 4ac  =  0

3² - 4(1)q  =  0

-4q  =  -9

q  =  9/4

Question 2 :

Two farmers Senthil and Ravi cultivates three varieties of grains namely rice, wheat and ragi. If the sale (in ₹) of three varieties of grains by both the farmers in the month of April is given by the matrix.

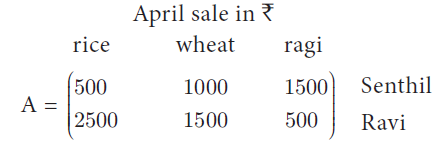
and the May month sale (in ₹) is exactly twice as that of the April month sale for each variety.

(i) What is the average sales of the months April and May.

(ii) If the sales continues to increase in the same way in the successive months, what will be sales in the month of August?

Solution :

In order to find the sales on May month, we multiply the April month sales by 2.

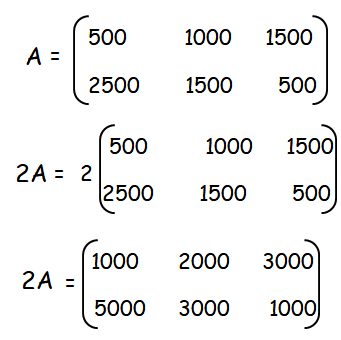
(i) What is the average sales of the months April and May

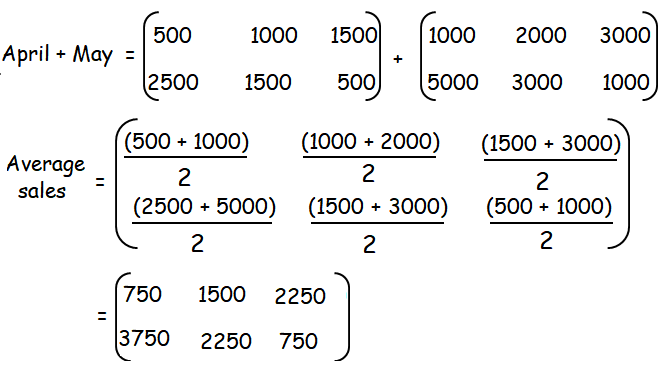
(ii)

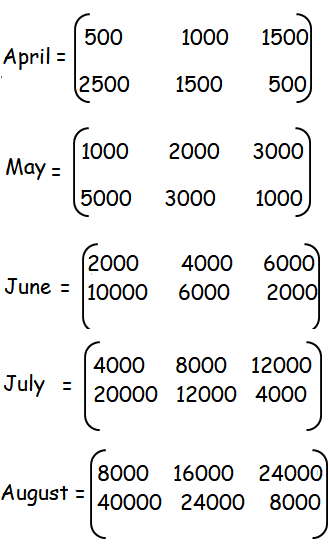
Question 3 :

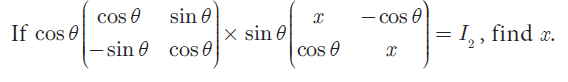
Question 4 :

Given 

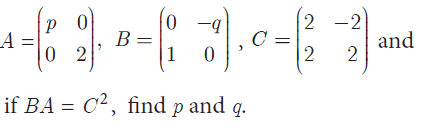
Solution :

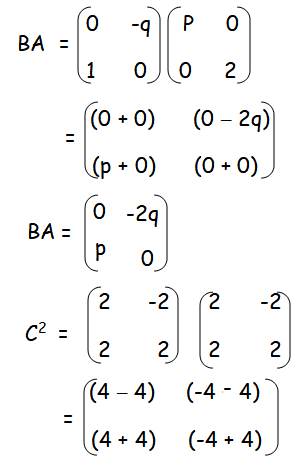
By equating the corresponding terms, we get 

-2q  =  -8

q  =  4

p  =  8

Hence the values of p and q are 8 and 4 respectively.

Question 5 :

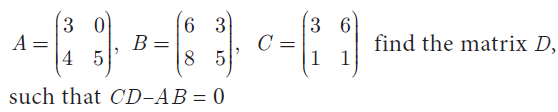
Solution :

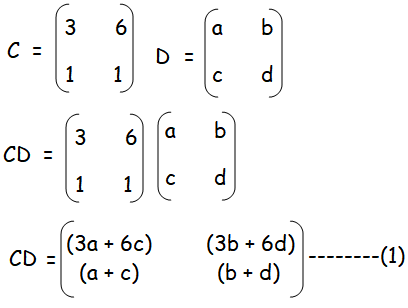
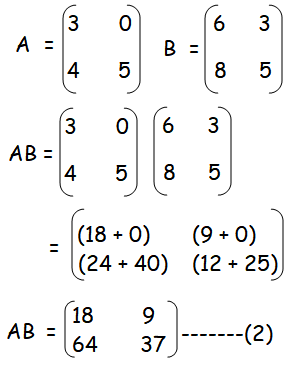
Equating the corresponding terms, we get 

CD - AB  =  0

3a + 6c - 18  =  0

3a + 6c  =  18

a + 2c  = 6 ------(3)  

a + c - 64  =  0

a + c  =  64------(4)  

(3) - (4)

c  =  6 - 64

c  =  -58

By applying the value of c in (3), we get 

a + 2(-58)  =  6

a - 116  =  6

a  =  6 + 116 

a  =  122

3b + 6d  =  9 

b + 2d  =  3 -----(5)

b + d  =  37 -----(6)

(5) - (6)

2d - d  =  3 - 37

d  =  -34

By applying the value of d in (5), we get

b + 2(-34)  =  3

b - 68  =  3

b  =  3 + 68

b  =  71

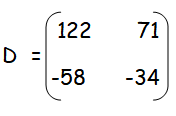
Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.