PRACTICE WORKSHEET RELATIONS AND FUNCTIONS WITH ANSWERS
Question 1 :
If the ordered pairs (x2 − 3x, y2 + 4y) and (-2,5) are equal, then find x and y.
Question 2 :
The Cartesian product A×A has 9 elements among which (–1, 0) and (0,1) are found. Find the set A and the remaining elements of A×A.
Question 3 :
Given that f(x) =
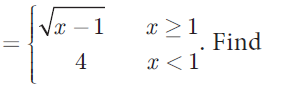
Question 4 :
Let A= {9, 10, 11, 12, 13, 14, 15, 16, 17} and let f : A-> N be defined by f (n) = the highest prime factor of n ∈ A. Write f as a set of ordered pairs and find the range of f.
Question 5 :
Two sets have m and k elements. If the total number of subsets of the first set is 112 more than that of the second set, find the values of m and k.
Question 6 :
If n(P(A)) = 1024, n(AUB) = 15 and n(P(B)) = 32, then find n(AnB)

Detailed Answer Key
Question 1 :
If the ordered pairs (x2 − 3x, y2 + 4y) and (-2,5) are equal, then find x and y.
Answer :
|
x2 - 3x = -2 x2 - 3x + 2 = 0 (x - 1)(x - 2) = 0 x = 1 and x = 2 |
y2 + 4y = 5 y2 + 4y - 5 = 0 (y + 5)(y - 1) = 0 y = -5 and y = 1 |
Question 2 :
The Cartesian product A×A has 9 elements among which (–1, 0) and (0,1) are found. Find the set A and the remaining elements of A×A.
Answer :
So, in this question since A X A has 9 elements, so the number of elements in the set A must obviously be 3.
Since the given elements of the cross product have -1, 0 and 1 as part of the entries, clearly these only must be the elements of the set A.
So A = {-1, 0, 1}
A X A
= {(-1, -1),(-1, 0),(-1, 1),(0, -1),(0, 0),(0, 1),(1, -1),(1, 0),(1, 1)}
Question 3 :
Given that f(x) =
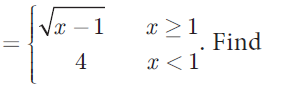
(i) f (0) (ii) f (3) (iii) f (a+1) in terms of a.(Given that a ≥ 0)
Answer :
(i) f(0)
Instead of x, we have 0, it is less than 1
f(0) = 4
(ii) f(3)
Instead of x, we have 3, it is greater than 1
f(3) = √(3 - 1)
f(3) = √2
(iii) f (a+1)
Instead of x, we have a+1, it is greater than 1
f(a + 1) = √(a + 1 - 1)
f(a + 1) = √a
Question 4 :
Let A= {9, 10, 11, 12, 13, 14, 15, 16, 17} and let f : A-> N be defined by f (n) = the highest prime factor of n ∈ A. Write f as a set of ordered pairs and find the range of f.
Answer :
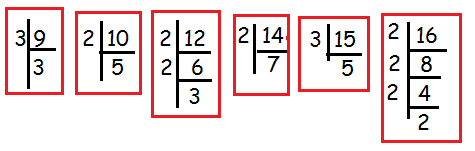
|
Elements of A 9 10 11 12 13 14 15 16 17 |
Highest prime factor 3 5 11 3 13 7 5 2 17 |
Set ordered pairs :
= {(9,3) (10, 5)(11, 11)(12, 3)(13, 13) (14, 7) (15, 5) (16,2) (17, 17)}
Range = {2, 3, 5, 7, 11, 13, 17}
Question 5 :
Two sets have m and k elements. If the total number of subsets of the first set is 112 more than that of the second set, find the values of m and k.
Solution :
If a set contains n number of elements, then using the formula 2n, we find the number of subsets.
Number of subsets of a set containing m elements = 2m
Number of subsets of a set containing k elements = 2k
To find the more number of subsets in second set which is containing k elements, we have to find the difference between number of subsets in these two sets.
2m - 2k = 112
Factoring 2k, we get
2k (2m - k - 1) = 112
Writing 112 in exponential form, we get
2k (2m - k - 1) = 24 x 7
2k = 24
So, one of the possible value of k is 4. Then 2m - k - 1 = 7
2m - k - 1 = 7
2m - k = 8
2m - k = 23
m - k = 3
m - 4 = 3
Then m must be 7.
Question 6 :
If n(P(A)) = 1024, n(AUB) = 15 and n(P(B)) = 32, then find n(AnB)
Solution :
n(P(A)) = 1024 = 210
Number of subsets = 2m
Where m is the number of elements in the set A. So, number of elements in the set A is 10.
n(P(B)) = 32= 25
Number of elements in set B is 5.
n(A) = 10, n(B) = 5 given that n(AUB) = 15
n(AUB) = n(A) + n(B) - n(AnB)
15 = 10 + 5 - n(AnB)
15 = 15 - n(AnB)
n(AnB) = 0
So, A and B are mutually exclusive sets.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
AP Calculus AB Problems with Solutions (Part - 8)
Jan 30, 25 09:48 AM
AP Calculus AB Problems with Solutions (Part - 8) -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Jan 29, 25 06:00 AM
SAT Math Resources (Videos, Concepts, Worksheets and More) -
Digital SAT Math Problems and Solutions (Part - 105)
Jan 29, 25 05:52 AM
Digital SAT Math Problems and Solutions (Part - 105)
