PRE CALCULUS PROBLEMS AND SOLUTIONS
(Part - 2)

Problem 1 :

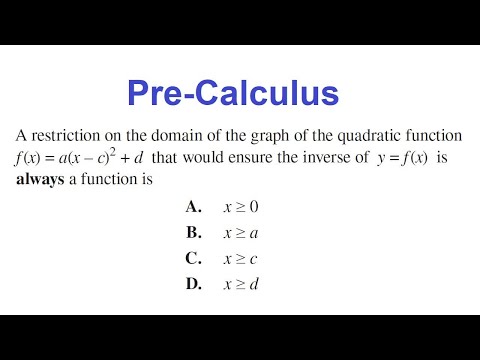
A restriction on the domain of the graph of the quadratic function

f(x) = a(x – c)² + d

that would ensure the inverse of y = f(x) is always a function is

A)  x ≥ 0

B)  x ≥ a

C)  x ≥ c

D)  x ≥ d

Solution :

Problem 2 :

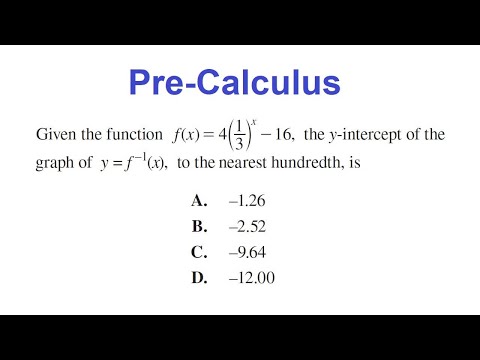
In the function given above, the y-intercept of the graph of f^-1(x), to the nearest hundredth is

A)  -1.26

B)  -2.52

C)  -9.64

D)  -12.00

Solution :

Problem 3 :

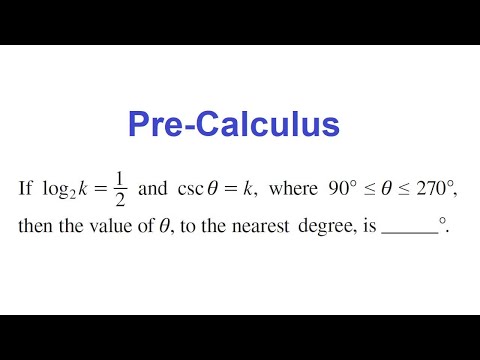
If log₂ k = ½ and csc θ = k, where 90° ≤ θ ≤ 270°, then  the value of θ to the nearest degree is _____.

Solution :

Problem 4 :

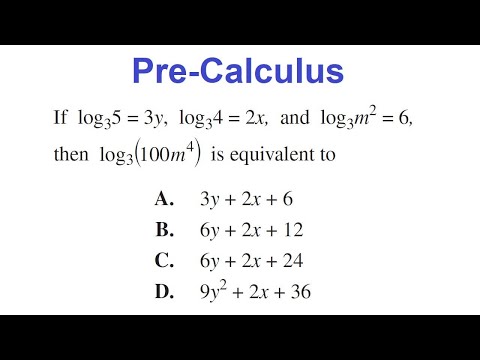
If log₃ 5 = 3y, log₃ 4 = 2x and log₃ m² = 6, then log₃ (100m⁴) is equivalent to

A)  3y + 2x + 6

B)  6y + 2x + 12

C)  6y + 2x + 24

D)  9y² + 2x + 36

Solution :

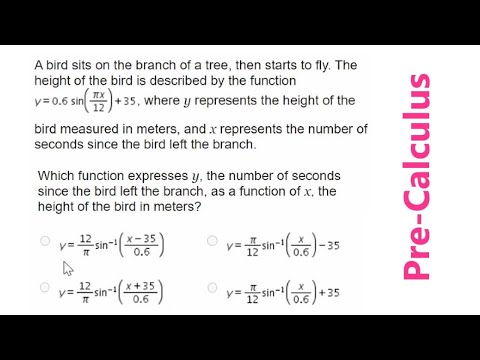
Problem 5 :

A bird sits on the branch of a tree, then starts to fly. The height of the bird is described by the function

where y represents the height of the bird measured in meters, and x represents the number of seconds since the bird left the branch.

Which function expresses y, the number of seconds since the bird left the branch, as a function of x, the height of the bird in meters ?

Solution :

Problem 6 :

If x² < 25, which of the following must be true ?

A)  0 < x < 5

B)  -5 < x < 0

C)  -5 < x < 5

D)  x < 5

Solution :

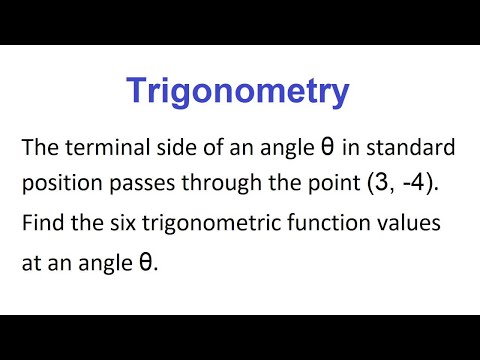
Problem 7 :

The terminal side of an angle θ in standard position passes through the point (3, -4). Find the six trigonometric function values at an angle θ.

Solution :

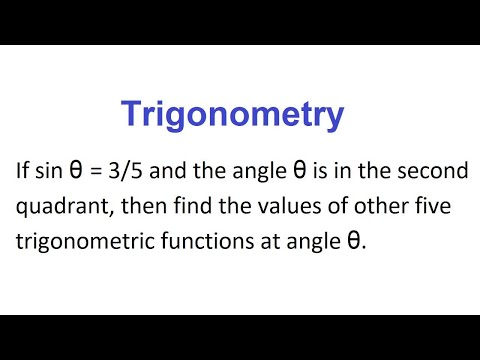
Problem 8 :

If sin θ = ⅗ and the angle θ is in the second quadrant, then find the values of other five trigonometric functions at angle θ.

Solution :

You might like these

Pre-Calculus Problems and Solutions (Part - 1)

Pre-Calculus Problems and Solutions (Part - 2)

Pre-Calculus Problems and Solutions (Part - 3)

Pre-Calculus Problems and Solutions (Part - 4)

Pre-Calculus Problems and Solutions (Part - 5)

Pre-Calculus Problems and Solutions (Part - 6)

Pre-Calculus Problems and Solutions (Part - 7)

Pre-Calculus Problems and Solutions (Part - 8)

Pre-Calculus Problems and Solutions (Part - 9)

Pre-Calculus Problems and Solutions (Part - 10)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.