PRECALCULUS PROBLEMS AND SOLUTIONS
(Part - 12)

Problem 1 :

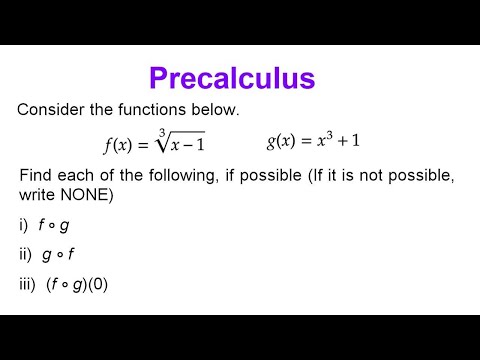
Consider the functions below.

Find each of the following, if possible (If it is not possible, write NONE)

i)  f ∘ g

ii)  g ∘ f

iii)  (f ∘ g)(0)

Solution :

Problem 2 :

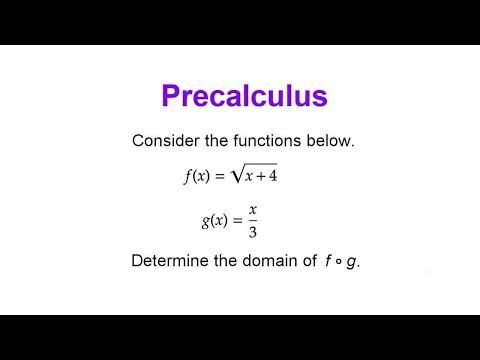
Consider the functions below.

Determine the domain of f ∘ g.

Solution :

Problem 3 :

A roofing contractor purchases a shingle delivery truck with a shingle elevator for $39,000. The vehicle requires an average expenditure of $5.50 per hour for fuel and maintenance, and the operator is paid $11.50 per hour.

(a)  Write a linear equation giving the total cost C of operating this equipment for t hours. (Include the purchase cost of the equipment.)

C(t) = ?

(b) Assuming that customers are charged $30 per hour for machine use, write an equation for the revenue R derived from t hours of use.   

R(t) = ?

(c) Use the formula for profit P = R – C to write an equation for the profit derived from t hours of use.   

P(t) = ?

(d) Use the result of part (c) to find the break-even point - that is, the number of hours this equipment must be used to yield a profit of 0 dollars.

t = ?

Solution :

Problem 4 :

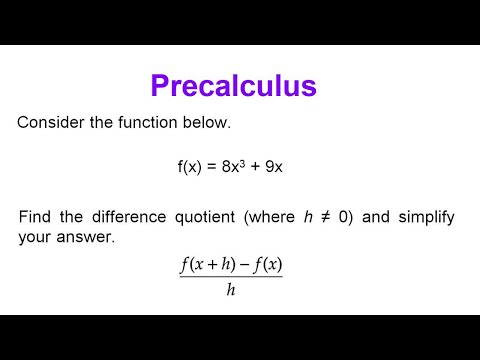
Consider the function below.

f(x) = 8x³ + 9x

Find the difference quotient (where h ≠ 0) and simplify your answer.

Solution :

Problem 5 :

Consider the function below.

Find the difference quotient (where x ≠ 11) and simplify your answer.

Solution :

You might like these

Precalculus Problems and Solutions (Part - 1)

Precalculus Problems and Solutions (Part - 2)

Precalculus Problems and Solutions (Part - 3)

Precalculus Problems and Solutions (Part - 4)

Precalculus Problems and Solutions (Part - 5)

Precalculus Problems and Solutions (Part - 6)

Precalculus Problems and Solutions (Part - 7)

Precalculus Problems and Solutions (Part - 8)

Precalculus Problems and Solutions (Part - 9)

Precalculus Problems and Solutions (Part - 10)

Precalculus Problems and Solutions (Part - 11)

Precalculus Problems and Solutions (Part - 12)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.