PROBLEMS INVOLVING ANGLE OF ELEVATION AND DEPRESSION

Problem 1 :

From the top of a tree of height 13 m the angle of elevation and depression of the top and bottom of another tree are 45° and 30° respectively. Find the height of the second tree. (√3 = 1.732)

Solution :

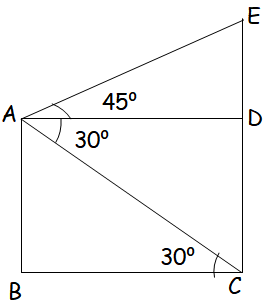
AB = 13 m  =  DC

In triangle AED,

tan 45  =  ED/AD

1  =  ED/AD

AD =  ED  --(1)

In triangle ABC,

tan 30  =  AB/BC

1/√3  =  13/BC

BC  =  13√3  ----(2)

AD  =  BC

By applying the value of AD in (1), we get 

ED  =  13√3 

height of second tree  =  ED + DC

  =  13√3 + 13

  =  13(√3 + 1)

  =  13(1.732 + 1)

  =  13(2.732)

  =  35.516 m

Hence the height of the second tree is 35.52 m

Problem 2 :

A man is standing on the deck of a ship, which is 40 m above water level. He observes the angle of elevation of the top of a hill as 60° and the angle of depression of the base of the hill as 30° . Calculate the distance of the hill from the ship and the height of the hill. (√3 = 1.732)

Solution :

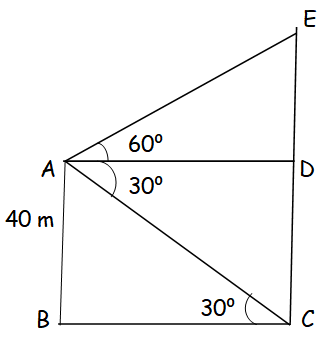
In triangle AED,

tan 60  =  ED/AD

√3  =  ED/AD 

AD  =  ED/√3 -----(1)

In triangle ABC,

tan 30  =  AB/BC

1/√3  =  40/BC

BC  =  40√3  -----(2)

BC  =  AD

(1)  =  (2)

40√3  =  ED/√3

ED  =  40√3(√3) 

  =  40(3)

ED  =  120 m

Height of hill  =  ED + DC 

  =  120 + 40

  =  160 m

Distance from hill to ship  =  40√3

=  40(1.732)

=  69.28 m

Problem 3 :

If the angle of elevation of a cloud from a point ‘h’ meters above a lake is θ₁ and the angle of depression of its reflection in the lake is θ₂ . Prove that the height that the cloud is located from the ground is h(tan θ₁+ tan θ₂)/(tan θ₂ - tan θ₁)

Solution :

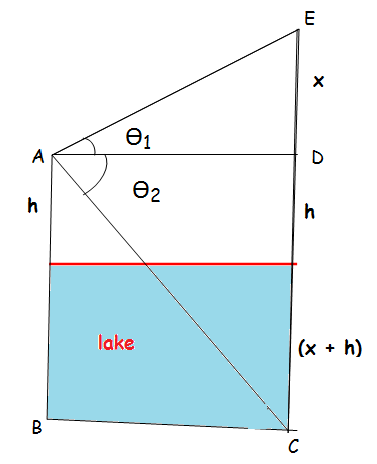
In triangle AED,

tan θ₁  =  ED/AD

tan θ₁  =  x/AD

AD  =  x/tan θ₁

AD  =  x cot θ₁  -----(1)

In triangle ADC,

tan θ₂  =  DC/AD

tan θ₂  =  (2h + x)/AD

AD  =  (2h + x)/tan θ₂

AD  =  (2h + x)cot θ₂ -----(2)

(1) = (2)

x cot θ_{1  =}  (2h + x)cot θ₂ 

x cot θ₁  =  2h cot θ₂ + x cot θ₂

x (cot θ₁ - cot θ₂) =  2h cot θ₂

x (tan θ₂ - tan θ₁)/(tan θ₁ tan θ₂) =  2h (1/tan θ₂)

2h  =  [xtan θ₂(tan θ₂ - tan θ₁)]/(tan θ₁ tan θ₂)

2h  =  x(tan θ₂ - tan θ₁)/tan θ₁

x  =  2h tan θ₁/(tan θ₂ - tan θ₁)

height of cloud  =  x + h

  =  [2h tan θ₁/(tan θ₂ - tan θ₁)] + h

  =  [2h tan θ₁+ h(tan θ₂ - tan θ₁)]/(tan θ₂ - tan θ₁)] 

  =  h[tan θ₁+ tan θ₂]/(tan θ₂ - tan θ₁)]

Hence proved.

Apart from the stuff given above, if you need any other stuff in Math, please use our google custom search here.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.