PROBLEMS ON EXTERIOR ANGLES OF A TRIANGLE

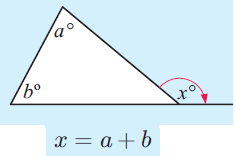
An exterior angle of a triangle is equal in size to the sum of its interior opposite angles.

Problem 1 :

In the diagram shown below, find the value of y.

Solution :

In the triangle above, using Exterior Angle Theorem,

y° + 80° = 125°

y + 80 = 125

Subtract 80 from both sides.

y = 45

Problem 2 :

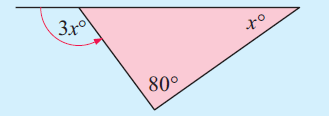
In the diagram shown below, find the value of x.

Solution :

In the triangle above, using Exterior Angle Theorem,

x° + 80° = 3x°

x + 80 = 3x

Subtract x from both sides.

80 = 2x

Divide both sides by 2.

40 = x

Problem 3 :

In the diagram shown below, find the values of a and b.

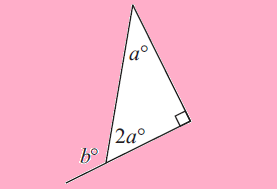
Solution :

In triangle, the sum of interior angles is 180 degrees.

a° + 2a° + 90° = 180°

3a + 90 = 180

Subtract 90 from both sides.

3a = 90

Divide both sides by 3.

a = 30

To find the value of b, we can use any one of the following two ways given below.

In both the ways, the answer will be same.

Problem 4 :

In the diagram shown below, find the value of p.

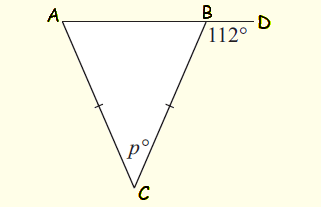
Solution :

The triangle ABC above is an isosceles triangle.

∠CAB = ∠CBA

∠CBA + ∠CBD = 180°

∠CBA + 112° = 180°

Subtract 112° from both sides.

∠CBA = 68°

In triangle ABC,

∠ACB + ∠CAB + ∠CBA = 180°

p° + 68° + 68° = 180°

p + 136 = 180

Subtract 136 from both side

p = 44

Problem 5 :

In the diagram shown below, find the values of x and y.

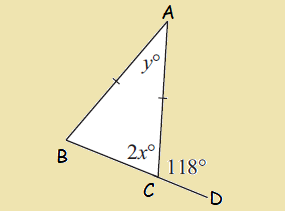
Solution :

2x° and 118° are linear pair.

2x° + 118° = 180°

Subtract 118 from both sides.

2x = 62

Divide both sides by 2.

x = 31

The given triangle ABC is a isosceles triangle.

∠ABC = ∠ACB = 2x

In triangle ABC,

∠ABC + ∠ACB + ∠CAB = 180°

2x + 2x + y = 180

4x + y = 180

Substitute x = 31.

4(31) + y = 180

124 + y = 180

Subtract 124 from both sides.

y = 56

y = 56

Problem 6 :

Find ∠LMN and ∠MLN.

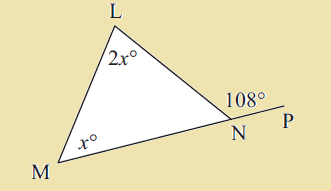
Solution :

In the triangle above, using Exterior Angle Theorem,

∠LMN + ∠MLN = ∠LNP

x + 2x = 108

3x = 108

Divide both sides by 3.

x = 36

∠LMN = 36°

∠MLN = 2(36°)

∠MLN = 72°

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.