PROBLEMS ON STANDARD INTEGRALS

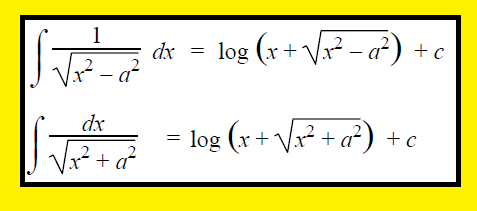
Problem 1 :

Evaluate 

∫1/(1+9x²) dx

Solution :

  =  ∫1/(1+3²x²)  dx

The given exactly matches with the formula 

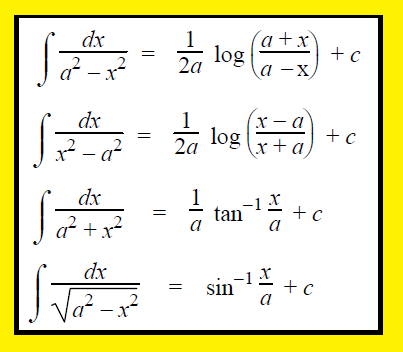
∫1/(a²+x²) dx  =  1/a tan^-1(x/a) + c

=  1/(1+(3x)²)  dx

=  1/3 tan^-1(3x/1) + C

=  1/3 tan^-1(3x) + C

Problem 2 :

Evaluate 

∫1/(1-9x²) dx

Solution :

  =  ∫1/(1-3²x²)  dx

The given exactly matches with the formula 

∫1/(a²-x²) dx  =  (1/2a) [log (a+x)/(a-x)] + c

a  =  1 and x  =  3x

=  (1/2) [log(1+3x)/(1-3x)] + C

Problem 3 :

Evaluate 

∫1/(1+x²/16) dx

Solution :

  =  ∫1/((1+(x/4)²) dx

The given exactly matches with the formula 

∫1/(a²+x²) dx  =  (1/a) tan^-1 (x/a) + c

a  =  1 and x  =  x/4

=  1 tan^-1 ((x/4)/1) + C

=  tan^-1 (x/4) + C

Problem 4 :

Evaluate 

∫1/((x+2)²-4) dx

Solution :

  =  ∫1/((x+2)²-2²) dx

The given exactly matches with the formula 

∫1/(x²-a²) dx  =  (1/2a) [log (x-a)/(x+a)] + c

x  =  x+2 and a  =  2

=  (1/2⋅2) [log(x+2-2)/(x+2+2)] + C

=  (1/4) [log(x/(x+4))] + C

Problem 5 :

Evaluate 

∫1/√(25-x²) dx

Solution :

  =  ∫1/√(5²-x²) dx

The given exactly matches with the formula 

∫1/√(a²-x²) dx  =  sin^-1(x/a) + c

a  =  5 and x  =  x

=  sin^-1(x/5) + c

Problem 6 :

Evaluate 

1/√(4x²-25) dx

Solution :

  =  ∫ 1/√((2x)²-5²) dx

The given exactly matches with the formula 

∫1/√(x²-a²) dx  =  log[x+√(x²-a²)]+C

a  =  5 and x  =  2x

=  (1/2) log[2x+√(4x²-25)] + C

Problem 7 :

Evaluate 

∫1/√(9x²+16) dx

Solution :

  =  ∫1/√((3x)²+4²) dx

The given exactly matches with the formula 

∫1/√(a²+x²) dx  =  log[x+√(a²+x²)] + c

x  =  3x and a  =  4

=  log[3x+√(9x²+4²) + C

=  log[3x+√(9x²+16) + C

Problem 8 :

Evaluate 

∫1/((3x+5)²+4) dx

Solution :

=  ∫1/((3x+5)²+4) dx

The given exactly matches with the formula 

∫1/(x²+a²) dx  =  (1/a) tan^-1(x/a) + c

x  =  3x+5 and a  =  2

=  (1/2) [tan^-1(3x+5)/2] + C

Problem 9 :

Evaluate 

∫1/((x-2)² + 1) dx

Solution :

  = ∫1/((x-2)² + 1) dx

The given exactly matches with the formula 

∫1/(x²+a²) dx = (1/a) tan^-1(x/a) + C

∫1/((x-2)² + 1) dx = (1/1) tan^-1[(x - 2) / 1] + C

= tan^-1(x - 2) + C

Problem 10 :

Evaluate 

∫x²/(x² + 5) dx

Solution :

  = ∫x²/(x² + 5) dx

Adding and subtracting 5 in the numerator, we get

  = ∫(x² + 5 - 5)/(x² + 5) dx

Decomposing into two fractions, we get

  = ∫(x² + 5)/(x² + 5) dx - ∫(5/(x² + 5)) dx

  = ∫dx - 5∫(1/(x² + 5)) dx

= x - 5 ∫(1/(x² + √5² )) dx

= x - 5 [(1/√5) tan^-1(x/√5)] + C

= x -  [√5 tan^-1(x/√5)] + C

Problem 11 :

Evaluate 

∫1/√(1 + 4x²) dx

Solution :

  = ∫1/√(1 + 4x²) dx

= ∫1/√(1 + (2x)²) dx

∫1/√(1 + x²) = log(x + √(1 + x²) + C 

= log(2x + √(1 + 4x²)/2 + C 

= (1/2) log (2x + √(1 + 4x²) + C 

Problem 12 :

Evaluate 

∫1/√(4x² - 25) dx

Solution :

  = ∫1/√(4x² - 25) dx

= ∫1/√((2x)² - 5²) dx

∫1/√(1 - x²) = log(x + √(1 - x²) + C 

= log(2x + √(4x²- 25))/2 + C 

= (1/2) log(2x + √(4x²- 25)) + C

Problem 12 :

Evaluate 

∫1/√(4x² - 25) dx

Solution :

  = ∫1/√(4x² - 25) dx

= ∫1/√((2x)² - 5²) dx

∫1/√(1 - x²) = log(x + √(1 - x²) + C 

= log(2x + √(4x²- 25))/2 + C 

= (1/2) log(2x + √(4x²- 25)) + C

Evaluate 

∫1/√(4x² - 25) dx

Solution :

  = ∫1/√(4x² - 25) dx

= ∫1/√((2x)² - 5²) dx

∫1/√(1 - x²) = log(x + √(1 - x²) + C 

= log(2x + √(4x²- 25))/2 + C 

= (1/2) log(2x + √(4x²- 25)) + C

Problem 13 :

Evaluate 

∫1/(x² - 2x + 5) dx

Solution :

  = ∫1/(x² - 2x + 5) dx

Since we have quadratic at the denominator, we have to rewrite it as sum of difference of square using the method of completing the square.

x² - 2x + 5 = x² - 2⋅x⋅1 + 1² - 1² + 5

= (x - 1)² - 1 + 5

= (x - 1)² + 4

∫1/(x² - 2x + 5) dx = ∫1 / [(x - 1)² + 4] dx

∫1 / (x² + a²) dx = (1/a) tan^-1 (x/a) + C

= (1/2) tan^-1 [(x-1)/2] + C

Problem 14 :

Evaluate 

∫1/√(x² + 12x + 11) dx

Solution :

  = ∫1/√(x² + 12x + 11) dx

Since we have quadratic at the denominator, we have to rewrite it as sum of difference of square using the method of completing the square.

x² + 12x + 11 = x² + 2⋅x⋅6 + 6² - 6² + 11

= (x + 6)² - 36 + 11

= (x + 6)² - 25

∫1/√[(x + 6)² - 25] dx = ∫1/√[(x + 6)² - 5²] dx

∫1 / √(x² - a²) dx = log (x + √(x² - a²)) + C

= log ( (x + 6) + √[(x + 6)² - 5²] ) + C

Problem 15 :

Evaluate 

∫1/√(12 + 4x - x²) dx

Solution :

  = ∫1/√(12 + 4x - x²) dx

The quadratic that we have at the denominator is not in the standard form.

12 + 4x - x² = -[x² - 4x - 12]

= -[x² - 2⋅x⋅2 + 2² - 2² - 12]

= -[(x - 2)² - 4 - 12]

= -[(x - 2)² - 16]

= 16 - (x - 2)²

= ∫1/√(12 + 4x - x²) dx = ∫1/√[16 - (x - 2)²] dx

= ∫1/√[4² - (x - 2)²] dx

∫1 / √(a² - x²) dx = sin^-1 (x/a) + C

∫1/√[4² - (x - 2)²] dx = sin^-1 [(x-2)/4] + C

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.