PROBLEMS ON SURFACE AREA OF CYLINDER

Curved surface area of cylinder is the measurement of outer area,  where the extension of top and bottom portion wont be included. 

Curved surface area of cylinder

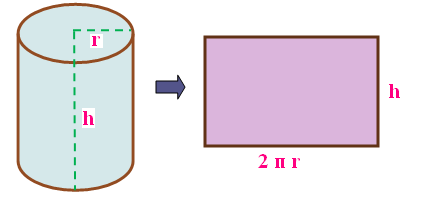
If a rectangle revolves about one side and completes one full rotation, the solid thus formed is called a right circular cylinder. The above picture shows that how rectangle forms a right circular cylinder. In other words curved surface area is simply said as CSA 

CSA of cylinder = 2 π r h

"r" and "h" stands for radius and height of cylinder.

Curved surface area of hollow cylinder

A hollow cylinder is a three dimensional solid bounded by two parallel cylindrical surfaces and by two parallel circular bases cut out from two parallel planes by these two cylindrical surfaces.

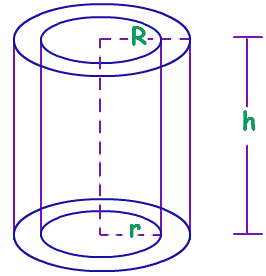
CSA of hollow cylinder = 2πh(R+r)

R  =  external radius, r = internal radius and h  =  height

Example 1 :

The external surface area of a hollow cylinder is 540Π cm².Its internal diameter is 16 cm and height is 15 cm. Find the curved surface area.

Solution :

External surface area of cylinder  =  540 Π cm²

2 Π R h  =  540 Π cm² 

Internal radius (r)  =  16/2  =  8 cm

height (h)  =  15 cm

  2⋅ Π ⋅ R ⋅ h  =  540 Π 

2 Π R⋅15  =  540 Π 

=  540 Π ⋅ (1/2) ⋅ (1/Π) ⋅ (1/15)

=  270/(15)

 R  =  18

Curved surface area =  2 Π h (R + r)

=  2Π(15)(18+8)

=  390Π cm²

Curved surface area of the cylinder  =  390Π cm²

Example 2 :

The external diameter of the cylindrical shaped iron pipe is 25 cm and its length is 20 cm. If the thickness of the pipe is 1 cm, find the curved surface area of the pipe.

Solution :

External radius of the pipe (R)  =  12.5 cm 

height of the pipe (h)  =  20 cm

thickness of the pipe (w)  =  1 cm

To find curved surface area of the cylinder, we have to find the internal radius (r)

W = R - r

1  =  12.5 - r

r  =  12.5 - 1

r  =  11 .5 cm

Curved surface area  =  2 Π h (R + r)

=  2 Π (20) (12.5 + 11.5)

=  960 Π cm²

Hence curved surface area of cylinder is 960 Π cm².

Example 3 :

The radii of two right circular cylinders are in the ratio 3:2 and their heights are in the ratio 5:3. Find the ratio of their curved surface areas.

Solution :

Let r₁ , r₂ and h₁, h₂ are radii and heights of first and second cylinders respectively.

Now we have to find the ratio of  their curved surface areas

Curved surface area of cylinder  =  2Πrh

Curved surface area of first cylinder  =  2Πr₁h₁

Curved surface area of second cylinder  =   2Πr₂h₂

r₁ : r₂  =  3:2

r₁/r₂  =  3/2

r₁  =  3r₂/2

h₁ : h₂ = 5 : 3

h₁/h₂  =  5/3

h₁  =  5h₂/3

 Ratios of curved surface area of two cylinders

2Πr₁h₁ : 2Πr₂h₂

(3r₂/2) (5h₂/3) :  r₂ : h₂  

=  5/2 : 1 

=  5 : 2

So, ratio of their curved surface areas is 5 : 2.

Example 4 :

The diameter of the road roller of length 120 cm is 84 cm. If it takes 500 complete revolutions to level play ground, then find the cost of leveling it at the cost of $ 1.5 per square meter.

Solution :

Radius of cylinder (r)  = 84/2

r  =  42 cm

height of cylinder (h) = 120 cm

Area covered by road roller in one revolution = CSA of the road roller 

Curved surface area of cylinder = 2 Π r h

=  2  ⋅ (22/7) ⋅ 42 ⋅ 120 

=  31680 cm²

Area covered by 500 revolutions  =  500 x 31680

=  15840000 cm²

=  1584 m²

Cost of leveling per square meter  =  $1.5 

Required cost  =  1584 x 1.5

=  $2376 

So, required cost is $ 2376.

Example 5 :

The internal and external radii of of a hollow cylinder are 12 cm and 18 cm respectively. If its height is 14 cm, then find its curved surface area.

Solution :

External radius (R)  =  18 cm 

Internal radius (r)  =  12 cm

height (h)  =  14 cm

Curved surface area  =  2Πh (R+r)

=  2 ⋅ (22/7) (14) (18 + 12)

=  2640 cm²

So, curved surface area of cylinder is 2640 cm²

Example 6 :

If the lateral surface of a cylinder is 94.2 sq.cm and its height is 5 cm, then find radius of its base.

Solution :

Lateral surface area of cylinder = 94.2 sq.cm

height = 5 cm

2 Πrh = 94.2

2 · (3.14)  · r  · 5 = 94.2

r = 94.2 / 2(3.14) (5)

r = 3

So, the radius of cylinder is 3 cm.

Example 7 :

A well of 14 m deep is having 2 m in radius. Find the cost of cementing the inner curved surface area at the rate of $2 per m².

Solution :

To find the area to be covered for cementing, we have to find the curved surface area.

Curved surface area = 2 Πrh

Here h = 14 m and r = 2 m

= 2(3.14) (2) (14)

= 175.84 square meter.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.