PROPERTIES OF DEFINITE INTEGRALS

By the second fundamental theorem of integral calculus, the following properties of definite integrals hold. They are stated here without proof.

Property 1 :

Definite integral is independent of the change of variable.

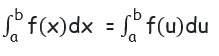
Property 2 :

The value of the definite integral changes by minus sign if the limits are interchanged

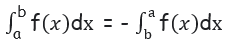
Property 3 :

Property 4 :

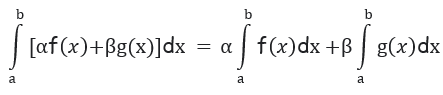
Property 5 :

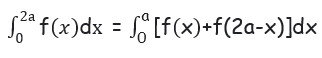
Property 6 :

If f(x) is even function, then

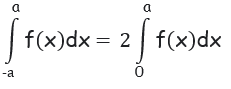
Property 7 :

If f(x) is odd function, then

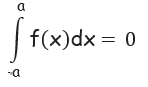
Property 8 :

If f(2a-x) = f(x)

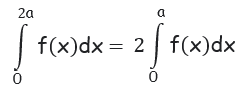
Property 9 :

If f(2a-x) = -f(x)

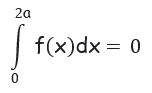
Property 10 :

If f(a-x) = f(x)

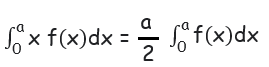
This property help us to remove the factor x present in the integrand of the LHS.

Example Problems Using Properties of Definite Integral

Problem 1 :

Evaluate the following integrals using properties of integration :

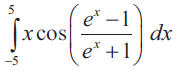
Solution :

Let f(x) = x cos [(e^x-1)/(e^x+1)]

Apply x = -x

f(-x) = -x cos [(e^-x-1)/(e^-x+1)]

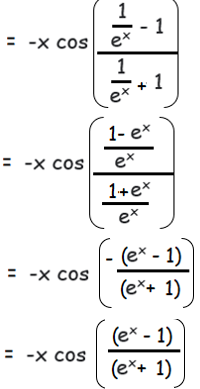
f(-x) = -f(x)

The given function f(x) is odd function. So, the value is 0.

Problem 2 :

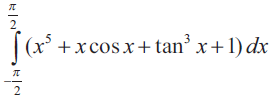
Solution :

Let f(x) = x⁵ + x cos x + tan³x + 1

Apply x = -x

f(-x) = -x⁵ -x cos x - tan³x + 1

f(x) = tan³x

f(-x) = [tan(-x)]³

f(-x) = -tan³x

So, x⁵, x cos x and tan³x are odd functions. By integrating them we will get 0.

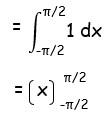
= π/2+π/2

= 2π/2

= π

Problem 3 :

Solution :

Let f(x) = (sin x)²

Apply x = -x

f(-x) = (sin (-x))²

f(-x) = (-sin x)²

f(-x) = sin²x

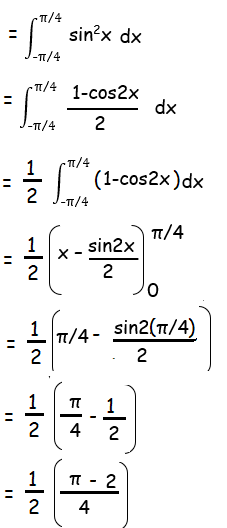
Problem 4 :

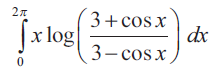
Solution :

Let f(x) = x log [(3+cosx)/(3-cosx)]

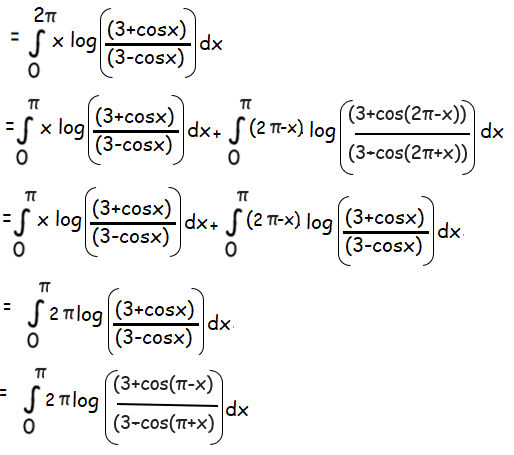
Let g(π-x) = 2π [log (3+cos(π-x))- log (3-cos(π-x))]

= 2π [log (3-cosx) - log (3+cosx)]

= -2π [log (3+cosx) - log (3+cosx)]

g(-x) = -g(x)

So, the value is 0.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.