PROPERTIES OF PARALLEL LINES
Property 1 :
Let m1 and m2 be the slopes of two lines.
If the two lines are parallel, then their slopes will be equal.
That is,
m1 = m2
Property 2 :
Let us consider the general form of equation of a straight line.
ax + by + c = 0
If the two lines are parallel, then their general forms of equations will differ only in the constant term and they will have the same coefficients of x and y.
That is,
ax + by + c1 = 0
ax + by + c2 = 0
Property 3 :
Let us consider the slope intercept form of equation of a straight line.
y = mx + b
If the two lines are parallel, then their slope-intercept form equations will will differ only in the "y"- intercept.
That is,
y = mx + b1
y = mx + b2
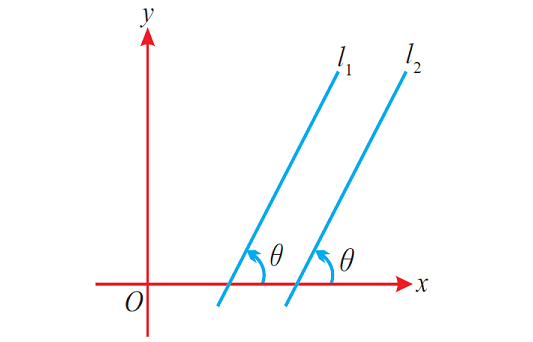
Property 4 :
Let l1 and l2 be two lines.
If the two lines are parallel, the angle between them and the positive side of x-axis will be equal.
The figure given below illustrates the above situation.
Property 5 :
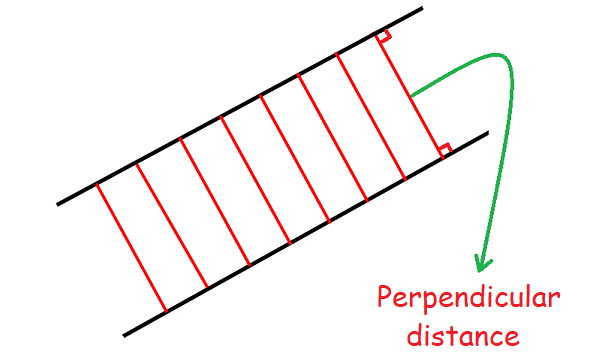
If the two lines are parallel, the perpendicular distance between them will be same at everywhere.
The figure given below illustrates the above situation.
Property 6 :
Let l1 and l2 be two parallel lines and the line m intersects the lines l1 and l2.
The figure shown below illustrates the above situation.
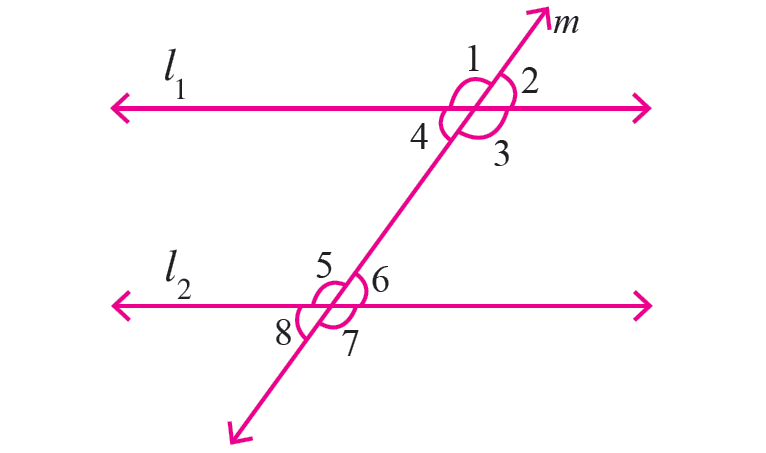
From the above figure, we can have the following important results.
|
Vertically opposite angles are equal. |
∠ 1 = ∠ 3 ∠ 2 = ∠ 4 ∠ 5 = ∠ 7 ∠ 6 = ∠ 8 |
|
Corresponding angles are equal. |
∠ 1 = ∠ 5 ∠ 2 = ∠ 6 ∠ 3 = ∠ 7 ∠ 4 = ∠ 8 |
|
Alternate interior angles are equal. |
∠ 3 = ∠ 5 ∠ 4 = ∠ 6 |
|
Consecutive interior angles are supplementary. |
∠ 3 + ∠ 6 = 180° ∠ 4 + ∠ 5 = 180° |
|
Same side exterior angles are supplementary. |
∠ 1 + ∠ 8 = 180° ∠ 2 + ∠ 7 = 180° |
Practice Problems
Problem 1 :
The slopes of the two lines are 7 and (3k + 2). If the two lines are parallel, find the value of k.
Solution :
If two lines are parallel, then their slopes are equal.
Then,
3k + 2 = 7
Subtract 2 from each side.
3k = 5
Divide each side by 5.
k = 5/3
Problem 2 :
If the following equations of two lines are parallel, then find the value of k.
3x + 2y - 8 = 0
(5k + 3)x + 2y + 1 = 0
Solution :
If the two lines are parallel, then their general forms of equations will differ only in the constant term and they will have the same coefficients of x and y.
To find the value of k, equate the coefficients of x.
5k + 3 = 3
Subtract 3 from each side.
5k = 0
Divide each side by 5.
k = 0
Problem 3 :
Find the equation of a straight line is passing through (2, 3) and parallel to the line 2x - y + 7 = 0.
Solution :
Because the required line is parallel to 2x - y + 7 = 0, the equation of the required line and the equation of the given line 2x - y + 7 = 0 will differ only in the constant term.
Then, the equation of the required line is
2x - y + k = 0 -----(1)
The required line is passing through (2, 3).
Substitute x = 2 and y = 3 in (1).
2(2) - 3 + k = 0
4 - 3 + k = 0
1 + k = 0
k = - 1
So, the equation of the required line is
(1)-----> 2x -y - 1 = 0
Problem 4 :
Verify, whether the following equations of two lines are parallel.
3x + 2y - 7 = 0
y = -1.5x + 4
Solution :
In the equations of the given two lines, the equation of the second line is not in general form.
Let us write the equation of the second line in general form.
y = -1.5x + 4
1.5x + y - 4 = 0
Multiply by 2 on both sides,
3x + 2y - 8 = 0
Now, let us compare the equations of two lines,
3x + 2y - 7 = 0
3x + 2y - 8 = 0
The above two equations differ only in the constant term.
So, the equations of the given two lines are parallel.
Problem 5 :
Verify, whether the following equations of two lines are parallel.
5x + 7y - 1 = 0
10x + 14y + 5 = 0
Solution :
In the equation of the second line 10x + 14y + 5 = 0, the coefficients of x and y have the common divisor 2.
So, divide the second equation by 2
(10x/2) + (14y/2) + (5/2) = (0/2)
5x + 7y + 2.5 = 0
Now, let us compare the equations of two lines,
5x + 7y - 1 = 0
5x + 7y + 2.5 = 0
The above two equations differ only in the constant term.
So, the equations of the given two lines are parallel.
Problem 6 :
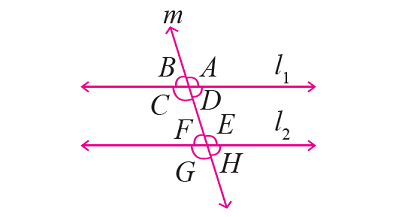
In the figure given below, let the lines l1 and l2 be parallel and m is transversal. If ∠F = 65°, find the measure of each of the remaining angles.
Solution :
From the given figure,
∠F and ∠H are vertically opposite angles and they are equal.
Then, ∠H = ∠F -------> ∠H = 65°
∠H and ∠D are corresponding angles and they are equal.
Then, ∠D = ∠H -------> ∠D = 65°
∠D and ∠B are vertically opposite angles and they are equal.
Then, ∠B = ∠D -------> ∠B = 65°
∠F and ∠E are together form a straight angle.
Then, we have
∠F + ∠E = 180°
Plug ∠F = 65°
∠F + ∠E = 180°
65° + ∠E = 180°
∠E = 115°
∠E and ∠G are vertically opposite angles and they are equal.
Then, ∠G = ∠E -------> ∠G = 115°
∠G and ∠C are corresponding angles and they are equal.
Then, ∠C = ∠G -------> ∠C = 115°
∠C and ∠A are vertically opposite angles and they are equal.
Then, ∠A = ∠C -------> ∠A = 115°
Therefore,
∠A = ∠C = ∠E = ∠G = 115°
∠B = ∠D = ∠F = ∠H = 65°
Problem 7 :
In the figure given below, let the lines l1 and l2 be parallel and t is transversal. Find the value of x.
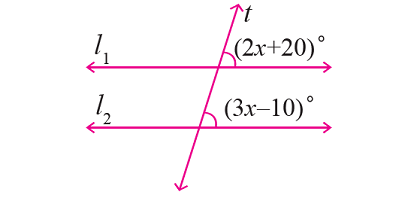
Solution :
From the given figure,
∠(2x + 20)° and ∠(3x - 10)° are corresponding angles.
So, they are equal.
Then, we have
(2x + 20)° = ∠(3x - 10)°
2x + 20 = 3x - 10
Subtract 2x from each side.
20 = x - 10
Add 10 to each side.
30 = x
Problem 8 :
In the figure given below, let the lines l1 and l2 be parallel and t is transversal. Find the value of x.
Solution :
From the given figure, ∠(3x + 20)° and ∠2x° are consecutive interior angles.
So, they are supplementary.
Then, we have
(3x + 20)° + 2x° = 180°
3x + 20 + 2x = 180
Simplify.
5x + 20 = 180
Subtract 20 from each side.
5x = 160
Divide each side by 8.
x = 32
Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here.
If you have any feedback about our math content, please mail us :
v4formath@gmail.com
We always appreciate your feedback.
You can also visit the following web pages on different stuff in math.
WORD PROBLEMS
Word problems on simple equations
Word problems on linear equations
Word problems on quadratic equations
Area and perimeter word problems
Word problems on direct variation and inverse variation
Word problems on comparing rates
Converting customary units word problems
Converting metric units word problems
Word problems on simple interest
Word problems on compound interest
Word problems on types of angles
Complementary and supplementary angles word problems
Trigonometry word problems
Markup and markdown word problems
Word problems on mixed fractrions
One step equation word problems
Linear inequalities word problems
Ratio and proportion word problems
Word problems on sets and venn diagrams
Pythagorean theorem word problems
Percent of a number word problems
Word problems on constant speed
Word problems on average speed
Word problems on sum of the angles of a triangle is 180 degree
OTHER TOPICS
Time, speed and distance shortcuts
Ratio and proportion shortcuts
Domain and range of rational functions
Domain and range of rational functions with holes
Graphing rational functions with holes
Converting repeating decimals in to fractions
Decimal representation of rational numbers
Finding square root using long division
L.C.M method to solve time and work problems
Translating the word problems in to algebraic expressions
Remainder when 2 power 256 is divided by 17
Remainder when 17 power 23 is divided by 16
Sum of all three digit numbers divisible by 6
Sum of all three digit numbers divisible by 7
Sum of all three digit numbers divisible by 8
Sum of all three digit numbers formed using 1, 3, 4
Sum of all three four digit numbers formed with non zero digits
Recent Articles
-
Problems on Surface Area of Cylinder
Jan 15, 21 07:34 AM
Problems on Surface Area of Cylinder
-
Direct and Inverse Variation Practice Worksheet
Jan 13, 21 08:31 AM
Direct and Inverse Variation Practice Worksheet
-
Practice Questions on Direct and Inverse Proportion
Jan 13, 21 07:40 AM
Practice Questions on Direct and Inverse Proportion
