PROVING TRIGONOMETRIC IDENTITIES

Problem 1 :

Prove the following trigonometric identities.

(i) cotθ + tanθ  =  secθ cscθ

Solution :

cotθ  =  cosθ/sinθ

tanθ  =  sinθ/cosθ

L.H.S :

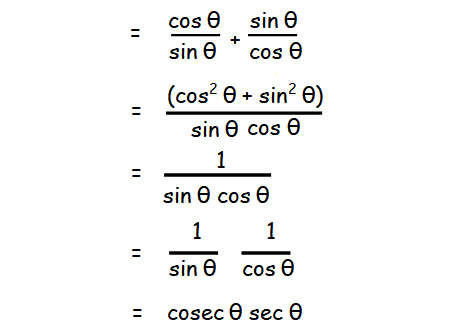
The reciprocal formula for 1/sin θ is cosec θ and 1/cos θ is sec θ.

Hence it is proved.

(ii) tan⁴θ + tan²θ  =  sec⁴θ − sec²θ

Solution :

L.H.S :

tan⁴θ + tan²θ  =  (tan²θ)² + tan²θ

  =  tan²θ(tan²θ + 1)

  =  tan²θ(sec²θ)

  =  (sec²θ - 1)(sec²θ)

  =  sec⁴θ - sec²θ

R.H.S

Hence it is proved.

Problem 2 :

Prove the following identities.

(i)  (1 - tan² θ)/(cot² θ - 1)  =  tan² θ

Solution :

L.H.S :

  =  (1 - tan² θ) / (cot² θ - 1)

Formula for tan θ and cot θ 

cot θ  =  cos θ / sin θ

tan θ  =  sin θ / cos θ

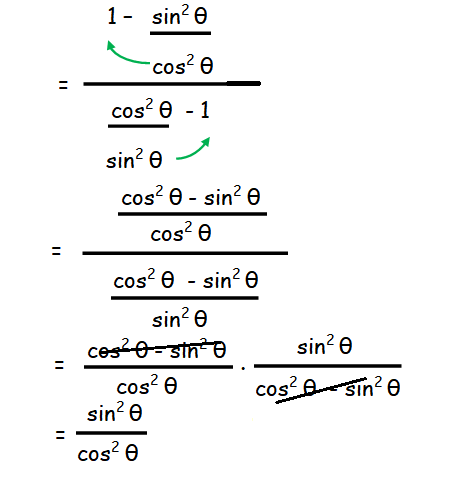
  =  tan² θ

R.H.S

Hence it is proved.

(ii) cos θ/(1 + sin θ)  =  sec θ + tan θ

Solution :

L.H.S :

  =  cos θ/(1 + sin θ) 

By multiplying the conjugate of denominator, we get

  =  [cos θ/(1 + sin θ)] [(1 - sin θ)/(1 - sin θ)]

  =  [cos θ(1 - sin θ)/(1 - sin θ)(1 + sin θ)]

  =  [cos θ(1 - sin θ)/(1 - sin² θ)]

  =  [cos θ(1 - sin θ)/cos² θ]

  =  (1 - sin θ)/cos θ

  =  (1/cos θ) - (sin θ/cos θ)

  =  sec θ - tan θ

R.H.S

Problem 3 :

Prove the following identities.

(i)  √[(1 + sin θ)/(1 - sin θ)]  =  sec θ + tan θ

Solution :

L.H.S :

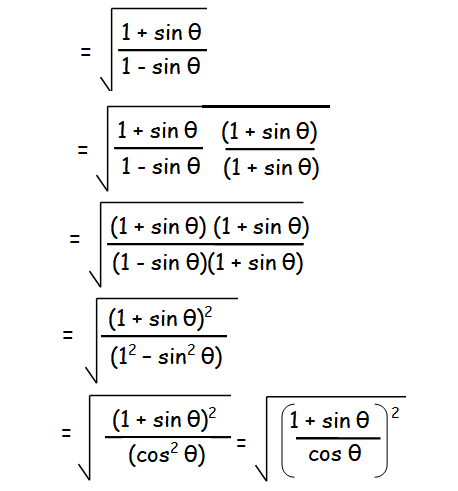
  =  (1/cos θ) + (sin θ/cos θ)

  =  sec θ + tan θ

 R.H.S

Hence proved.

(ii)  [√(1 + sin θ)/(1 - sin θ)] + [√(1 - sin θ)/(1 + sin θ)]  = 2 sec θ

Solution :

L.H.S : 

   =   [√(1 + sin θ)/(1 - sin θ)] + [√(1 - sin θ)/(1 + sin θ)]

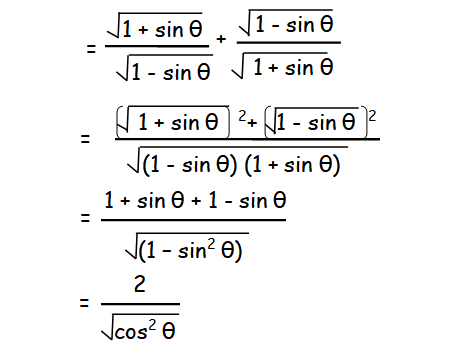
  =  2/cos θ

  =  2 sec θ

R.H.S 

Hence proved .

Problem 4 :

Prove the following identities.

(i) sec⁶ θ = tan⁶ θ + 3 tan² θ sec² θ + 1

Solution :

R.H.S : 

tan⁶ θ + 3 tan² θ sec² θ + 1

  =  (tan² θ)³ + 3 (tan² θ) (sec² θ) + 1

(a + b)³  =  a³ + b³ + 3ab (a + b) 

  =  (tan² θ)³ + 3 (tan² θ) 1 (tan² θ + 1) + 1³

=  (tan² θ + 1)³

=  (sec² θ)³

=  sec⁶ θ

R.H.S

Hence proved.

(ii) (sin θ + sec θ)² + (cos θ + cosec θ)² = 1 + (sec θ + cosec θ)²

Solution :

L.H.S :

  =  (sin θ + sec θ)² + (cos θ + cosec θ)²

  =  sin² θ + sec² θ + 2 sin θ sec θ + cos² θ + cosec² θ + 2 cos θ cosec θ

  =  (sin² θ + cos² θ) + sec² θ  + cosec² θ + 2 (sin θ sec θ + cos θ cosec θ)

  =  1 + sec² θ  + cosec² θ + 2 [(sin θ/cos θ) + (cos θ/sin θ)]

  =  1 + sec² θ  + cosec² θ + 2 [(sin²θ + cos²θ)/cos θ sin θ)]

  =  1 + sec² θ  + cosec² θ + 2 (1/cos θ sin θ)

  =  1 + sec² θ  + cosec² θ + 2 secθ cosec θ 

  =  1 + (sec θ  + cosec θ)²

R.H.S

Hence proved.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.