PROVING TRIGONOMETRY QUESTIONS FOR GRADE 10

Question 1 :

Prove that 

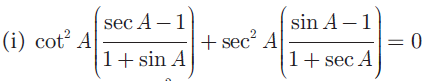
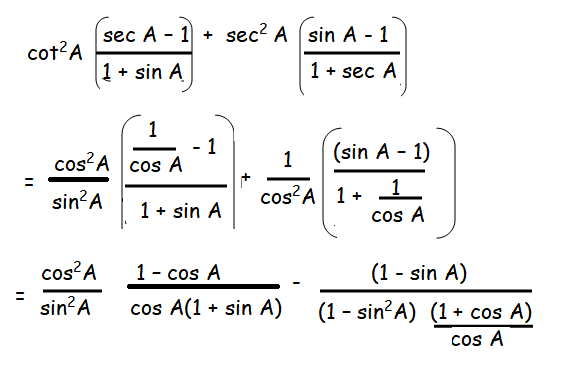
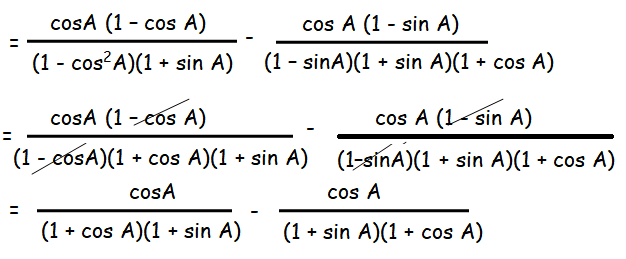
  =  0

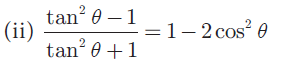
Solution :

L.H.S :

  =  (tan²θ - 1)/(tan²θ + 1)

  =  [(sin²θ/cos²θ) - 1] / [(sin²θ/cos²θ) + 1]

  =  [(sin²θ - cos²θ)/cos²θ] / [(sin²θ + cos²θ)/cos²θ]

  =  [(sin²θ - cos²θ)/cos²θ] ⋅ [cos²θ/1]

  =  sin²θ - cos²θ

  =  1 - cos²θ - cos²θ

  =  1 - 2cos²θ

R.H.S 

Hence proved.

Question 2 :

Prove that 

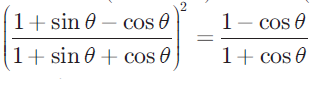
Solution :

[(1 + sin θ - cos θ)/(1 + sin θ + cos θ)]² 

   =   [(1 + sinθ) - cos θ]²/[(1 + sinθ) + cos θ]²

Expanding the numerator and denominator using the formula (a - b)² and (a + b)²

   =   [(1+sinθ)²+cos²θ - 2(1+sin θ)cos θ] / [(1+sinθ)²+cos²θ + 2(1+sin θ)cos θ]

Numerator :

(1 + sinθ)² + cos²θ - 2(1 + sin θ)cos θ

  =  1 + sin²θ + 2sinθ + cos²θ - 2cos θ - 2cos θ sin θ

  =  1 + (sin²θ + cos²θ) + 2sinθ  - 2cos θ - 2cos θ sin θ

  =  2 + 2sinθ  - 2cos θ - 2cos θ sin θ

  =  2(1 + sinθ  - cos θ - cos θ sin θ) 

=  2 [(1 + sinθ) - cos θ(1 + sinθ)]

=  2 [(1 + sinθ) (1 - cos θ)]  -----(1)

Denominator :

[(1+sinθ)²+cos²θ + 2(1+sin θ)cos θ]

  =  1 + sin²θ + 2sinθ + cos²θ + 2cos θ + 2cos θ sin θ

  =  1 + (sin²θ + cos²θ) + 2sinθ  + 2cos θ + 2cos θ sin θ

  =  2 + 2sinθ + 2cos θ + 2cos θ sin θ

  =  2(1 + sinθ + cos θ + cos θ sin θ) 

=  2 [(1 + sinθ) + cos θ(1 + sinθ)]

=  2 [(1 + sinθ) (1 + cos θ)]  -----(2)

(1)/(2)

  =  {2 [(1 + sinθ) (1 - cos θ)]  }/{2 [(1 + sin θ) (1 + cos θ)]}

  =  (1 - cos θ)/(1 + cos θ)

Hence proved.

Question 3 :

If x sin³ θ + y cos³ θ  = sin θ cos θ and x sin θ = y cos θ , then prove that x² +y² = 1 .

Solution :

x sinθ (sin²θ) + (y cosθ)cos²θ = sinθcosθ

x sinθ (sin²θ) + (x sinθ) cos²θ = sinθcosθ

Since x sinθ = y cosθ

x sinθ (sin²θ + cos²θ) = sin θ cosθ

x sinθ = sinθ cosθ

x = cos θ 

y = sinθ

Hence x² + y² = cos²θ + sin²θ = 1. 

Question 4 :

If a cos θ - b sin θ  =  c, then prove that (a sin θ + b cos θ)  =  ± √(a² + b² - c²)

Solution :

a cos θ - b sin θ = c (Squaring on both sides )

a²cos² θ + b² sin² θ - 2 a b sin θ cos θ = c² ----------(1)

Let a sin θ + b cos θ = k  (Squaring on both sides )

b² cos² θ + a² sin² θ + 2ab sin θ cos θ = k² ----------(2)

Adding (1) and (2) we get

a² + b²  = c² + k²

k² = a² + b² - c²

k = √(a² + b² - c²)

a sin θ + b cos θ = √(a² + b² - c²)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.