PUT RATIONAL NUMBERS IN ORDER
- Convert integers, decimals and mixed numbers to fractions.
- Find the least common multiple of all the denominators.
- Using least common multiple, rewrite fractions as equivalent fractions.
- Order the new fractions by the numerator.
Example 1 :
Write the following rational numbers in order from greatest to least.
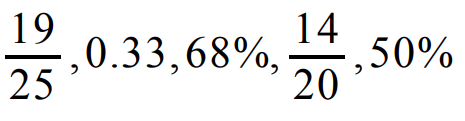
|
19/25 is already a fraction, so we can leave it as it is. |
= 19/25 |
|
For converting the second number 0.33 into fraction, we have to multiply both numerator and denominator by 100. |
0.33 x (100/100) = 33/100 |
|
68% can be written as 68/100 |
= 68/100 |
|
Since 14/20 is already in fractional form, we don't have to convert this |
14/20 |
|
50% can be written as 50/100 |
50/100 |
The denominators of the above fractions are 25, 100, 100, 20 and 100.
Since the denominators are different, we have to find least common multiple.
L.C.M = 100
19/25 (1) ==> (19/25) x (4/4) = 76/100
0.33 (2) ==> (33/100)
68% (3) ==> (68/100)
14/20 (4) ==> (14/20) x (5/5) = 70/100
50% (5) ==> (50/100)
The rational numbers from greatest to least is 76/100, 70/100, 68/100, 50/100, 33/100
Hence the required order is 19/25, 14/20, 68%, 50%, 0.33
Example 2 :
Write the following rational numbers in order from greatest to least.
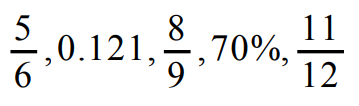
|
5/6 is already a fraction, so we can leave it as it is. |
= 5/6 |
|
For converting the second number 0.121 into fraction, we have to multiply both numerator and denominator by 1000. |
0.121 x (1000/1000) = 121/1000 |
|
8/9 is already a fraction, so we can leave it as it is. |
= 8/9 |
|
70% can be written as 70/100 |
70/100 |
|
11/12 is already a fraction, so we can leave it as it is. |
11/12 |

The denominators of the above fractions are 6, 1000, 9, 100 and 12.
Since the denominators are different, we have to find least common multiple.
L.C.M = 3 x 2 x 5 x 2 x 5 x 10 x 3 = 9000
5/6 (1)==> (5/6) x (1500/1500) = 7500/9000 (3)
0.121 (2)==> 0.121 x (1000/1000) = 121/1000 (5)
8/9 (3)==> (8/9) (1000/1000) = 8000/9000 (2)
70% (4)==> (70/100) x (90/90) = 6300/9000 (4)
11/12 (5)==> (11/12) x (750/750) = 8250/9000 (1)
The rational numbers from greatest to least is 8250/9000, 8000/9000, 7500/9000, 6300/9000, 121/9000
Hence the required order is 11/12, 8/9, 5/6, 70%, 0.121
Example 3 :
Write the following rational numbers in order from least to greatest.
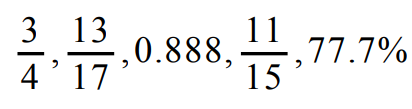
|
3/4 is already a fraction, so we can leave it as it is. |
= 3/4 |
|
13/17 is already a fraction, so we can leave it as it is. |
= 13/17 |
|
To convert 0.888 as fraction, we have to multiply both numerator and denominator by 1000 |
0.888 x (1000/1000) = 888/1000 |
|
11/15 is already a fraction, so we can leave it as it is. |
11/15 |
|
77.7% can be written as 77.7/100. In order to convert this into fraction, we have to multiply both numerator and denominator by 10. |
(77.7/100) x (10/10) = 777/1000 |
The denominators of the above fractions are 4, 17, 1000, 15 and 1000.
Since the denominators are different, we have to find least common multiple.
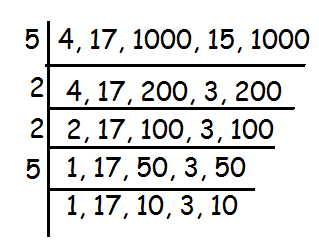
L.C.M = 5 x 2 x 2 x 5 x 17 x 10 x 3 x 10 = 510000
3/4 (1)==>(3/4)x(127500/127500) = 382500/510000 (3)
13/17 (2)==>(13/17)x(30000/30000)=390000/510000 (4)
0.888(3)==>
(8/1000)x(510/510)=4080/510000 (1)
11/15 (4)==>
(11/15)x(34000/34000)=374000/510000 (2)
77.7 (5)==>
(777/1000) x (510/510) = 396270/510000 (5)
The rational numbers from greatest to least is 8250/9000, 8000/9000, 7500/9000, 6300/9000, 121/9000
Hence the required order is 0.888, 11/15, 3/4, 13/17, 77.7%
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Pascal Triangle and Binomial Expansion
Feb 01, 25 10:12 AM
Pascal Triangle and Binomial Expansion - Concept - Examples -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Feb 01, 25 06:26 AM
SAT Math Resources (Videos, Concepts, Worksheets and More) -
Digital SAT Math Problems and Solutions (Part - 106)
Feb 01, 25 06:23 AM
Digital SAT Math Problems and Solutions (Part - 106)

