PYTHAGOREAN THEOREM IN THE COORDINATE PLANE WORKSHEET

Problem 1 :

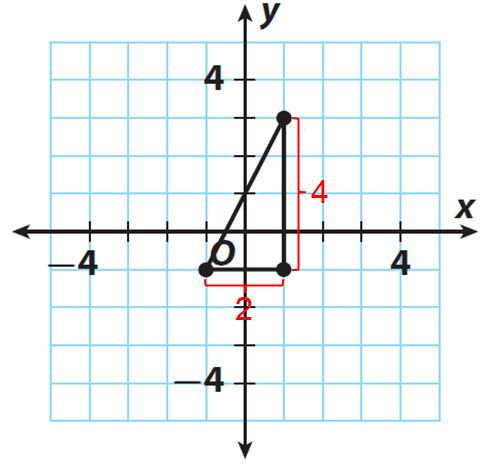
The figure shows a right triangle. Find the approximate length of the hypotenuse to the nearest tenth. Check your answer for reasonableness.

Problem 2 :

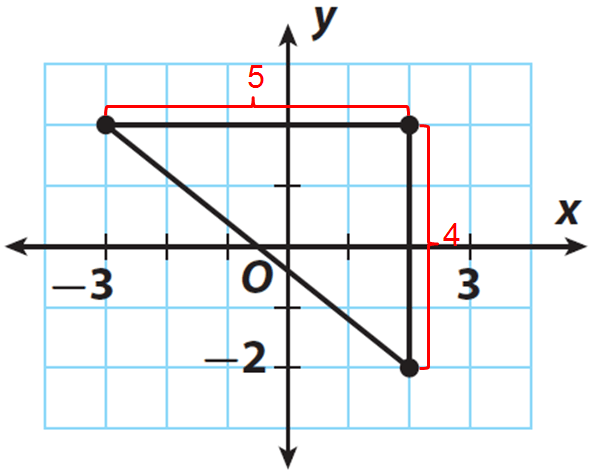
The figure shows a right triangle. Find the approximate length of the hypotenuse to the nearest tenth. Check your answer for reasonableness.

Answers

1. Answer :

Step 1 :

Find the length of each leg.

The length of the vertical leg is 4 units.

The length of the horizontal leg is 2 units. 

Step 2 :

Let a = 4 and b = 2 and c represent the length of the hypotenuse.

Because a and b are legs and c is hypotenuse, by Pythagorean Theorem, we have

a² + b²  =  c²

Step 3 :

Substitute a = 4 and b = 2 in (a² + b²  =  c²⁾ to solve for c. 

4² + 2²  =  c²

Simplify.

16 + 4  =  c²

20  =  c²

Take the square root of both sides.

√20  =  √c²

√20  =  c

Step 4 :

Find the value of √20 using calculator and round to the nearest tenth

4.5  ≈  c

Step 5 :

Check for reasonableness by finding perfect squares close to 20.

√20 is between √16 and √25, so 4 < √20 < 5.

Since 4.5 is between 4 and 5, the answer is reasonable.

The hypotenuse is about 4.5 units long.

2. Answer :

Step 1 :

Find the length of each leg.

The length of the vertical leg is 4 units.

The length of the horizontal leg is 5 units. 

Step 2 :

Let a = 4 and b = 5 and c represent the length of the hypotenuse.

Because a and b are legs and c is hypotenuse, by Pythagorean Theorem, we have

a² + b²  =  c²

Step 3 :

Substitute a = 4 and b = 5 in (a² + b²  =  c²) to solve for c. 

4² + 5²  =  c²

Simplify.

16 + 25  =  c²

41  =  c²

Take the square root of both sides.

√41  =  √c²

√41  =  c

Step 4 :

Find the value of √41 using calculator and round to the nearest tenth

6.4  ≈  c

Step 5 :

Check for reasonableness by finding perfect squares close to 41.

√41 is between √36 and √49, so 6 < √41 < 7.

Since 6.4 is between 6 and 7, the answer is reasonable.

The hypotenuse is about 6.4 units long.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.