FORMATION OF QUADRATIC EQUATION WITH GIVEN ROOTS
If α and β are the two roots of a quadratic equation, then the formula to construct the quadratic equation is
x2 - (α + β)x + αβ = 0
That is,
x2 - (sum of roots)x + product of roots = 0
If a quadratic equation is given in standard form, we can find the sum and product of the roots using coefficient of x2, x and constant term.
Let us consider the standard form of a quadratic equation,
ax2 + bx + c = 0
(a, b and c are real and rational numbers)
Let α and β be the two zeros of the above quadratic equation.
Then the formula to get sum and product of the roots of a quadratic equation :
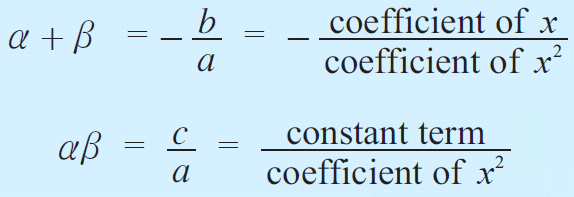
Note :
Irrational roots of a quadratic equation occur in conjugate pairs.
That is, if (m + √n) is a root, then (m - √n) is the other root of the same quadratic equation equation.
Example 1 :
Form the quadratic equation whose roots are 2 and 3.
Solution :
Sum of the roots is
= 2 + 3
= 5
Product of the roots is
= 2 x 3
= 6
Formation of quadratic equation :
x2 - (sum of the roots)x + product of the roots = 0
x2 - 5x + 6 = 0
Example 2 :
Form the quadratic equation whose roots are 1/4 and -1.
Solution :
Sum of the roots is
= 1/4 + (-1)
= 1/4 - 1
= 1/4 - 4/4
= (1 - 4)/4
= -3/4
Product of the roots is
= (1/4) x (-1)
= -1/4
Formation of quadratic equation :
x2 - (sum of the roots)x + product of the roots = 0
x2 - (-3/4)x + (-1/4) = 0
x2 + (3/4)x - 1/4 = 0
Multiply each side by 4.
4x2 + 3x - 1 = 0
Example 3 :
Form the quadratic equation whose roots are 2/3 and 5/2.
Solution :
Sum of the roots is
= 2/3 + 5/2
The least common multiplication of the denominators 3 and 2 is 6.
Make each denominator as 6 using multiplication.
Then,
= 4/6 + 15/6
= (4 + 15)/6
= 19/6
Product of the roots is
= 2/3 x 5/2
= 5/3
Formation of quadratic equation :
x2 - (sum of the roots)x + product of the roots = 0
x2 - (19/6)x + 5/3 = 0
Multiply each side by 6.
6x2 - 19x + 10 = 0
Example 4 :
If one root of a quadratic equation (2 + √3), then form the equation given that the roots are irrational.
Solution :
(2 + √3) is an irrational number.
We already know the fact that irrational roots of a quadratic equation will occur in conjugate pairs.
That is, if (2 + √3) is one root of a quadratic equation, then (2 - √3) will be the other root of the same equation.
So, (2 + √3) and (2 - √3) are the roots of the required quadratic equation.
Sum of the roots is
= (2 + √3) + (2 - √3)
= 4
Product of the roots is
= (2 + √3)(2 - √3)
= 22 - √32
= 4 - 3
= 1
Formation of quadratic equation :
x2 - (sum of the roots)x + product of the roots = 0
x2 - 4x + 1 = 0
Example 5 :
If α and β be the roots of x2 + 7x + 12 = 0, find the quadratic equation whose roots are
(α + β)2 and (α - β)2
Solution :
Given : α and β be the roots of x2 + 7x + 12 = 0.
Then,
sum of roots = -coefficient of x/coefficient of x2
α + β = -7/1
= -7
product of roots = constant term/coefficient of x2
αβ = 12/1
= 12
Quadratic equation with roots (α + β)2 and (α - β)2 is
x2 - [(α + β)2 + (α - β)2]x + (α + β)2(α - β)2 = 0 ----(1)
Find the values of (α + β)2 and (α - β)2.
(α + β)2 = (-7)2
(α + β)2 = 49
(α - β)2 = (α + β)2 - 4αβ
(α - β)2 = (-7)2 - 4(12)
(α - β)2 = 49 - 48
(α - β)2 = 1
So, the required quadratic equation is
(1)----> x2 - [49 + 1]x + 49 ⋅ 1 = 0
x2 - 50x + 49 = 0
Example 6 :
If α and β be the roots of x2 + px + q = 0, find the quadratic equation whose roots are
α/β and β/α
Solution :
Given : α and β be the roots of x2 + px + q = 0.
Then,
sum of roots = -coefficient of x/coefficient of x2
α + β = -p/1
α + β = -p
product of roots = constant term/coefficient of x2
αβ = q/1
αβ = q
Quadratic equation with roots α/β and β/α is
x2 - (α/β + β/α)x + (α/β)(β/α) = 0
x2 - [α/β + β/α]x + 1 = 0 ----(1)
Find the value of (α/β + β/α).
α/β + β/α = α2/αβ + β2/αβ
= (α2 + β2)/αβ
= [(α + β)2 - 2αβ]/αβ
= (p2 - 2q)/q
So, the required quadratic equation is
(1)----> x2 -[(p2 - 2q)/q]x + 1 = 0
Multiply each side by q.
qx2 - (p2 - 2q)x + q = 0
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 150)
Apr 25, 25 11:46 AM
Digital SAT Math Problems and Solutions (Part - 150) -
AP Calculus AB Problems with Solutions (Part - 19)
Apr 24, 25 11:10 PM
AP Calculus AB Problems with Solutions (Part - 19) -
AP Calculus AB Problems with Solutions (Part - 18)
Apr 24, 25 11:06 PM
AP Calculus AB Problems with Solutions (Part - 18)
