RATE OF CHANGE AND SLOPE

A rate of change is a ratio of the amount of change in the dependent variable to the amount of change in the independent variable.

When the rate of change of a relationship is constant, any segment of its graph has the same steepness. The constant rate of change is called the slope of the line

The slope of a line is the ratio of the change in y-values (rise) for a segment of the graph to the corresponding change in x-values (run).

Example 1 :

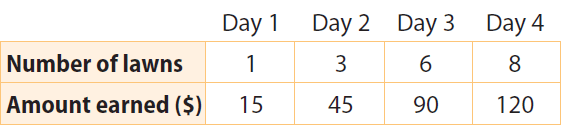
Eve keeps a record of the number of lawns she has mowed and the money she has earned. Tell whether the rates of change are constant or variable.

Solution :

Step 1 :

Identify the independent and dependent variables.

Independent : Number of lawns

Dependent : Amount earned

Step 2 :

Find the rates of change.

Day 1 to Day 2 :

Change in $/Change in lawns  =  (45 - 15)/(3 - 1) 

Change in $/Change in lawns  =  30/2 

Change in $/Change in lawns  =  15

Day 2 to Day 3 :

Change in $/Change in lawns  =  (90 - 45)/(6 - 3) 

Change in $/Change in lawns  =  45/3 

Change in $/Change in lawns  =  15

Day 3 to Day 4 :

Change in $/Change in lawns  =  (120 - 90)/(8 - 6) 

Change in $/Change in lawns  =  30/2 

Change in $/Change in lawns  =  15

The rates of change are constant : $15 per lawn.

Example 2 : 

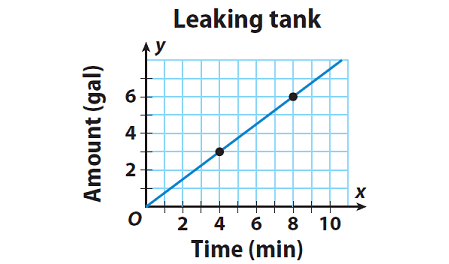
The graph shows the rate at which water is leaking from a tank. The slope of the line gives the leaking rate in gallons per minute. Find the slope of the line.

Solution :

Step 1 :

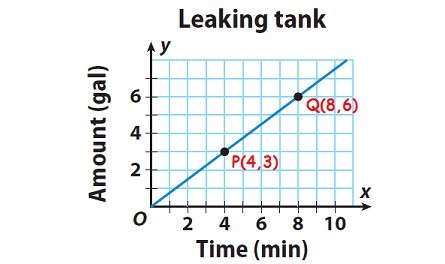
Choose two points on the line.

P(x₁, y₁)  =  P(4, 3)

Q(x₂, y₂)  =  Q(8, 6) 

Step 2 :

Find the change in y-values (rise  =  y₂ - y₁) and the change in x-values (run  =  x₂ - x₁) as you move from one point to the other.

rise  =  y₂ - y₁     run  =  x₂ - x₁

rise  =  3 - 6     run  =  4 - 8

rise  =  -3     run  =  -4

Step 3 :

m  =  rise/run

m  = (y₂ - y₁)/(x₂ - x₁)

m  =  (-3)/(-4)

m  =  3/4

Example 3 : 

The equation y = 375x represents the relationship between x, the time that a plane flies in hours, and y, the distance the plane flies in miles for Plane A. The table represents the relationship for Plane B. Find the slope of the graph for each plane and the plane’s rate of speed. Determine which plane is flying at a faster rate of speed.

Solution :

Step 1 :

Use the equation y  =  375x to find the slope of the graph of Plane A.

Slope  =  Unit rate

Here, unit rate is the distance covered by the plane in one hour.

To find unit rate, substitute x  =  1 in y  =  375x

Slope  =  375(1) 

Slope  =  375 miles/hour

Step 2 :

Use the table to find the slope of the graph of Plane B.

Slope  =  Unit rate 

Slope  =  (850 - 425)/(2 - 1) 

Slope  =  425/1

Slope  =  425 miles/hour.

Step 3 :

Compare the unit rates.

425 > 375

So, Plane B is flying faster.  

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.