RATIO AND PROPORTION IN GEOMETRY

Computing Ratios

If a and b are two quantities that are measured in the same units, then the ratio of a to b is

a/b or a : b

The ratio of a to b can also be written as a : b. Because a ratio is a quotient, its denominator cannot be zero.

Ratios are usually expressed in simplified form. For instance, the ratio of 9 : 6 is usually simplified as 3 : 2.

Simplifying Ratios

Example 1 :

Simplify the ratio :

16 cm/4 m

Solution :

To simplify ratios with unlike units, convert to like units so that the units divide out. Then simplify the fraction, if possible.

16 cm/4 m = 16 cm/4 ⋅ 100 cm

16 cm/4 m = 16 cm/400 cm

16 cm/4 m = 16/400

16 cm/4 m = 1/25 or 1 : 25

Example 2 :

Simplify the ratio :

12 ft/24 in.

Solution :

The given two quantities are in different units.

That is, the first one is in feet and the second in inches.

As we did in the first example, we can convert them into like units and then simplify the fraction, if possible.

12 ft/24 in. = 12 ⋅ 12 in./24 in.

12 ft/24 in. = 144 in./24 in.

12 ft/24 in. = 144/24

12 ft/24 in. = 6/1 or 6 : 1

Using Ratio

Example 3 :

The perimeter of rectangle PQRS shown below is 60 centimeters. The ratio of PQ : QR is 3 : 2. Find the length and width of the rectangle.

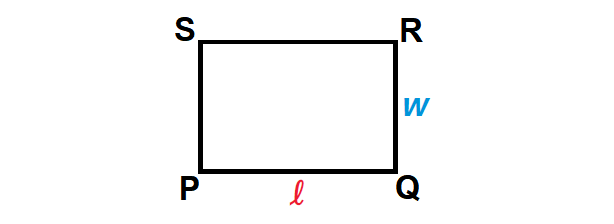
Solution :

Because the ratio of PQ : QR is 3 : 2, we can represent the length PQ as 3x and the width QR as 2x.

2l + 2w = p

Substitute l = 3x and w = 2x.

2(3x) + 2(2x) = 60

Simplify.

6x + 4x = 60

10x = 60

Divide both sides by 10.

x = 6

length = 3 ⋅ 6 = 18 cm

width = 2 ⋅ 6 = 12 cm

Hence, rectangle PQRS has a length of 18 centimeters and a width of 12 centimeters.

Example 4 :

The ratios of the side lengths of ΔABC to the corresponding side lengths of ΔPQR are 2 : 1. Find the unknown lengths.

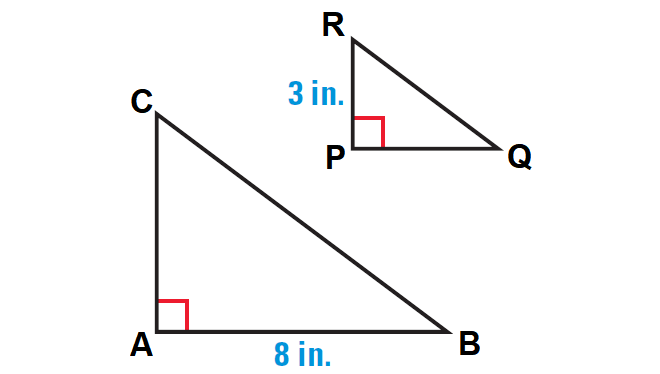
Solution :

From the given information and the diagram shown above, we can consider the following points.

AB is twice PQ and AB = 8, so PQ = 1/2 ⋅ 8 = 4 in.

Using the Pythagorean Theorem, we can determine that QR = 5.

AC is twice PR and PR = 3, so AC = 2 ⋅ 3 = 6 in.

BC is twice QR and QR = 5, so BC = 2 ⋅ 5 = 10 in.

Using Extended Ratios 

Example 5 :

The measure of the angles in ABC are in the extended ratio of 1 : 2 : 3. Find the measures of the angles.

Solution :

Begin by sketching a triangle. Then use the extended ratio of 1 : 2 : 3 to label the measures of the angles as x°, 2x°, and 3x°.

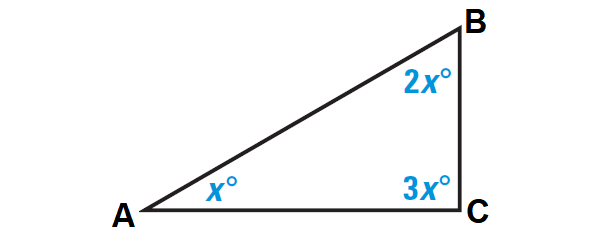
By Triangle Sum Theorem,

x° + 2x° + 3x° = 180°

x + 2x + 3x = 180

Simplify.

6x = 180

Divide both sides by 6.

x = 30

x° = 30°

2x° = 2 ⋅ 30° = 60°

3x° = 3 ⋅ 30° = 90°

Hence, the angle measures are 30°, 60° and 90°.

Proportions

An equation that equates two ratios is a proportion.

For instance, if the ratio a/b is equal to the ratio c/d, then the following proportion can be written :

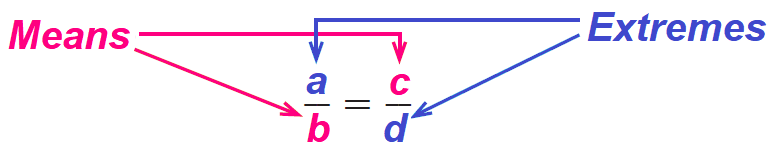
The numbers a and d are the extremes of the proportion.

The numbers b and c are the means of the proportion.

Properties of Proportions

1. Cross Product Property :

The product of the extremes equals the product of the means.

If a/b = c/d, then ad = bc.

2. Reciprocal Property :

If two ratios are equal, then their reciprocals are also equal.

If a/b = c/d, then b/a = d/c.

Solving Proportions

Example 6 :

Solve the proportion :

6/x = 12/5

Solution :

Write the original proportion.

6/x = 12/5

By reciprocal property, we have

x/6 = 5/12

Multiply each side by 6.

6(x/6) = (5/12)6

Simplify.

x = 5/2

Example 7 :

Solve the proportion :

3/(p + 2) = 2/p

Solution :

Write the original proportion.

3/(p + 2) = 2/p

By cross product property, we have

3p = 2(p + 2)

3p = 2p + 4

Subtract 2p from both sides.

p = 4

Using Proportions in Real Life

Example 8 :

The photo below shows a painting. The actual painting is 12 inches high. How wide is it?

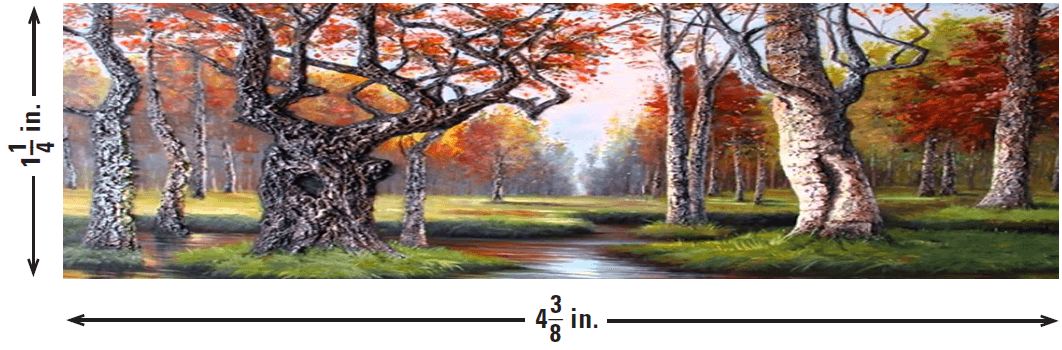
Solution :

We can reason that in the photograph all measurements of the artist’s painting have been reduced by the same ratio.

That is, the ratio of the actual width to the reduced width is equal to the ratio of the actual height to the reduced height.

The photograph is 1¼ inches by 4⅜ inches.

Problem Solving Strategy :

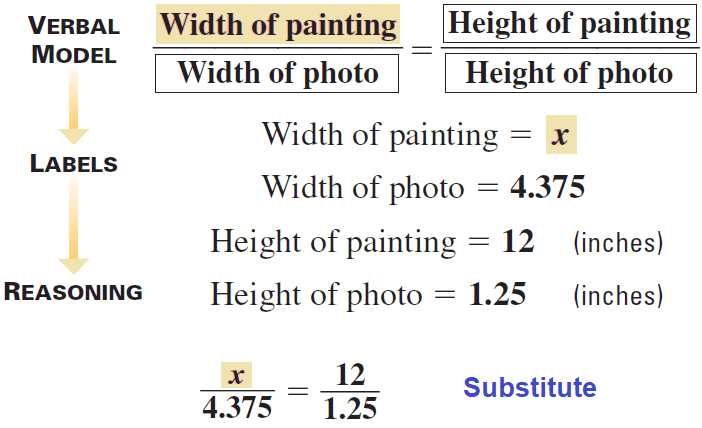
Multiply each side by 4.375.

4.375(x/4.375) = (12/1.25)4.375

Simplify.

x = (12/1.25)4.375

Using calculator, we have

x = 42

So, the actual painting is 42 inches wide.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.