RATIONAL ROOT THEOREM
Ration Root (or Rational Zero) Theorem :
Suppose that all the coefficients of the polynomial function described by
p(x) = anxn + an–1xn–1 + ....................+ a2x2 + a1x + a0
are integers with an ≠ 0 and a0 ≠ 0. If p/q is a root of p(x) in lowest terms, then p is a factor of a0 and q is a factor of an.
Stated another way, the Rational-Root Theorem says that if a simple fraction in lowest terms (a rational number) is a root of a polynomial function with integer coefficients, then the numerator of the rational root is a factor of the constant term of the polynomial, and the denominator of the rational root is a factor of the leading coefficient of the polynomial.
Example 1 :
State the possible rational roots of f(x) and find all rational roots.
f(x) = 5x3 + 29x2 + 19x - 5
Solution :
The constant term is 5 and the leading coefficient is -5.
Factors of -5 : ±1, ±5.
Factors of 5 : ±1, ±5.
So, the possible rational roots are
±1/1, ±1/5, ±5/1, ±5/5
or
±1/1, ±1/5, ±5
Check 1 is rational root to f(x).
f(1) = 5(1)3 + 29(1)2 + 19(1) - 5
= 5 + 29 + 19 - 5
= 5 + 29 + 19 - 5
= 48 ≠ 0
Check -1 is rational root to f(x).
f(-1) = 5(-1)3 + 29(-1)2 + 19(-1) - 5
= -5 + 29 - 19 - 5
= 0
Check 1/5 is rational root to f(x).
f(1/5) = 5(1/5)3 + 29(1/5)2 + 19(1/5) - 5
= 5(1/125) + 29(1/25) + 19/5 - 5
= 1/25 + 29/25 + 19/5 - 5
= 1/25 + 29/25 + 19/5 - 5
= 1/25 + 29/25 + 19/5 - 5
= 0
Check -1/5 is rational root to f(x).
f(1/5) = 5(-1/5)3 + 29(-1/5)2 + 19(-1/5) - 5
= 5(-1/125) - 29(1/25) - 19/5 - 5
= -1/25 - 29/25 - 19/5 - 5
= -1/25 + 29/25 + 19/5 - 5
= 1/25 + 29/25 + 19/5 - 5
= 98/25 ≠ 0
Check 5 is rational root to f(x).
f(5) = 5(5)3 + 29(5)2 + 19(5) - 5
= 625 + 725 + 95 - 5
= 1440 ≠ 0
Check -5 is rational root to f(x).
f(-5) = 5(-5)3 + 29(-5)2 + 19(-5) - 5
= -625 + 725 - 95 - 5
= 0
f(x) becomes zero when
x = -5, -1, 1/5
So, the rational roots of f(x) are -5, -1 and 1/5.
Example 2 :
Use the Rational-Root Theorem to find all rational roots of
f(x) = 4x4 + 3x3 + 4x2 + 11x + 6
Solution :
The constant term is +6 and the leading coefficient is 4.
Factors of 6 : ±1, ±2, ±3, ±6
Factors of 4 : ±1, ±2, ±4
The possible rational roots are
±1, ±2, ±3, ±6, ±1/2, ±3/2, ±1/4, ±3/4
There are sixteen possible rational roots. It's little hard to check each rational root with f(x) as we have done in the previous problem.
So, we can use the graph of the given function to find the actual rational roots.
The smallest possible rational root is –6 and the largest is 6, so graph f on the interval –6 ≤ x ≤ 6 to see where rational roots might be.

The graph above shows that there are two real roots on the interval –1 ≤ x ≤ 0.
Use a graphing utility to find the x-intercepts of the graph of f(x).
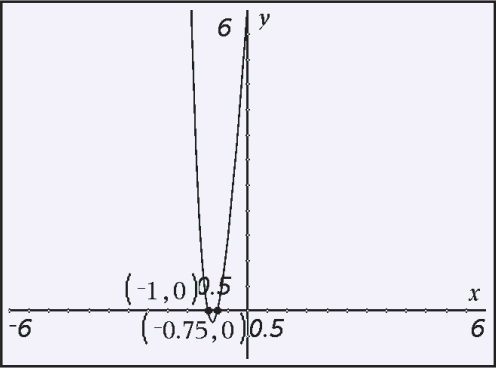
The possible rational roots in the interval –1 ≤ x ≤ 0 are
-1,-3/4, -1/2, -1/4
Test these values with f(x).
f(-1) = 0
f(-3/4) = 0
f(-1/2) ≈ 1.38
f(-1/4) ≈ 3.47
So, –1 and –3/4 are the only rational roots of f(x).
Note :
The Rational-Root Theorem identifies all possible rational roots of a polynomial with integer coefficients. So, any real roots that are not identified must be irrational.
Example 3:
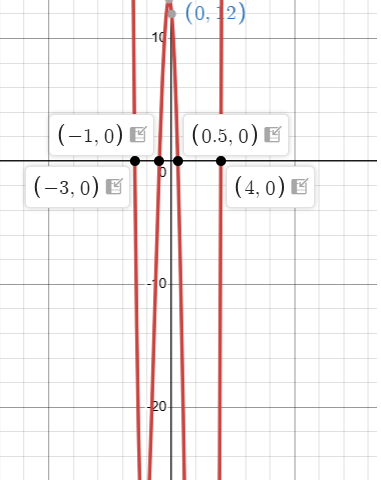
Write out all zeroes of P(x) = 2x4 - x3 - 26x2 - 11x + 12 then sketch the graph of P(x). Clearly show all x- intercepts and y-intercepts.
Solution :
P(x) = 2x4 - x3 - 26x2 - 11x + 12
The constant term is +12 and the leading coefficient is 2.
Factors of 12 : ±1, ±2, ±3, ±4, ±6, ±12
Factors of 2 : ±1, ±2
The possible rational roots are
±1, ±2, ±3, ±1/2, ±1/3, ±1/4, ±1/6, ±1/12, ±2/3
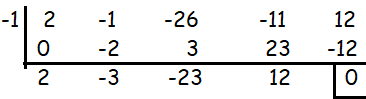
-1 is a solution.

-3 is a solution.
2x2 - 9x + 4 = 0
(2x - 1)(x - 4) = 0
x = 1/2 and x = 4
So, the roots are -1, -3, 1/2 and 4.
To find y-intercept, put x = 0
P(x) = 2x4 - x3 - 26x2 - 11x + 12
P(0) = 2(0)4 - 03 - 26(0)2 - 11(0) + 12
= 12 is the y-intercept.
Example 4:
List all of the possible rational zeros for the given polynomial. Then use synthetic division to locate one of the zeros. Use the quotient to find the remaining zeros.
p(x) = x3 - 6x2 + 11x - 6
Solution :
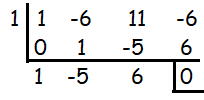
x = 1
x2 - 5x + 6 = 0
x2 - 2x - 3x + 6 = 0
x(x - 2) - 3(x - 2) = 0
(x - 2)(x - 3) = 0
x = 2 and x = 3
So, the zeroes are 1, 2 and 3.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 146)
Apr 18, 25 06:52 AM
Digital SAT Math Problems and Solutions (Part - 146) -
Logarithmic Derivative Problems and Solutions
Apr 16, 25 09:25 PM
Logarithmic Derivative Problems and Solutions -
Digital SAT Math Problems and Solutions (Part - 145)
Apr 16, 25 12:35 PM
Digital SAT Math Problems and Solutions (Part - 145)

