REFERENCE ANGLES WORKSHEET
Find the reference angles for the following angle measures.
1) 5π/3
2) 240°
3) 870°
4) 8π/3
5) -135°
6) -13π/4

1. Answer :
The given angle 5π/3 (or 150°) is less than 2π (or 360°).
The reference angle is the acute angle formed by the terminal side of the angle 5π/3 and the x-axis (see the figure shown below).
The angle 5π/3 has its terminal side in quadrant IV, as shown below.
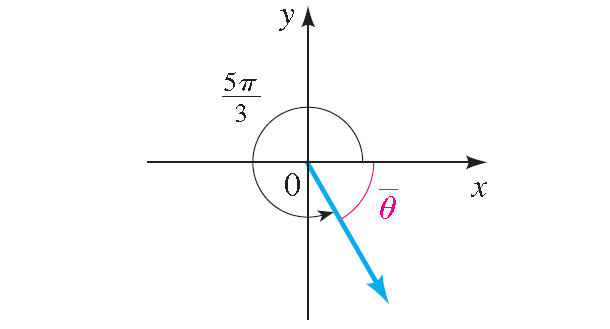
So, the reference angle is
= 2π - 5π/3
= π/3
2. Answer :
The given angle 240° is less than 360°.
The angle 240° has its terminal side in quadrant III, as shown below.
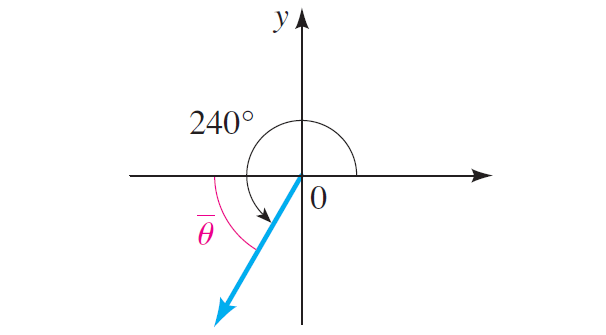
So, the reference angle is
= 240° - 180°
= 60°
3. Answer :
The given angle 870° is greater than 360°.
Find the positive angle between 0° and 360° that is coterminal with 870°.
Divide 870° by 360° and take the remainder.
870° ÷ 360° ---> Remainder = 150°
The positive angle between 0° and 360° that is coterminal with 870° is 150°.
The angle 150° has its terminal side in quadrant III, as shown below.
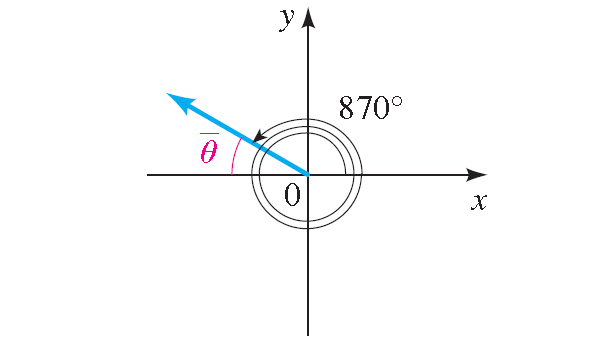
So the reference angle is
= 180° - 150°
= 30°
4. Answer :
The given angle 8π/3 is greater than 2π.
Find the positive angle between 0 and 2π that is coterminal with 8π/3.
To make the process easier, convert 8π/3 radians to degrees.
8π/3 = 8(180°)/3 = 480°
Divide 480° by 360° and take the remainder.
480° ÷ 360° ---> Remainder = 120°
The terminal side of the angle 120° is in quadrant II.
120° ⋅ π/180° = 2π/3 radians
So, the reference angle is
= π - 2π/3
= π/3
5. Answer :
The given angle -135° is negative.
Add multiples of 360° to -135° to make the angle as positive such that it is between 0° and 360°.
-135° + 360° = 225°
225° is positive and less than 360°.
The terminal side of the angle 225° is in quadrant III.
So, the reference angle is
= 225° - 180°
= 45°
6. Answer :
The given angle -13π/4 is negative.
Add multiples of 2π to -13π/4 to make the angle as positive such that it is between 0 and 2π.
-13π/4 + 2(2π) = -13π/4 + 4π = 3π/4
3π/4 is positive and less than 2π.
The terminal side of the angle 3π/4 (or 135°) is in quadrant II.
So, the reference angle is
= π - 3π/4
= π/4
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 146)
Apr 18, 25 06:52 AM
Digital SAT Math Problems and Solutions (Part - 146) -
Logarithmic Derivative Problems and Solutions
Apr 16, 25 09:25 PM
Logarithmic Derivative Problems and Solutions -
Digital SAT Math Problems and Solutions (Part - 145)
Apr 16, 25 12:35 PM
Digital SAT Math Problems and Solutions (Part - 145)

