REPRESENTING A DECIMAL NUMBER
Look at the picture shown below which represents tens, ones and tenths.
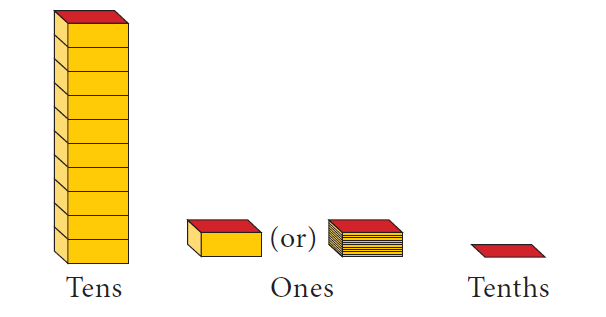
For example, the decimal number 3.2 is 3 ones and 2 tenths that can be represented using the pictorial representations as follows.

Similarly any decimal number can be represented as above.
Try This
Represent the following decimal numbers pictorially.
(i) 5 ones and 3 tenths
(ii) 6 tenths
(iii) 7 ones and 9 tenths
(iv) 6 ones and 4 tenths
(v) Seven tenths
A whole number can be written in expanded form, using the place values of digits in that number.
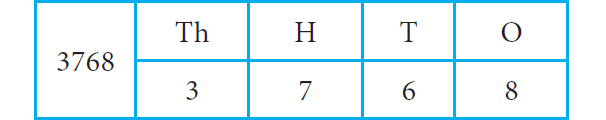
For example, consider the whole number 3768. The place value of 3 is 1000, 7 is 100, 6 is 10 and 8 is 1.
To get the expanded form of a whole number, we have to multiply each digit by the corresponding place value and add all the products.
Then, the expanded form of 3768 is
3x1000 + 7x100 + 6x10 + 8
Now let us extend our learning of place values to decimal digits of a number.
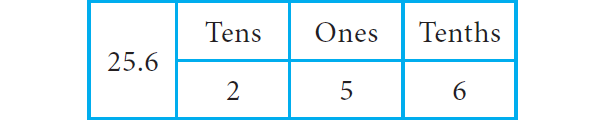
Consider a decimal number 235.68.
Expressing the decimal number in the expanded form, we get
235.68 = 200 + 30 + 5 + 0.6 + 0.08
= 2x100 + 3x10 + 5x1 + ⁶⁄₁₀ + ⁸⁄₁₀₀
= 2x100 + 3x10 + 5x1 + 6x¹⁄₁₀ + 8x¹⁄₁₀₀
The above expression can also be expressed in terms of powers of 10 as follows
235.68 = 2x102 + 3x101 + 5x100 + 6x10-1 + 8x10-2
Hence, in any number, when we move towards right from one digit to the next, the place value of a digit is divided by 101.
Representing the whole number 3768 in the place value grid :
Representing the decimal number 25.6 in the place value grid :
Consider the two lengths 4.5 cm and 4.3cm.
These lengths can also be represented in the place value grid as follows.
4.5 cm = 4 cm and 5 tenths of a cm
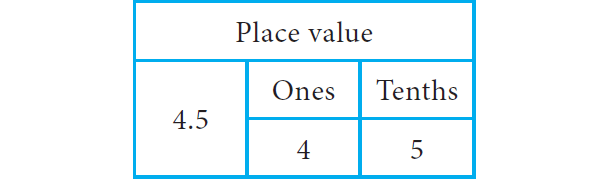
4.3 cm = 4 cm and 3 tenths of a cm
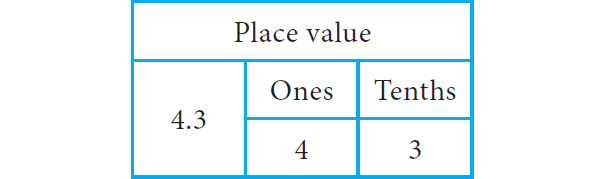
We know that the digit to the right of ones place is known as tenths and the point is called decimal point which separates the integral part and decimal part of a number.
In the above situation, we have expressed numbers with one decimal digit in place value grid. Now to express numbers with two decimal digits in place value grid, let us consider the following example.
Brian has purchased dress materials for Christmas. The length of the pant material is 4 m 75 cm. To express the centimeter in terms of meters, we proceed as follows.
We know that
100 cm = 1 m
1 cm = ¹⁄₁₀₀ m
Therefore one centimeter can be represented as one hundredth of a meter.
Similarly,
75 cm = ⁷⁵⁄₁₀₀ = 0.75 m
So the length of the pant material is 4 + 0.75 m.
That is 4.75 m. It can be read as four and seventy five hundredth of a meter or four point seven five meters.
Note :
The decimal digits of a number have to be read as separate digits.
Try These
1. Express the following decimal numbers in an expanded form and place value grid form.
(i) 56.78 (ii) 123.32 (iii) 354.56
2. Express the following measurements in terms of metre and in decimal form. One is done for you.
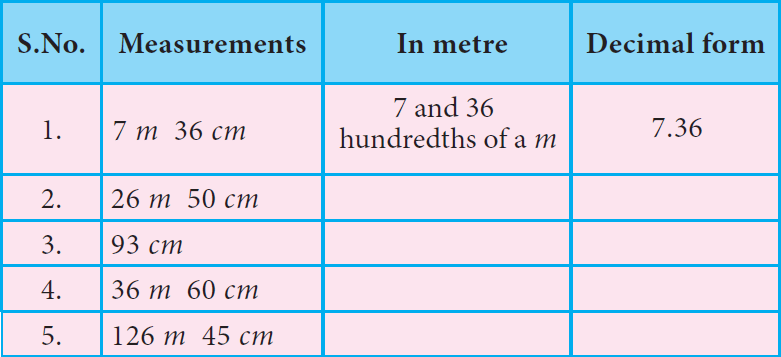
3. Write the following numbers in the place value grid and find the place value of the underlined digits.
(i) 36.37 (ii) 267.06 (iii) 0.23 (iv) 27.69 (v) 53.27
Note that the tenth and hundredth place values of a number can be written as ¹⁄₁₀ and ¹⁄₁₀₀ respectively.
Solved Problems
Problem 1 :
Represent the following decimal numbers pictorially.
0.3, 3.6, 2.7, 11.4
Solution :
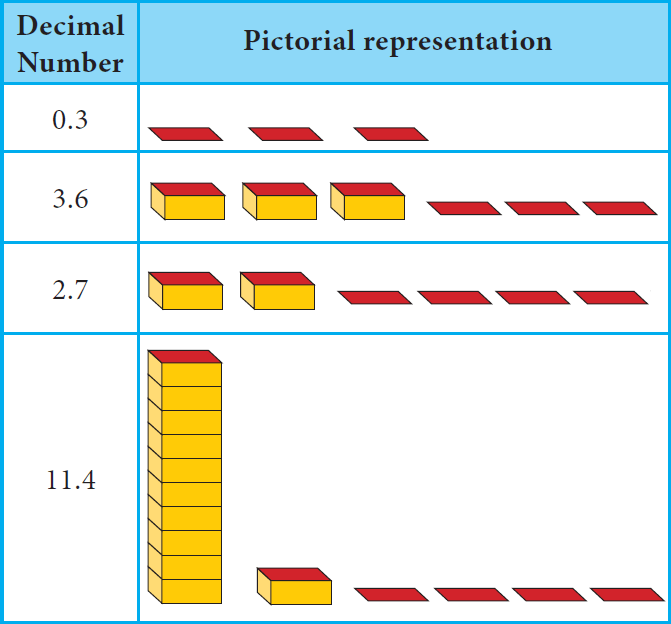
Problem 2 :
Write the following in the place value grid and find the place value of the underlined digits.
(1) 0.37, (2) 2.73, (3) 28.271
Solution :
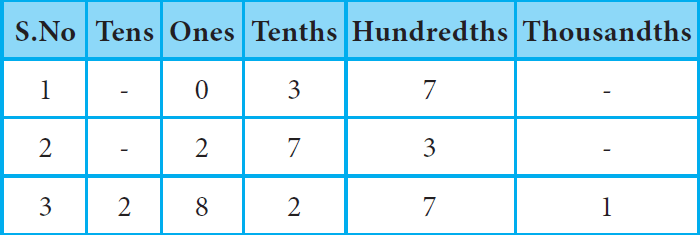
(1) The place value of 7 in 0.37 is Hundredth.
(2) The place value of 7 in 2.73 is Tenth.
(3) The place value of 7 in 28.271 is Hundredth.
Problem 3 :
Express the numbers given in expanded form in the place value grid. Also write its decimal representation.
(i) 3 + ⁵⁄₁₀ + ³⁄₁₀₀ + ⁴⁄₁₀₀₀
(ii) 40 + 6 + ⁷⁄₁₀ + ²⁄₁₀₀ + ⁶⁄₁₀₀₀
Solution :
(i) :

3 + ⁵⁄₁₀ +³⁄₁₀₀ +⁴⁄₁₀₀₀ = 3.534
(ii) :

40 + 6 + ⁷⁄₁₀ + ²⁄₁₀₀ + ⁶⁄₁₀₀₀ = 46.726
Problems 4-5 : Write the decimal numbers for the given pictorial representation of numbers.
Problem 4 :
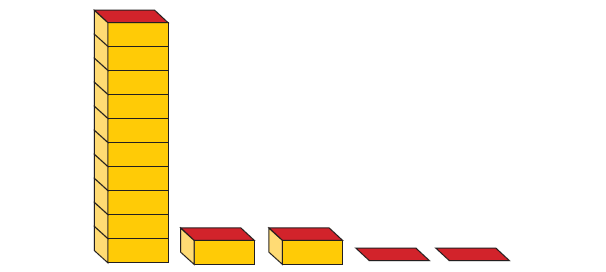
Solution :
10 + 1 + 1 + ¹⁄₁₀ + ¹⁄₁₀ = 12 + 0.1 + 0.1
= 12.2
Problem 5 :
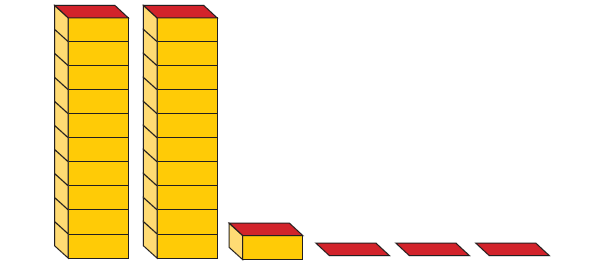
Solution :
10 + 10 + 1 + ¹⁄₁₀ + ¹⁄₁₀ + ¹⁄₁₀ = 21 + 0.1 + 0.1 + 0.1
= 21.3
Problem 6 :
Fill in the following place value table.
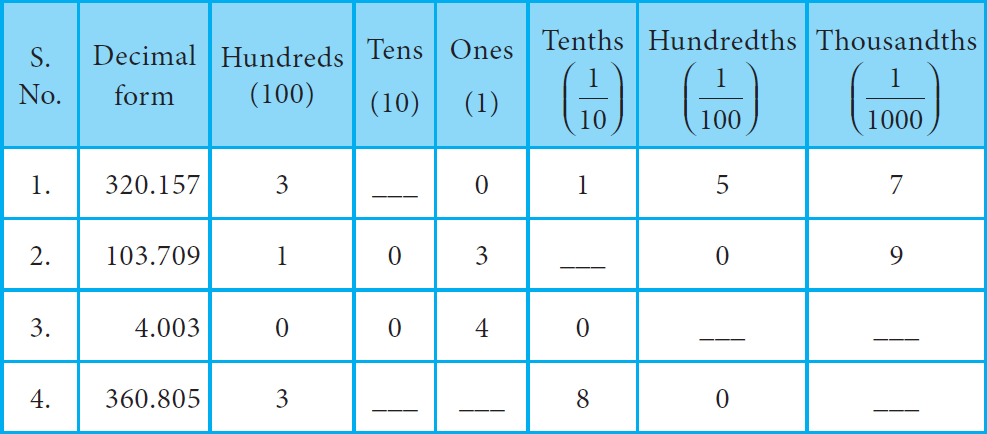
Solution :
(1) Tens : 2
(2) Tenths : 7
(3) Hundredths : 0, Thousandths : 3
(4) Tens : 6, Ones : 0, Thousandths : 5
Problem 7 :
Write the decimal numbers from the following place value table.
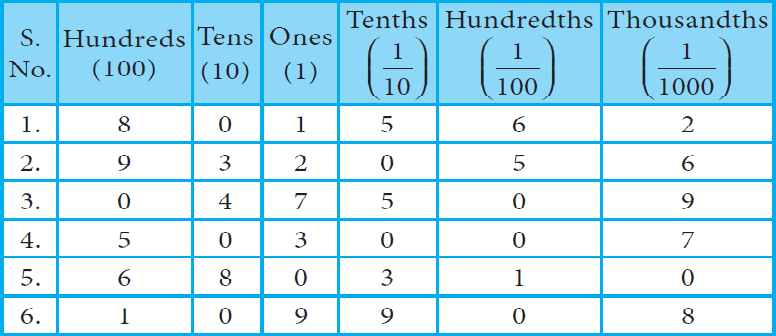
Solution :
(1) 801.562
(2) 932.056
(3) 47.509
(4) 503.007
(5) 680.310
(6) 109.908
Problems 8-10 : Write the shaded portion of the figures given below as a fraction and as a decimal number.
Problem 8 :
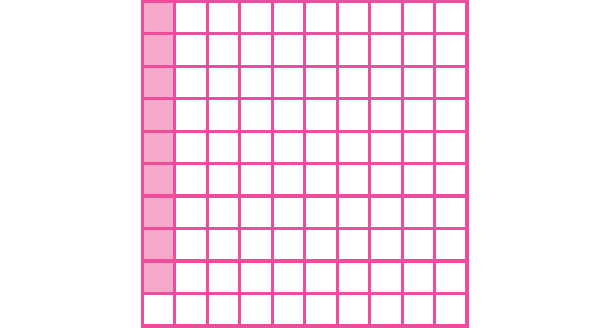
Solution :
9 squares out of 100 squares are shaded.
= ⁹⁄₁₀₀
= 0.09
Problem 9 :
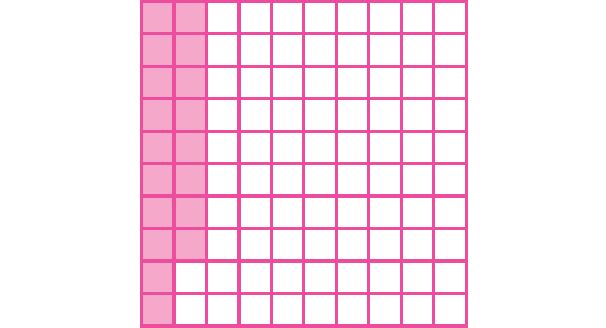
Solution :
18 squares out of 100 squares are shaded.
= ¹⁸⁄₁₀₀
= 0.18
Problem 10 :
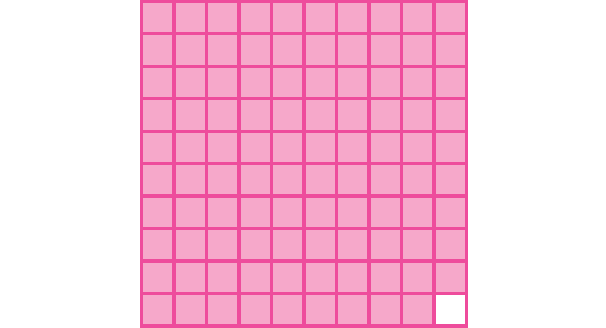
Solution :
99 squares out of 100 squares are shaded.
= ⁹⁹⁄₁₀₀
= 0.99
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 134)
Apr 02, 25 12:40 AM
Digital SAT Math Problems and Solutions (Part - 134) -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Apr 02, 25 12:35 AM
SAT Math Resources (Videos, Concepts, Worksheets and More) -
Digital SAT Math Problems and Solutions (Part 135)
Apr 02, 25 12:32 AM
Digital SAT Math Problems and Solutions (Part 135)

