SECOND DERIVATIVE TEST FOR LOCAL EXTREMA

Problem 1 :

Find intervals of concavity and points of inflexion for the following functions:

(i) f (x) = x(x − 4)³

(ii)  y  =  sin x + cos x, 0 < x < 2π

f(x)  =  1/2 (e^x-e^-x)

Solution

Problem 2 :

Find the local extrema for the following functions using second derivative test :

(i)  f(x) = −3x⁵+ 5x³

(ii)  f(x)  =  x logx

(iii)  f(x)  =  x²e^-2x               Solution

Problem 3 :

For the function

f(x)  =  4x³+3x²-6x+1

find the intervals of 

(i)  monotonicity

(ii)  local extrema

(iii)  intervals of concavity and

(iv)  points of inflection.      Solution

Problem 4 :

What are the coordinates of the points of inflection for the graph of f(x) = sin²x on the closed interval [0, π] ?

a) x = 3π/4 only 

b)  x = π/4, x = π/2 and x = 3π/4

c)  x = π/4 and x = 3π/4

d) x = π/2             e) x = π/4

Solution

Problem 5 :

The function g is defined by the equation

g(x) = 6x⁵ - 10x³

Determine the values of x for which the graph of function g is concaved upwards

a) x > 1/2     b) -√2/2 < x < 0 or x > √2/2

c)  -1/2 < x < 0 or x > 1/2      d) -1/2 < x < 1/2

e) -√2/2 < x < √2/2

Solution

Application of first derivatives

Problem 1 :

If the volume of a cube of side length x is v = x³ . Find the rate of change of the volume with respect to x when x = 5 units.

Solution

Problem 2 :

If the mass m(x) (in kilograms) of a thin rod of length x (in meters) is given by, m(x) = √3x then what is the rate of change of mass with respect to the length when it is x = 3 and x = 27 meters

Solution

Problem 3 :

A stone is dropped into a pond causing ripples in the form of concentric circles. The radius r of the outer ripple is increasing at a constant rate at 2 cm per second. When the radius is 5 cm find the rate of changing of the total area of the disturbed water?

Solution

Problem 4 :

A beacon makes one revolution every 10 seconds. It is located on a ship which is anchored 5 km from a straight shore line. How fast is the beam moving along the shore line when it makes an angle of 45° with the shore?

Solution

Problem 5 :

If a ball is thrown into the air with a velocity of 40 ft/s, its height in feet after t seconds is given by

y = -16t² + 40t

a) Find the average velocity for the time period beginning when t = 2 lasting 0.5 s 

b) Find the instantaneous velocity when t = 2.

Solution

Problem 6 :

If an arrow is shot upward on the moon with a velocity of 58 m/s, its height in meters after t seconds is given by:

h = 58t - 0.83t²

a) Find the velocity of the arrow at 1 s.

b) Find the velocity of the arrow when t=a.

c) When will the arrow hit the moon?

d) With what velocity will the arrow hit the moon?

Solution

Problem 7 :

Temperature readings (in degrees C) where recorded every hour starting at 5:00 am on a day in April in Whitefish, Montana. This table shows some of the readings:

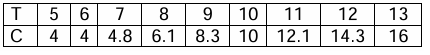
Find the rate of average rate of change for each change in time:

a) 8:00 am to 11:00 am

b) 8:00 am to 10:00 am

c) 8:00 am to 9:00 am

Solution

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.