SETS OF REAL NUMBERS

A group of items is called a set. A Venn diagram uses intersecting circles to show relationships among sets of numbers or things.

The set of real numbers consists of the set of rational numbers and the set of irrational numbers.

The picture given below clearly illustrates this.

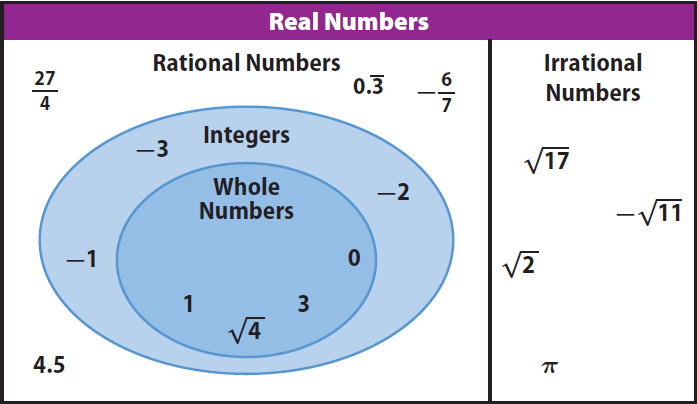
When a set is contained within a larger set in a Venn diagram, the numbers in the smaller set are also members of the larger set.

When we classify a number, we can use the Venn diagram to help figure out which other sets, if any, it belongs to.

Example 1 : 

Classify the number given below by naming the set or sets to which it belongs.

37

Answer : 

Whole, Integer, Rational

37 is a whole number.

All whole numbers are integers. All integers are rational numbers.

Example 2 : 

Classify the number given below by naming the set or sets to which it belongs.

-98

Answer : 

Integer, Rational

-98 is an integer.

All integers are rational numbers.

Example 3 : 

Classify the number given below by naming the set or sets to which it belongs.

√5

Answer : 

Irrational

5 is in square root. It is a whole number, but it is not a perfect square. 

So, √5 is irrational.

Example 4 : 

Classify the number given below by naming the set or sets to which it belongs.

-56.12

Answer : 

Rational

-56.12 is a rational number.

It is not a whole number because it is negative. It is not an integer because there are non-zero digits after the decimal point.

Example 5 : 

Classify the number given below by naming the set or sets to which it belongs.

2√3

Answer : 

Irrational

We have 3 in square root. 3 is a whole number, but it is not a perfect square. 

So, √3 is irrational. 

We already know the fact, if an irrational number is multiplied by a rational number, the product is irrational. 

So, 2√3 is irrational.

Example 6 : 

Classify the number given below by naming the set or sets to which it belongs.

7/8

Answer : 

Rational

7/8 is a rational number.

It is not a whole number, because it is a fraction of a whole number. It is not an integer because it is not a whole number or the opposite of a whole number.

Example 7 : 

Classify the number given below by naming the set or sets to which it belongs.

102.353535......

Answer : 

Rational

102.353535........is a rational number.

Since 102.353535...... is a recurring decimal, it can be converted into fraction. And also it is not a whole number or integer, because it is a fraction. 

Example 8 : 

Classify the number given below by naming the set or sets to which it belongs.

√25

Answer : 

Whole, Integer, Rational

25 is in square root. 25 is a whole number and also it is a perfect square. 

So, we have

√25  =  √(5x5)  =  5

So, √25 is whole, integer, rational.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.