SIMILAR TRIANGLES PROBLEMS WITH ANSWERS

Problem 1 :

P and Q are points on sides AB and AC respectively, of triangle ABC. If AP = 3 cm, PB = 6 cm, AQ = 5 cm and QC = 10 cm, show that BC = 3 PQ.

Solution :

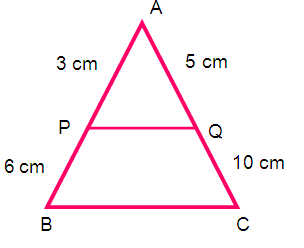
AP/PB  =  AQ/AC

3/9  =  5/15

1/3  =  1/3

In triangles APQ, and ABC we get

(AP/AB)  =  (AQ/AC)

∠ A  =  ∠A

By using SAS criterion ∆ APQ ~ ∆ ABC

(AP/AB)  =  (Q/AC)  =  (PQ/BC)

(AP/PB)  =  (PQ/BC)

PQ/BC  =  3/9

PQ/BC  =  1/3

3 PQ  =  BC

BC  =  3 PQ 

Problem 2 :

In triangle ABC, AB = AC and BC = 6 cm. D is a point on the side AC such that AD = 5 cm and CD = 4 cm. Show that triangle BCD and triangle ACB are congruent and hence find BD.

Solution :

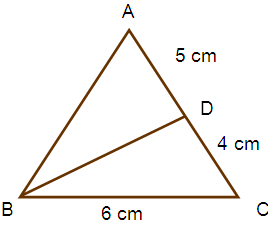
In triangle ABC, AB = AC

(BC/AC)  =  (CD/CB)

 (6/9)  =  (4/6)

From the triangles ∆ BCD, ∆ ACB we get

(BC/AC) = (CD/CB)

∠ C = ∠ C (Common angle)

By using SAS criterion, we get

∆ BCD ~ ∆ ACB

(BD/AB)  =  (BC/AC)

Here,  AB  =  AC

BD/AC  =  6/9

BD/9  =  6/9

BD  =  6 cm

Problem 3 :

The points D and E are on the sides AB and AC of triangle ABC respectively, such that DE and BC are parallel. If AB = 3 AD and the area triangle ABC is 72 cm², then find the area of the quadrilateral DBCE.

Solution :

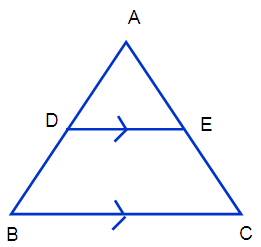
From the diagram, 

the sides DE and BC are parallel and AB = 3 AD

(AD/AB)  =  1/3

By taking the two triangles,  ∆ ADE and ∆ ABC

∠ ADE  =  ∠ ABC

∠A  =  ∠A (Common angle)

By using AA similarity criterion ∆ADE ~ ∆ABC

Area of ∆ ADE/Area of ∆ ABC  =  AD²/AB²

Area of ∆ ADE/72  =  1/9

Area of ∆ ADE   =  (1/9) ⋅ 72  =  8 cm

Area of quadrilateral  =  area of ∆ ABC – Area of ∆ DE

  =  72 – 8 

=  64 cm²

Therefore the required area of quadrilateral is 64 cm².

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.