SOLVE INEQUALITIES

An open sentence that contains the symbol <, >, ≤ or ≥  is called an inequality. Inequalities can be solved in the same way as equations.

Difference Between Equation and Inequality

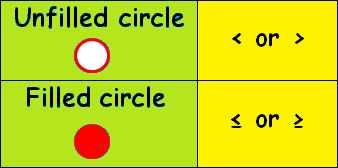
Solving Inequalities - Concept

• If we have the inequality < (less than) or > (greater than), we have to use the empty circle.
• If we have the inequality sign ≤ (less than or equal to) or ≥ (greater than or equal to), we have to use the filled circle.

Solve Inequalities - Examples

Example 1 :

Solve each inequality. Then check your solution, and graph it on a number line.

x + 14 ≥ 18

Solution : 

Step 1 :

x + 14 ≥ 18

Subtract 14 on both sides,

x + 14 - 14 ≥ 18 - 14

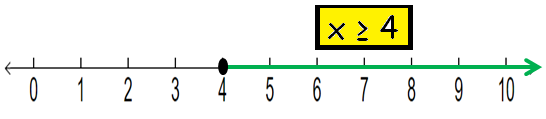
x ≥ 4

Step 2 :

To check the solution, we need to take any values greater than or equal to 4 and check whether it satisfies the condition or not.

Let us take x  =  5

Now we have to apply 5 instead of "x" in the given inequality.

5 + 14 ≥ 18

19 ≥ 18 (True)

Step 3 :

To graph the solution, we have to draw a number line and shade the portion which satisfies the given condition.

Example 2 :

Solve each inequality. Then check your solution, and graph it on a number line.

d + 5 ≤ 7

Solution : 

Step 1 :

d + 5 ≤ 7

Subtract 5 on both sides,

d + 5 - 5 ≤ 7 - 5

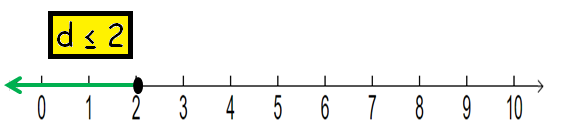
d  ≤ 2

Step 2 :

To check the solution, we need to take any value less than or equal to 2 and check whether it satisfies the condition or not.

Let us take d  =  0

Now we have to apply 0 instead of "d" in the given inequality.

0 + 5 ≤ 7

 5 ≤ 7 (True)

Step 3 :

To graph the solution, we have to draw a number line and shade the portion which satisfies the given condition.

Example 3 :

Solve each inequality. Then check your solution, and graph it on a number line.

-3  ≥  q - 7

Solution : 

Step 1 :

-3  ≥  q - 7

Add 7 on both sides

-3 + 7  ≥  q - 7 + 7

 7  ≥  q 

If we flip the variable to the right side and value to the left side, then we have to change its original sign.

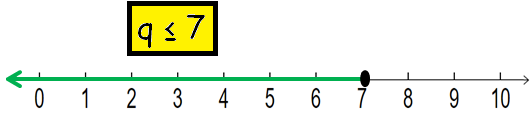
 q ≤ 7 

Step 2 :

To check the solution, we need to take any value lesser than or equal to 7 and check whether it satisfies the condition or not.

Let us take q  =  5

Now we have to apply 5 instead of "q" in the given inequality.

-3  ≥  5 - 7

-3  ≥  - 2 (True)

Step 3 :

To graph the solution, we have to draw a number line and shade the portion which satisfies the given condition.

Example 4 :

Solve each inequality. Then check your solution, and graph it on a number line.

2y  >  -8 + y

Solution : 

Step 1 :

2y  >  -8 + y

Subtract y on both sides

2y - y  >  -8 + y - y

 y  >  -8

Step 2 :

To check the solution, we need to take any value greater than -8.

Let us take y  =  -5

Now we have to apply -5 instead of "y" in the given inequality.

2(-5)  >  -8 - 5

-10 > -13 (True)

Step 3 :

To graph the solution, we have to draw a number line and shade the portion which satisfies the given condition.

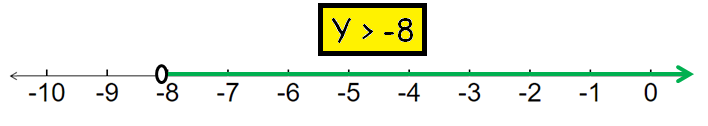
Example 5 :

Solve each inequality. Then check your solution, and graph it on a number line.

3f < -3 + 2f

Solution : 

Step 1 :

3f < -3 + 2f

Subtract 2f on both sides

3f - 2f  <  -3 + 2f - 2f

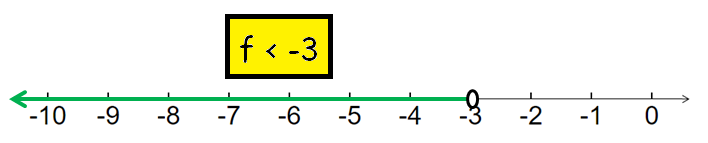
 f  <  -3

Step 2 :

To check the solution, we need to take any value lesser than -2.

Let us take f  =  -2

Now we have to apply -2 instead of "f" in the given inequality.

3(-2) < -3 + 2(-2) 

-6 < -3 - 4 

-6 < -7 (True)

Step 3 :

To graph the solution, we have to draw a number line and shade the portion which satisfies the given condition.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.