SOLVING COMPOUND INEQUALITIES

Compound Inequalities with "And"

A compound inequality containing and is true only if both inequalities are true. Thus, the graph of a compound inequality containing and is the intersection of the graphs of the two inequalities.

In other words, the solution must be a solution of both inequalities.

The intersection can be found by graphing each inequality and then determining where the graphs overlap.

Compound Inequalities with "Or"

Another type of compound inequality contains the word or. A compound inequality containing or is true if one or more of the inequalities is true. The graph of a compound inequality containing or is the of the graphs of the two inequalities.

In other words, the solution of the compound inequality is a solution of either inequality, not necessarily both. The union can be found by graphing each inequality.

Example 1 :

Graph the solution set of x < 3 and x ≥ -2

Solution :

Let us draw the graph for the first given inequality x < 3. In the number line we have to shade the portion which is lesser than 3.

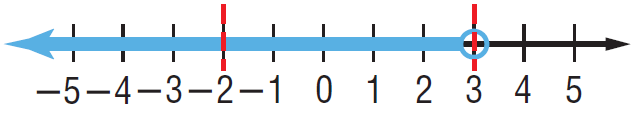
Let us draw the graph for the first given inequality x ≥ -2. In the number line we have to shade the portion which is greater than or equal to -2.

The overlaps of the above two graphs is between -2 and 3.

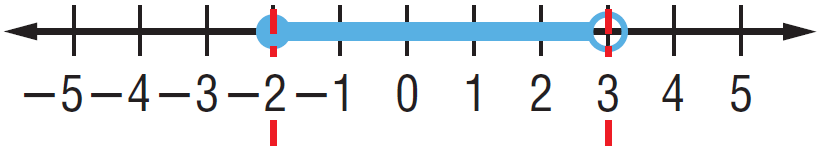
Example 2 :

Solve -3h + 4 < 19 or 7h - 3 > 18. Then graph the solution set.

Solution :

-3h + 4 < 19

Subtract 4 on both sides

-3h + 4 - 4 < 19 - 4

-3h < 15

Divide by -3 on both sides,

-3h/(-3) < 15/ (-3)

h < -5

7h - 3 > 18.

Add 3 on both sides,

7h - 3 + 3 > 18 + 3

7h > 21

Divide by 7 on both sides

7h/7 > 21/7

h > 3

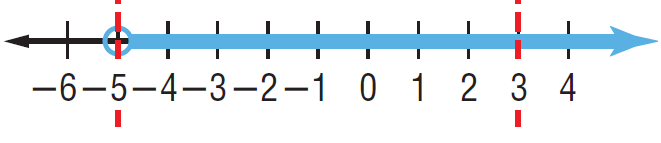
Let us draw the graph for the first given inequality h> 3. In the number line we have to shade the portion which is greater than 3.

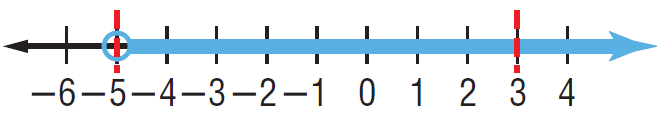
Let us draw the graph for the first given inequality x < -5. In the number line we have to shade the portion which is lesser than -5.

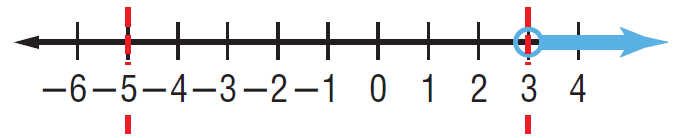
By combining the above two graphs, we get

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.

SOLVING COMPOUND INEQUALITIES

Compound Inequalities with "And"

Compound Inequalities with "Or"

Recent Articles

Precalculus Problems and Solutions (Part - 1)

Precalculus Problems and Solutions (Part - 12)

Precalculus Problems and Solutions (Part - 11)