SOLVING INEQUALITIES WITH VARIABLES ON BOTH SIDES
Some inequalities have variable terms on both sides of the inequality symbol. You can solve these inequalities like you solved equations with variables on both sides.
Use the properties of inequality to “collect” all the variable terms on one side and all the constant terms on the other side.
Solving Inequalities with Variables on Both Sides
Solve each inequality and graph the solutions :
Example 1 :
4x + 10 ≤ 3x + 95
Solution :
4x + 10 ≤ 3x + 95
To collect the variable terms on one side, subtract 2m from each side, subtract 3x from each side.
x + 10 ≤ 95
Because 10 is added to x, subtract 100 from each side to undo the addition.
x ≤ 85
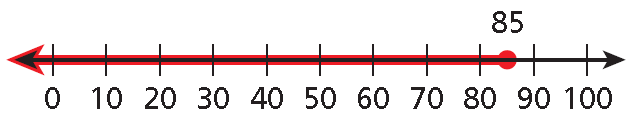
Example 2 :
3m < 5m + 8
Solution :
3m < 5m + 8
To collect the variable terms on one side, subtract 2m from each side.
0 < 2m + 8
Because 8 is added to 2m, subtract 8 from each side to undo the addition.
-8 < 2m
Because m is multiplied by 2, divide each side by 2 to undo the multiplication.
-4 < m
m > -4
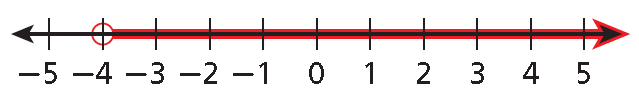
Example 3 :
5k - 1 ≤ 2.5k + 4
Solution :
5k - 1 ≤ 2.5k + 4
To collect the variable terms on one side, subtract 2m from each side, subtract 2.5k from each side.
2.5k - 1 ≤ 4
Because 1 is subtracted from 2.5k, add 1 to each side to undo the subtraction.
2.5k ≤ 5
Because k is multiplied by 2.5, divide each side by 2.5 to undo the multiplication.
k ≤ 2

Simplifying Each Side Before Solving
Solve each inequality and graph the solutions.
Example 4 :
6(1 - m) < 3m
Solution :
6(1 - m) < 3m
Distribute 6 on the left side of the inequality.
6 - 6m < 3m
Add 6m to each side so that the coefficient of m is positive.
6 < 9m
Because m is multiplied by 9, divide each side by 9 to undo the multiplication.
2/3 < m
m > 2/3
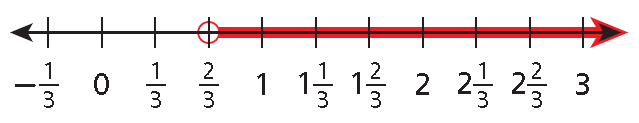
Example 5 :
1.5k < -0.3(k + 1) + 1.2
Solution :
1.5k < -0.3(k + 1) + 1.2
Distribute -0.3 on the right side of the inequality.
1.5k < -0.3k - 0.3 + 1.2
Combine the like terms on the right side.
1.5k < -0.3k + 0.9
Add 0.3k to each side.
1.8k < 0.9
Because k is multiplied by 1.8, divide each side by 1.8 to undo the multiplication.
k < 1/2
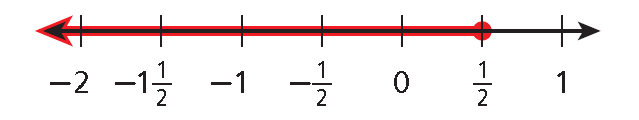
Some inequalities are true no matter what value is substituted for the variable. For these inequalities, all real numbers are solutions.
Some inequalities are false no matter what value is substituted for the variable. These inequalities have no solutions.
If both sides of an inequality are fully simplified and the same variable term appears on both sides, then the inequality has all real numbers as solutions or it has no solutions. Look at the other terms in the inequality to decide which is the case.
All Real Numbers as Solutions or No Solutions
Example 6 :
y + 4 ≥ y + 3
Solution :
y + 4 ≥ y + 3
The same variable term (y) appears on both sides. Look at the other terms.
For any number y, adding 4 will always result in a greater number than adding 3.
All values of x make the inequality true.
All real numbers are solutions.
Example 7 :
2(p + 3) < 5 + 2p
Solution :
2(p + 3) < 5 + 2p
Distribute 2 on the left side.
2p + 6 < 5 + 2p
The same variable term (2p) appears on both sides. Look at the other terms.
For any number 2p, adding 6 will never result in a lesser number than adding 5.
No values of x make the inequality true.
There are no solutions.
Business Application
Example 8 :
The Daily Info charges a fee of $675 plus $75 per week to run an ad. The People’s Paper charges $150 per week. For how many weeks will the total cost at Daily Info be less expensive than the cost at People’s Paper?
Solution :
Let x be the number of weeks the ad runs in the paper.
675 + 75x < 150x
Subtract 75x from each side.
675 < 75x
Because x is multiplied by 75, divide each side by 75 to undo the multiplication.
9 < x
x > 9
The total cost at Daily Info is less than the cost at People’s Paper, if the ad runs for more than 9 weeks.
Example 9 :
-5(2b + 7) + b < -b - 11
Solution :
-5(2b + 7) + b < -b - 11
Distributing -5,
-10b - 35 + b < -b - 11
-9b - 35 < -b - 11
Adding b on both sides
-9b + b - 35 < -11
-8b - 35 < - 11
Adding 35 on both sides.
-8b < -11 + 35
-8b < 24
Dividing by -8 on both sides
x > -3
Example 10 :
-3(-7p - 6) - 7 < p - 29
Solution :
-3(-7p - 6) - 7 < p - 29
Distributing -3, we get
21p + 18 - 7 < p - 29
21p + 11 < p - 29
Subtracting p on both sides
21p - p + 11 < -29
20p + 11 < - 29
Subtracting 11 on both sides
20p < -29 - 11
20p < -40
Dividing by 20 on both sides
p < -40/20
p < -2
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 150)
Apr 25, 25 11:46 AM
Digital SAT Math Problems and Solutions (Part - 150) -
AP Calculus AB Problems with Solutions (Part - 19)
Apr 24, 25 11:10 PM
AP Calculus AB Problems with Solutions (Part - 19) -
AP Calculus AB Problems with Solutions (Part - 18)
Apr 24, 25 11:06 PM
AP Calculus AB Problems with Solutions (Part - 18)
