SOLVING LINEAR EQUATIONS IN THREE VARIABLES USING RANK METHOD

• If there are n unknowns in the system of equations and ρ (A) = ρ ([A| B]) = n, then the system AX = B, is consistent and has a unique solution.
• If there are n unknowns in the system AX = B, and ρ (A) = ρ ([A| B]) = n − k, k ≠  0 then the system is consistent and has infinitely many solutions and these solutions form a k − parameter family. In particular, if there are 3 unknowns in a system of equations and ρ (A) = ρ ([A| B]) = 2, then the system has infinitely many solutions and these solutions form a one parameter family. 
• In the same manner, if there are 3 unknowns in a system of equations and ρ (A) = ρ ([A| B]) = 1, then the system has infinitely many solutions and these solutions form a two parameter family.
• If ρ(A) ≠ ρ ([A| B]), then the system AX = B is inconsistent and has no solution.

Question :

Test for consistency and if possible, solve the following systems of equations by rank method.

(iii) 2x + 2y + z = 5, x − y + z =1, 3x + y + 2z = 4

Solution :

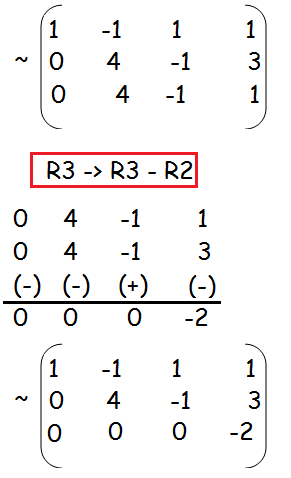
 ρ (A) = 3, ρ ([A| B]) = 2

Since the ranks of matrices A and [A, B} are not equal, it has no solution.

(iv) 2x − y + z = 2, 6x − 3y + 3z = 6, 4x − 2y + 2z = 4

Solution :

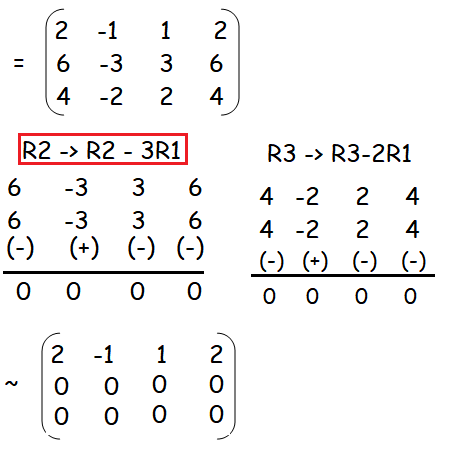
ρ (A) = 1, ρ ([A| B]) = 1

Since the ranks of matrices A and [A, B] are equal and it is less than 3, it has infinitely many solution.

2x - y + z  =  2

Let y = s and z = t

2x - s + t  =  2

2x  =  2 + s - t

x  =  (2 + s - t)/2

Hence the solution is ((2 + s - t)/2, s, t) where s,t belongs to R.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.