SOLVING RATIONAL INEQUALITIES WITH FRACTIONS ON BOTH SIDES

Example 1 :

Solve the following inequality

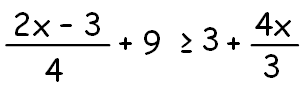
Solution :

First, let us take L.C.M on both sides

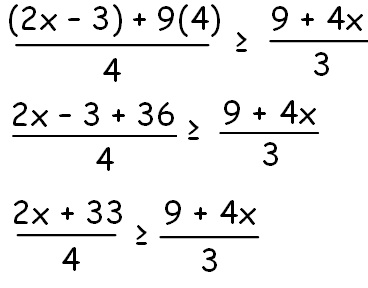
Multiply by 4 on both sides, we get

 2x + 33 ≥ (4/3)(9 + 4x)

Multiply by 3 on both sides, we get

3(2x + 33) ≥ 4(9 + 4x)

6x + 99 ≥ 36 + 16x

Subtract 16x on both sides

6x - 16x + 99 ≥ 36 + 16x - 16x

-10x + 99 ≥ 36

Subtract 99 on both sides

-10x + 99 - 99 ≥ 36 - 99

-10x ≥ -63

Divide by -10 on both sides

x ≤ 63/10

Since we divide by -10 throughout the equation, the sign has changed from ≥ to ≤.

So, the solution set of the given equation is (-∞, 63/10]

Example 2 :

Solve the following inequality

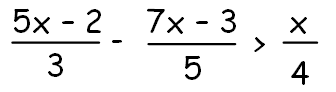
Solution :

First, let us take L.C.M on left side

[5(5x - 2) - 3(7x - 3)]/15 > (x/4)

(25x - 10 - 21x + 9) /15 > (x/4)

(4x - 1)/15 > (x/4)

Multiply by 4 throughout the equation

(4/15)(4x-1) > x

Multiply by 15 throughout the equation

4(4x - 1) > 15x 

16x - 4 > 15x 

Subtract 15 through out the equation

x - 4 > 0

Add 4 on both sides

x > 4

So, the solution is (4, ∞).

Example 3 :

Solve the following inequality

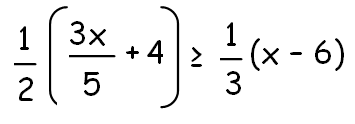
Solution :

First, let us take L.C.M on left side

(1/2)[(3x + 20)/5] ≥ (x - 6)/3

(3x + 20)/10 ≥ (x - 6)/3

Multiply by 10 on both sides

3x + 20 ≥ (10/3)(x - 6)

Multiply by 3 on both sides

3(3x + 20) ≥ 10(x - 6)

9x + 60 ≥ 10x - 60

Subtract 10 on both sides

9x - 10x + 60 ≥ 10x - 10x - 60

-x + 60 ≥ -60

Subtract 60 on both sides

-x ≥ -60 - 60

-x ≥ -120

Divide by -1 on both sides

x  ≤ 120

So, the solution is (-∞, 120].

Example 4 :

Solve the following inequality

(3/5)(x - 2) ≥ (5/3)(2 - x)

Solution :

Multiply by 3 on both sides

(9/5) (x - 2) ≥ 5(2 - x)

Multiply by 5 on both sides

9(x - 2) ≥ 25(2 - x)

9x - 18  ≥ 50 - 25x

Add 25x on both sides

9x + 25x - 18  ≥ 50

Add 18 on both sides

34x  ≥ 50 + 18

34x ≥ 68

Divide by 34 on both sides

x  ≥ 68/34  

x  ≥ 2

So, the solution set of the given function is [2, ∞). 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.