SOLVING RIGHT TRIANGLES WORKSHEET

Problem 1 :

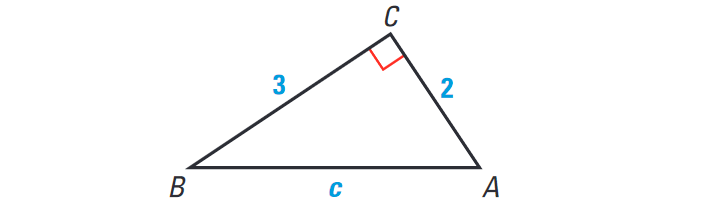
Solve the right triangle shown below and round decimals to the nearest tenth.

Problem 2 :

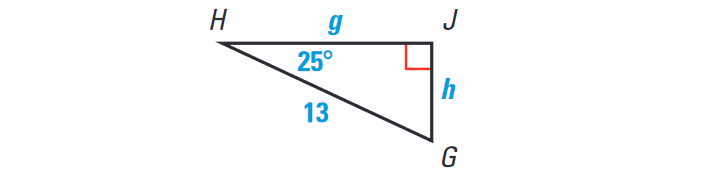
Solve the right triangle shown below and round decimals to the nearest tenth.

Problem 3 :

During a space shuttle's approach to earth, it’s glide angle changes.

(i) When the shuttle’s altitude is about 15.7 miles, its horizontal distance to the runway is about 59 miles. What is its glide angle ? Round the answer to the nearest tenth.

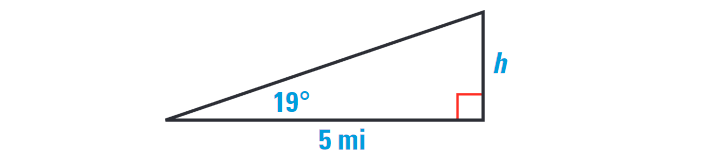
(ii)  When the space shuttle is 5 miles from the runway, its glide angle is about 19°. Find the shuttle’s altitude at this point in its descent. Round the answer to the nearest tenth.

Answers

1. Answer :

Begin by using the Pythagorean Theorem to find the length of the hypotenuse.

Pythagorean Theorem : 

(Hypotenuse)²  =  (Leg)² + (Leg)²

Substitute.

c²  =  2² + 3²

Simplify.

c²  =  4 + 9

c²  =  13

Take square root on each side. 

√c²  =  √13

c  =  √13

Use calculator to approximate.

c  ≈  3.6

Now, use a calculator to find the measure of ∠B :

Finally, because ∠A and ∠B are complements, we can write

m∠A  =  90° - ∠B  ≈  90° - 33.7°  =  56.3°

The side lengths of the triangle are 2, 3, and √13, or about 3.6. The triangle has one right angle and two acute angles whose measures are about 33.7° and 56.3°.

2. Answer :

Use trigonometric ratios to find the values of g and h.

Because ∠H and ∠G are complements, we can write

m∠G  =  90° - ∠H  =  90° - 25°  =  65°

The side lengths of the triangle are about 5.5, 11.8, and 13. The triangle has one right angle and two acute angles whose measures are 65° and 25°.

3. Answer :

(i) When the shuttle’s altitude is about 15.7 miles, its horizontal distance to the runway is about 59 miles. What is its glide angle ? Round the answer to the nearest tenth.

(ii)  When the space shuttle is 5 miles from the runway, its glide angle is about 19°. Find the shuttle’s altitude at this point in its descent. Round the answer to the nearest tenth.

Part (i) :

Sketch a right triangle to model the situation. Let x° be the measure of the shuttle’s glide angle.

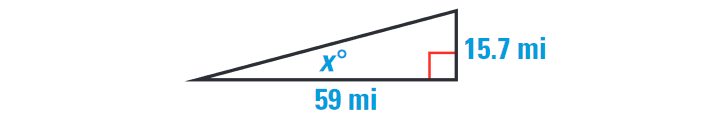
We can use the tangent ratio and a calculator to find the approximate value of x.

Write ratio.

tan x°  =  opp. / adj.

Substitute.

tan x°  =  15.7 / 59

x  =  tan^-1 (15.7 / 59)

Use calculator to find the value of tan^-1 (15.7 / 59).

x  ≈  14.9

When the space shuttle’s altitude is about 15.7 miles, the glide angle is about 14.9°.

Part (ii) :

Sketch a right triangle to model the situation. Let h be the altitude of the shuttle. We can use the tangent ratio and a calculator to find the approximate value of h. 

Write ratio.

tan x°  =  opp. / adj.

Substitute.

tan 19°  =  h / 5

Use a calculator.

0.3443  ≈  h / 5

Multiply each side by 5.

5 ⋅ 0.3443  ≈  h

1.7  ≈  h

The shuttle’s altitude is about 1.7 miles.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.