SOLVING SYSTEMS OF LINEAR EQUATIONS BY GRAPHING
We may know to solve system of linear equations using the methods like substitution or elimination.
In this section, we are going to see, how to solve the system of linear equations by graphing.
To solve the system of linear equations by graphing, we have to sketch the graph of each equation.
The point at which the graphs of the linear equations (straight lines) intersect is the solution of the given system of equations.
The x and y coordinates in the point of intersection are the values of x and y respectively.
For example, if(a, b) is the point of intersection, 'a' is the value of x and 'b' is the value of y.
That is.
x = a
y = b
Once we get the solution from the graph, we can check whether the ordered pair (a, b) is a solution of an equation in two variables by substituting the x- and y-values into the equation.
When we substitute the x- and y-values into the equation, if it results a true statement, then (x, y) is a solution of an equation.
Example 1 :
Solve the following system of equations by graphing.
x + y - 4 = 0
3x - y = 0
Solution :
Step 1 :
Let us re-write the given equations in slope-intercept form (y = mx + b).
y = - x + 4
(slope is -1 and y-intercept is 4)
y = 3x
(slope is 3 and y-intercept is 0)
Based on slope and y-intercept, we can graph the given equations.
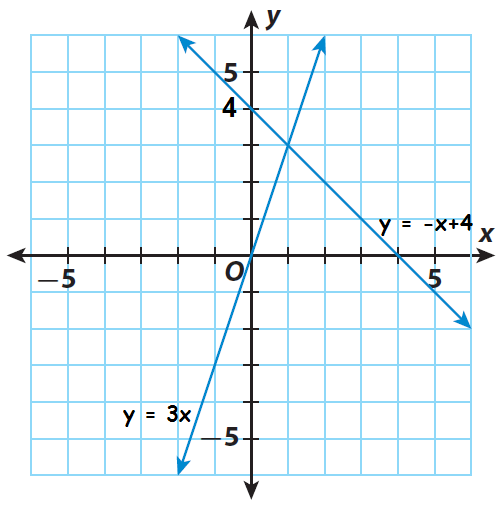
Step 2 :
Find the point of intersection of the two lines. It appears to be (1, 3). Substitute to check if it is a solution of both equations.
x + y - 4 = 0
1 + 3 - 4 = 0 ?
4 - 4 = 0 ?
0 = 0 True
3x - y = 0
3(1) - 3 = 0 ?
3 - 3 = 0 ?
0 = 0 True
Because the point (1, 3) satisfies both the equations, the solution of the system is (1, 3).
Example 2 :
Solve the following system of equations by graphing.
3x - y - 3 = 0
x - y - 3 = 0
Solution :
Step 1 :
Let us re-write the given equations in slope-intercept form.
y = 3x - 3
(slope is 3 and y-intercept is -3)
y = x - 3
(slope is 1 and y-intercept is -3)
Based on slope and y-intercept, we can graph the given equations.
Step 2 :
Find the point of intersection of the two lines. It appears to be (0, -3). Substitute to check if it is a solution of both equations.
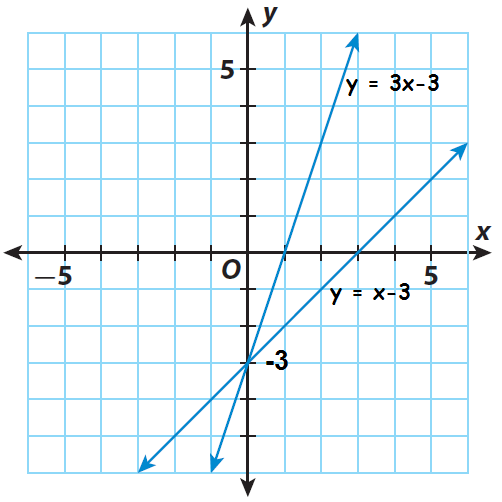
3x - y - 3 = 0
3(0) - (-3) - 3 = 0 ?
0 + 3 - 3 = 0 ?
0 = 0 True
x - y - 3 = 0
0 - (-3) - 3 = 0 ?
3 - 3 = 0 ?
0 = 0 True
Because the point (0, -3) satisfies both the equations, the solution of the system is (0, -3).
Example 3 :
During school vacation, Alex wants to go bowling and to play laser tag. He wants to play 6 total games but needs to figure out how many of each he can play if he spends exactly $20. Each game of bowling is $2 and each game of laser tag is $4.
Solution :
Step 1 :
Let "x" be the no. of games Alex bowls and "y" be the no. of laser tag he plays.
No. of games he bowls + No. of laser tags = Total games
x + y = 6 -------- (1)
Step 2 :
Write an equation which represents the money spent on these two items.
Cost of "x" no. of games he bowls = 2x
Cost of "y" no. of laser tags he plays = 4y
Total cost = $20
Then, we have
2x + 4y = 20
Divide both sides by 2.
x + 2y = 10 -------- (2)
Step 3 :
Write the equations (1) and (2) in slope-intercept form. Then graph.
(1) ----- > x + y = 6 ----- > y = -x + 6
(2) ---- > x + 2y = 10 --- > y = -(1/2)x + 5
Graph the equations y = -x + 6 and y = -(1/2)x + 5.
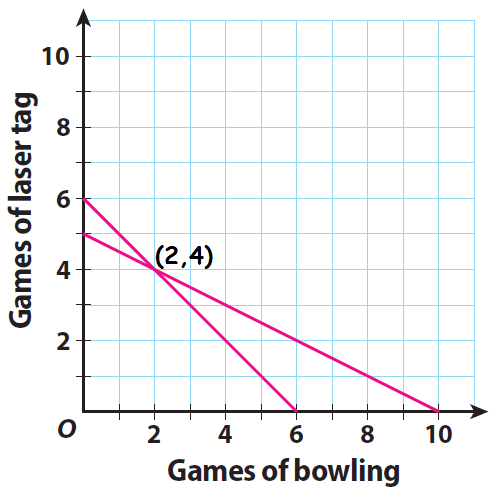
Step 4 :
Look at the graph and identify the solution of the system of equations. Check your answer by substituting the ordered pair into both equations.
The point of intersection of the lines is (2, 4).
Let us check whether the ordered pair (2, 4) satisfies both the equations.
y = -x + 6
4 = -2 + 6 ?
4 = 4 True
y = -(1/2)x + 5
4 = -(1/2)(2) + 5 ?
4 = -1 + 5 ?
4 = 4 True
Since the ordered pair (2, 4) satisfies both the equations, the solution of the system is (2, 4).
Step 5 :
Interpret the solution in the original context.
Alex will bowl 2 games and play 4 games of laser tag.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Derivative of Absolute Value of x Using Limit Definition
Apr 23, 25 11:11 AM
Derivative of Absolute Value of x Using Limit Definition -
Digital SAT Math Problems and Solutions (Part - 149)
Apr 23, 25 02:33 AM
Digital SAT Math Problems and Solutions (Part - 149) -
Digital SAT Math Problems and Solutions (Part - 148)
Apr 22, 25 08:20 AM
Digital SAT Math Problems and Solutions (Part - 148)

