SOLVING WORD PROBLEMS INVOLVING THE SHAPES SQUARES AND RECTANGLES

Problem 1 :

The side of a square exceeds the side of another square by 4 cm and the sum of the area of two squares is 400 sq.cm. Find the dimensions of the squares.

Solution :

Let x be side length of one square

The side of a square exceeds the side of another square by 4 cm

So, the side length of another square  =  x + 4

Area of one square with side length x  =  x²

Area of one square with side length x + 4 is (x + 4)²

Sum of the area of two squares  =  400 sq.cm

x² + (x + 4)²  =  400

x² + x² + 2 ⋅ x ⋅ 4 + 4²  =  400

2x² + 8x + 16 - 400  =  0

2x² + 8x - 384  =  0

By dividing the entire equation by 2, we get

x² + 4x - 192  =  0

(x + 16) (x - 12) = 0

Therefore sides of one square is 12 cm.

Side length of another square  =  (12 + 4)  =  16 cm.

Problem 2 :

The length of the rectangle exceeds its width by 2 cm and the area of the rectangle is 195 sq.cm. Find the dimensions of the rectangle.

Solution :

Let x and y be the width and length of rectangle respectively

The length of the rectangle exceeds its width by 2 cm

So, length (y)  =  x + 2

Area of the rectangle  =  195 sq.cm

Length ⋅ width = 195

x⋅(x + 2)  =  195

x² + 2x - 195  =  0

(x + 15) (x - 13)  =  0

Here x represents width of the rectangle. So, the negative value is not possible.

To find the value of y we have to apply the value of x in the equation y = x + 2

y  =  13 + 2

y  =  15 cm

Therefore length of rectangle is 15 cm and width of the rectangle is 13 cm.

Problem 3 :

The footpath of uniform width runs all around a rectangular field 28 meters long and 22 meters wide. If the path occupies 600 m² area, find the width of the path.

Solution :

Let x be the width of the path

Length of the rectangular field  =  28 m

Width of the rectangular field  =  22 m

Area of the path  =  600 m²

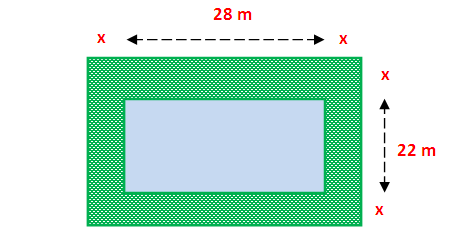
Length of the larger rectangle :

  =  28 + x + x

  =  28 + 2x

Width of the larger rectangle :

  =  22 + x + x

  =  22 + 2x

Area of the path  =  Area of larger rectangle - Area of smaller rectangle

600  =  (28 + 2x)  (22 + 2x) - 28 ⋅ 22

600  =  616 + 56x + 44x + 4x² - 616

600  =  56x + 44x + 4x²

600  =  4x² + 100 x

4x² + 100x  =  600

Dividing the entire equation by 4, we get

x² + 25x  =  150

x² + 25x - 150  =  0

(x - 5) (x + 30)  =  0

Negative value is not possible. Because x represents width of the path.

Therefore width of the path is 5 m

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.