SOLVING WORD PROBLEMS USING LINEAR EQUATIONS

Problem 1 : 

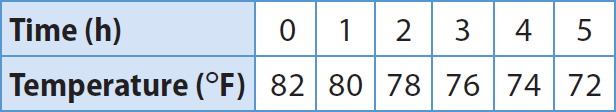
The table shows the temperature of a fish tank during an experiment. Write the appropriate linear equation for the given situation and use the equation to find temperature at the 7th hour.

Solution :

Step 1 : 

Notice that the change in the temperature is the same for each increase of 1 hour in time. So, the relationship is linear.

Since we want to find the temperature at the 7th hour, the appropriate linear equation for the given situation is slope-intercept form (y = mx + b), assuming y as temperature and x as hours.  

Step 2 : 

Choose any two points in the form (x, y), from the table to find the slope :

For example, let us choose (0, 82) and (1, 80).

Use the slope formula.

Substitute (x₁, y₁) = (0, 82) and (x₂, y₂) = (1, 80).

m = ⁽⁸⁰ ⁻ ⁸²⁾⁄₍₁ ₋ ₀₎

m = -²⁄₁

m = -2

Step 3 : 

Find the y-intercept using the slope and any point from the table.

Slope-intercept form equation of a line :

y = mx + b

Substitute m = -2, and (x, y) = (0, 82).

82 = -2(0) + b

82 = 0 + b

82 = b

Step 4 : 

Now, substitute m = -2 and b = 82 in slope-intercept form equation of a line.

y = mx + b

y = -2x + 82

Step 5 : 

Find the temperature at the 7th hour.

Substitute x = 7 in the equation y = -2x + 82. 

y = -2(7) + 82

y = -14 + 82

y = 68

So, the temperature at the 7th hour is 68⁰ F.

Problem 2 : 

Lily has just opened her new computer store. She makes $25 on every computer she sells and her monthly expenses are $10,000. What is the minimum number of computer does she need to sell in a month to make a profit ?

Solution :

Step 1 : 

Let y stand for the profit and x stand for number of computers sold. 

From the given information, we have 

Profit = 27(No. of computers sold) - Monthly expenses

y = 27x - 10,000

Step 2 : 

Let us find the number of computers sold for no profit. 

That is, find the value of x when y = 0. 

Substitute y = 0 in the equation y = 27x - 10,000. 

0 = 25x - 10,000

Add 10,000 to both sides.

10,000 = 25x

Divide both sides by 25. 

400 = x

Step 3 : 

When Lily sells 400 computers in a month, her profit is equal to zero.

So, she has to sell more than 400 computer per month to make a profit.

To make a profit, the minimum number of computers per month, she needs to sell is 401.

Problem 3 :

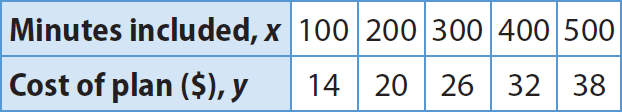
Elizabeth’s cell phone plan lets her choose how many minutes are included each month. The table shows the plan’s monthly cost y for a given number of included minutes x. Write an equation in slope-intercept form to represent the situation and use it to estimate cost of plan for 800 minutes included.

Solution :

Step 1 : 

Notice that the change in cost is the same for each increase of 100 minutes. So, the relationship is linear.

Step 2 : 

Choose any two points in the form (x, y), from the table to find the slope :

For example, let us choose (100, 14) and (200, 20).

Use the slope formula.

Substitute (x₁, y₁) = (100, 14) and (x₂, y₂) = (200, 20).

m = ⁽²⁰ ⁻ ¹⁴⁾⁄₍₂₀₀ ₋ ₁₀₀₎

m = ⁶⁄₁₀₀

m = 0.06

Step 3 : 

Find the y-intercept using the slope and any point from the table.

Slope-intercept form equation of a line :

y = mx + b

Substitute m = 0.06, and (x, y) = (100, 14)

14 = 0.06(100) + b

14 = 6 + b

8 = b

Step 4 : 

Now, substitute m = 0.06 and b = 8 in slope-intercept form equation of a line.

y = mx + b

y = 0.06x + 8

Step 5 : 

Estimate cost of plan for 800 minutes included.

Substitute x = 800 in the equation y = 0.06x + 8.

y = 0.06(800) + 8

y = 48 + 8

y = 56

So, the cost of plan for 800 minutes included is $56.

Problem 4 : 

The rent charged for space in an office building is a linear relationship related to the size of the space rented. At west main street office rentals, $750 rent charged for 600 square feet of space and $1150 rent charged for 900 square feet of space. Write an equation in slope-intercept form for the rent at West Main Street Office Rentals and use it to calculate the rent for 1200 square feet of space.

Solution :

Step 1 :

Identify the independent and dependent variables.

The independent variable (x) is the square footage of floor space.

The dependent variable (y) is the monthly rent.

Step 2 :

Write the information given in the problem as ordered pairs.

The rent for 600 square feet of floor space is $750 :

(600, 750)

The rent for 900 square feet of floor space is $1150 :

(900, 1150)

Step 3 : 

Find the slope.

Substitute (x₁, y₁) = (600, 750) and (x₂, y₂) = (900, 1150).

m = ⁽¹¹⁵⁰ ⁻ ⁷⁵⁰⁾⁄₍₉₀₀ ₋ ₆₀₀₎

m = ⁴⁰⁰⁄₃₀₀

m = ⁴⁄₃

Step 4 : 

Find the y-intercept.

Use the slope ⁴⁄₃ and one of the ordered pairs (600, 750).

Slope-intercept form :

y = mx + b

Substitute m = ⁴⁄₃, x = 600 and y = 750.

750 = (⁴⁄₃)(600) + b

750 = 4(200) + b

750 = 800 + b

-50 = b

Step 5 : 

Substitute the slope and y-intercept.

Slope-intercept form

y = mx + b 

Substitute m = ⁴⁄₃ and b = -50

y = (⁴⁄₃)x + (-50)

y = (⁴⁄₃)x - 50 

Step 6 : 

Calculate the rent for 1200 square feet of space.

Substitute x = 1200 in the equation y = (⁴⁄₃)x - 50.

y = (⁴⁄₃)(1200) - 50

y = 4(400) - 50

y = 1600 - 50

y = 1550

So, the rent for 1200 square feet of space is $1550.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.