SUM AND PRODUCT OF THE ROOTS OF A QUADRATIC EQUATION EXAMPLES

If a quadratic equation is given in standard form, we can find the sum and product of the roots using coefficient of x², x and constant term. 

Let us consider the standard form of a quadratic equation,  

ax² + bx + c = 0

(Here a, b and c are real and rational numbers)

Let α and β be the two roots or zeros of the above quadratic equation. 

Then the formula to get sum and product of the roots or zeros of a quadratic equation is,

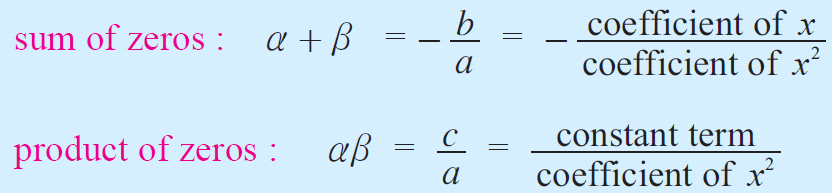
Find the sum and product of roots of the following quadratic equations.

Example 1 :

x² - 5x + 6 = 0

Solution :

Comparing

x² - 5x + 6 = 0

and 

ax² + bx + c = 0

we get

a = 1, b = -5 and c = 6

Therefore, 

sum of the roots  = -b/a

= -(-5)/1

= 5

product of the roots = c/a

= 6/1

= 6

Example 2 :

x² - 6 = 0

Solution :

Comparing

x² - 6 = 0

and 

ax² + bx + c = 0

we get

a = 1, b = 0 and c = -6

Therefore, 

sum of the roots = -b/a

= 0/1 = 0

product of the roots = c/a

= -6/1

= -6

Example 3 :

3x² + x + 1  =  0

Solution :

Comparing

3x² + x + 1 = 0

and 

ax² + bx + c = 0

we get

a = 3, b = 1 and c = 1

Therefore, 

sum of the roots = -b/a

= -1/3

product of the roots = c/a

= 1/3

Example 4 :

3x² + 7x = 2x - 5

Solution :

First write the given quadratic equation in standard form.

3x² + 7x = 2x - 5

Subtract 2x from both sides.

3x² + 5x = -5

Add 5 to both sides.

3x² + 5x + 5 = 0

Comparing

3x² + 5x + 5 = 0

and 

ax² + bx + c = 0

we get

a = 3, b = 5 and c = 5

Therefore, 

sum of the roots = -b/a

= -5/3

product of the roots = c/a

= 5/3

Example 5 :

3x² -7x + 6 = 6

Solution :

First write the given quadratic equation in standard form.

3x² -7x + 6 = 6

Subtract 6 from both sides.

3x² - 7x = 0

Comparing

3x² - 7x = 0

and

ax² + bx + c = 0

we get

a = 3, b = -7 and c = 0

Therefore, 

sum of the roots = -b/a

= -(-7)/3

= 7/3

product of the roots = c/a

= 0/3

= 0

Example 6 :

x² + 5x + 1 = 3x² + 6

Solution :

First write the given quadratic equation in standard form.

x² + 5x + 1 = 3x² + 6

Subtract 3x² from both sides.

-2x² + 5x + 1 = 6

Subtract 6 from both sides.

-2x² + 5x - 5 = 0

Multiply both sides by -1.

2x² - 5x + 5 = 0

Comparing

2x² - 5x + 5 = 0

and 

ax² + bx + c = 0

we get

a = 2, b = -5 and c = 5

Therefore, 

sum of the roots = -b/a

= -(-5)/2

= 5/2

product of the roots = c/a

= 5/2

Example 7 :

2x² + 8x - m³ = 0 

Solution :

Comparing

2x² + 8x - m³ = 0

and 

ax² + bx + c = 0

we get

a = 2, b = 8 and c = -m³

Given : Product of the roots is 4.

c/a = 4

-m³/2 = 4

Multiply both sides by (-2). 

m³ = -8

m³ = (-2)³

m = -2

Example 8 :

x² - (p + 4)x + 5 = 0

Solution :

Comparing

x² - (p + 4)x + 5 = 0

and 

ax² + bx + c = 0

we get

a = 1, b = -(p + 4) and c = 5

Given : Sum of the roots is 0.

-b/a = 0

-[-(p + 4)] = 0

p + 4 = 0

p = -4

Example 9 :

x² + (2p - 1)x + p² = 0

Solution :

Comparing

x² + (2p - 1)x + p² = 0

and 

ax² + bx + c = 0

we get

a = 1, b = (2p - 1) and c = p²

Given : Product of the roots is 1.

c/a = 1

p²/1 = 1

p² = 1

Take square root on both sides. 

√p² = √1

 p = ±1

Example 10 :

1/(x + 1) + 2/(x - 4) = 2

Solution :

1/(x + 1) + 2/(x - 4) = 2

[1(x - 4) + 2(x + 1)] / [(x + 1)(x - 4)] = 2

1(x - 4) + 2(x + 1) = 2(x + 1)(x - 4)

x - 4 + 2x + 2 = 2(x² - 4x + x - 4)

3x - 2 = 2(x² - 3x - 4)

3x - 2 = 2x² - 6x - 8

0 = 2x² - 9x - 6

2x² - 9x - 6 = 0

Comparing

2x² - 9x - 6 = 0

and 

ax² + bx + c = 0

we get

a = 2, b = -9 and c = -6

Therefore, 

sum of the roots = -b/a

= -(-9)/2

= 9/2

product of the roots = c/a

= -6/2

= -3

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.