SYSTEM OF LINEAR EQUATIONS IN TWO VARIABLES

A linear system of two equations with two variables is any system that can be written in the form.

a₁x + b₁y + c₁ = 0

a₂x + b₂y + c₂ = 0

A solution to a system of equations is a value of x and and value of y, when substituted into the equations, satisfies both equations at the same time.

For the example,

3x - y = 7 ----(1)

2x + 3y = 1 ----(2)

3(1) + (2)

9x - 3y + 2x + 3y = 21 + 2

11x = 22

x = 2

By applying the value of x in (1), we get

3(2) - y = 7

6 - y = 7

y = -1

x = 2 and y = −1 is a solution to the system.

Checking :

3x - y = 7 ----(1)

2x + 3y = 1 ----(2)

Example Problem of Solving System of Linear Equations By Graphing Method

Example 1 :

x + y = 7

x - y = 3

Solution :

x + y = 7 ----(1)

x - y = 3 ----(2)

From (1),

y = 7 - x

Substitute some random values for x and solve for y. 

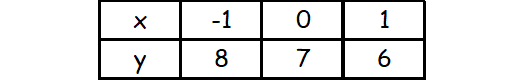
The points on the first line are

(-1, 8), (0, 7), (1, 6)

From (2),

y = x - 3

Substitute some random values for x and solve for y. 

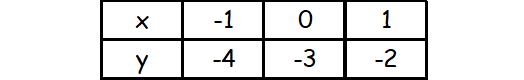
The points on the second line are

(-1, -4), (0, -3), (1, -2)

Plot the points from the above tables and sketch the graph

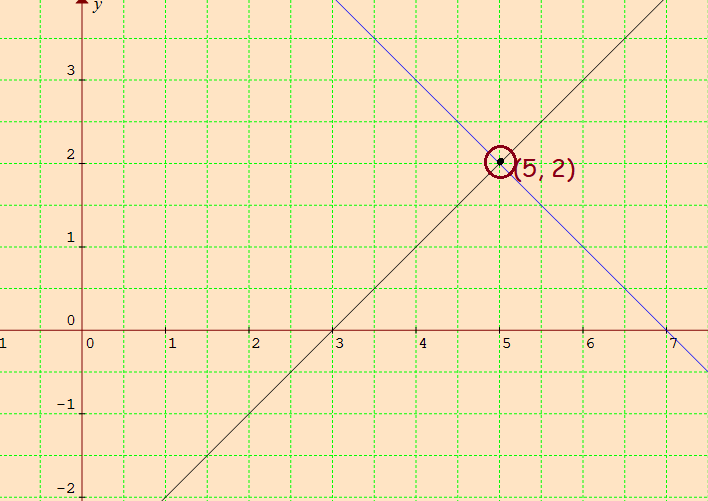
The point of intersection of the two lines is the solution.

So, the solution is (x, y) = (5, 2).

Example Problem of Solving System of Linear Equations By Substitution Method

Example 2 :

Solve the following system of equations by substitution method.

2x - 3y = -1

y = x - 1

Solution :

2x - 3y = -1 ----(1) 

y = x - 1 ----(2) 

Step 1 :

From (2), substitute (x - 1) for y into (1).

(1)----> 2x - 3(x - 1) = -1

Simplify.

2x - 3x + 3 = -1

-x - 3 = -1

Add 3 to each side. 

-x = 2

Multiply each side (-1). 

x = -2

Step 2 : 

Substitute -2 for x into (2). 

(2)----> y = 2 - 1

 y = 1

Therefore, the solution is 

(x, y) = (-2, 1)

Example Problem of Solving System of Linear Equations By Elimination Method

Example 3 :

Solve the following system of linear equations by elimination method.

x + 2y = 7

x – 2y = 1 

Solution :

x + 2y = 7 ----(1)

x – 2y = 1 ----(2)

The coefficients of x and y are equal in both the equations.

(1) + (2) :

2x = 8

x = 8/2

x = 4

By substituting x = 4 in (1), we get

4 + 2y = 7

2y = 7 – 4

2y = 3

y = 3/2

Hence the solution is (4, 3/2).

Example Problem of Solving System of Linear Equations By Cross Multiplication Method

Example 4 :

Solve the following system of linear equations by cross multiplication method.

3x + 4y = 24

20x - 11y = 47

Solution :

3x + 4y - 24 = 0 ----(1)

20x - 11y - 47 = 0 ----(2)

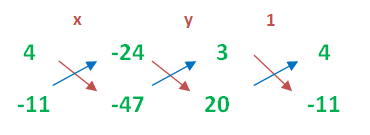
x/(-188 - 264) = y/(-480 -(-141)) = 1/(-33 - 80)

x/(-452) = y/(-480 + 141)) = 1/(-33 - 80)

x/(-452) = y/(-339) = 1/(-113)

So, the solution is (4, 3).

Finding Unknown When Solution of Linear Equation is Given

Example 5 :

If the point (4,-2) lies on the line 5x + 2y + k = 0.

Find the value of k.

Solution :

Here the given point (4,-2) lies on the given line.

To solve this problem we have to apply the given points in the equation as the value of x and y.

Here x = 4 and y = -2

The given equation is 5x + 2y + k = 0.

By applying the values of x and y, we get

5(4) + 2(-2) + k = 0

20 - 4 + k = 0

16 + k = 0

k = -16

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.