SYSTEMS OF LINEAR INEQUALITIES WORKSHEET

Problem 1 : 

Tell whether the ordered pair (2, 1) is a solution of the following system.

y < -x + 4

y ≤ x + 1

Problem 2 : 

Tell whether the ordered pair (2, 0) is a solution of the following system.

y ≥ 2x

y < x + 1

Problem 3 : 

Graph the following system of linear inequalities. Give two ordered pairs that are solutions and two that are not solutions.

8x + 4y ≤ 12

y > (1/2)x - 2

Problem 4 : 

y < 2 x - 3

y > 2x + 2

Problem 5 : 

y > x - 3

y ≤ x + 1

Problem 6 : 

y ≤ - 3x - 2

y ≤ -3x + 4

Problem 7 : 

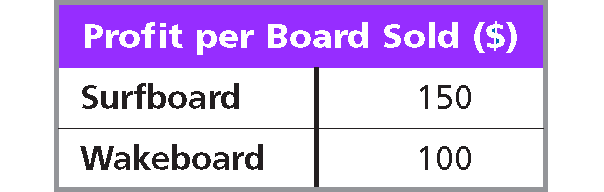
A surf shop makes the profits given in the table. The shop owner sells at least 10 surfboards and at least 20 wakeboards per month. He wants to earn at least $2000 a month. Show and describe all possible combinations of surfboards and wakeboards that the store owner needs to sell to meet his goals. List two possible combinations.

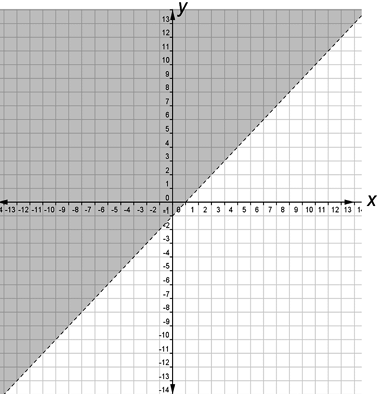
Problem 8 :

What linear inequality is represented by the graph at the right?

a. x – y > 1        b. x – y < 1     c. -x + y > 1     d. -x + y < 1

Problem 9 :

In the inequality c – 4d ≤ 10, what could be the possible value of d if c = 8?

a)  d ≤ -1/2    b)  d ≥ -1/2      c)  d ≤ 1/2    d)  d ≥ 1/2

Detailed Answer Key

1. Answer :

(2, 1) is a solution to the system, because it satisfies both inequalities.

2. Answer : 

(2, 0) is not a solution to the system, because it does not satisfy both inequalities.

3. Answer : 

Solve the first inequality for y.

8x + 4y ≤ 12

4y ≤ 12 - 8x

y ≤ 3 - 2x

Graph the system.

y ≤ 3 - 2x

y > (1/2)x - 2

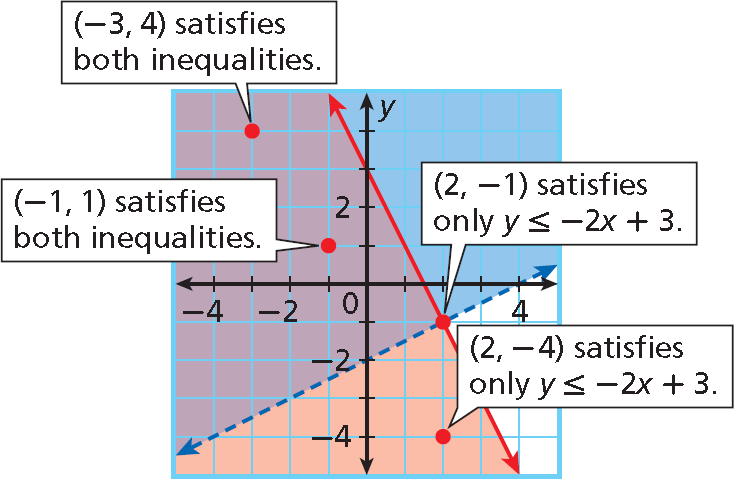
(-1, 1) and (-3, 4) are solutions.

(2, -1) and (2, -4) are not solutions.

4. Answer : 

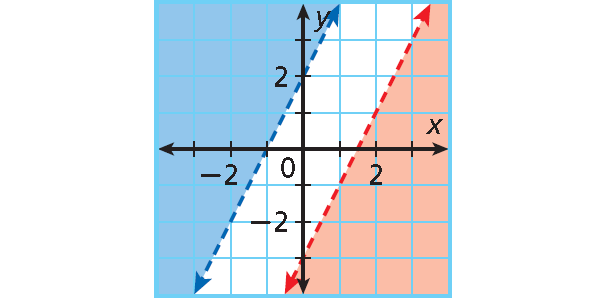
This system has no solution.

5. Answer :

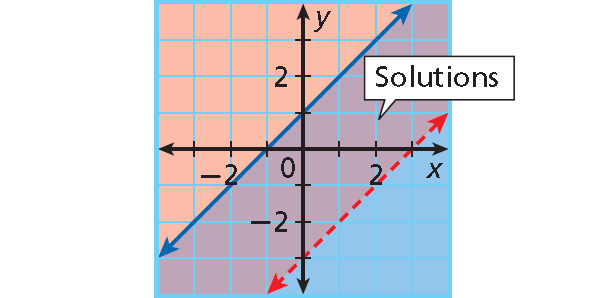
The solutions are all points between the parallel lines and on the solid line.

6. Answer :

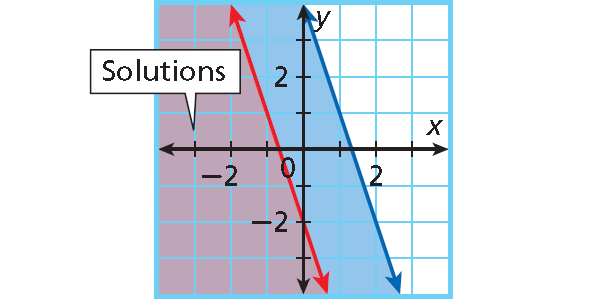
The solutions are the same as the solutions of y ≤ -3x - 2.

7. Answer : 

Step 1 : 

Write a system of inequalities. Let x represent the number of surfboards and y represent the number of wakeboards.

x ≥ 10 ---> He sells at least 10 surfboards.

y ≥ 20 ---> He sells at least 20 wakeboards.

He wants to earn a total of at least $2000.

150x + 100y ≥ 2000

Step 2 : 

Graph the system.

The graph should be in only the first quadrant because sales are not negative.

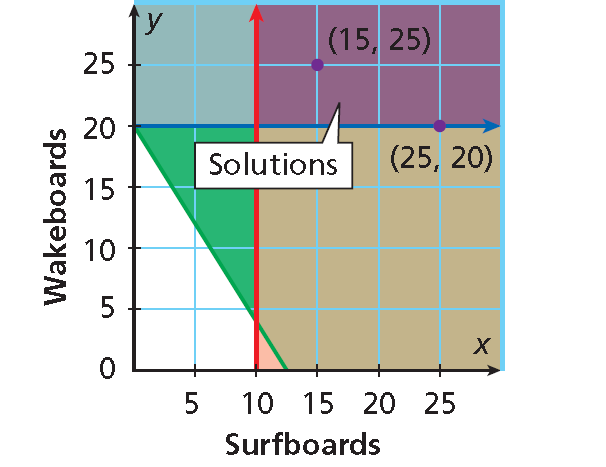
Step 3 : 

Describe all possible combinations. To meet the sales goals, the shop could sell any combination represented by an ordered pair of whole numbers in the solution region. Answers must be whole numbers because the shop cannot sell part of a surfboard or wakeboard. 

Step 4 : 

List two possible combinations.

Two possible combinations are :

15 surfboards and 25 wakeboards

25 surfboards and 20 wakeboards

8 Answer :

a. x – y > 1       b. x – y < 1         c. -x + y > 1      d. -x + y < 1

Finding slope of the line, (2, 1) and (3, 2)

Slope = (2 - 1) / (3 - 2)

= 1/1

= 1

Since it is raising line, the line has positive slope. y-intercept is -1.

y = mx + b

y = 1x + (-1)

y = x - 1

Applying one of the points from the shaded region, we get

(1, 3)

3 = 1 - 1

3 = 0 

To make the above statement true, we have to use the sign >.

y > x - 1

-x + y > -1

Multiplying by negative on both sides, we get

x - y < 1

So, option b is correct.

9 Answer :

c – 4d ≤ 10, when c = 8

8 - 4d ≤ 10

Subtracting 8 on both sides

-4d ≤ 10 - 8

-4d ≤ 2

Dividing by -4 on both sides,

d ≥ 1/2

So, option d is correct.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.