TANGENTS TO CIRCLES

A tangent is a line in the plane of a circle that intersects the circle in exactly one point. Line k in the diagram above is a tangent.

Point of Tangency :

The point where a tangent line touches the circle. Point m in the diagram above is the point of tangency.

Common Tangent :

A line or segment that is tangent to two coplanar circles is called a common tangent.

Theorems 

Theorem 1 :

If a line is tangent to circle, then it is perpendicular to the radius drawn to the point of tangency. 

In the diagram shown below, if l is tangent to circle Q at P, then l ⊥ QP.

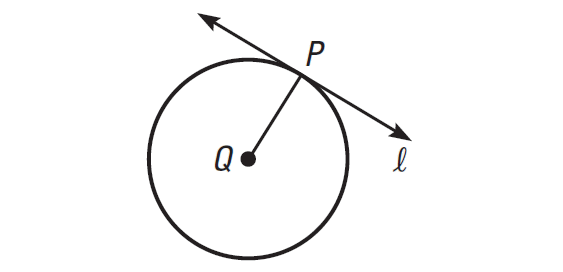
Theorem 2 :

If a plane, if a line is perpendicular to a radius of a circle at its endpoint on the circle, then the line is tangent to the circle.  

In the diagram shown below, if l ⊥ QP at P, then  l is tangent to circle Q. 

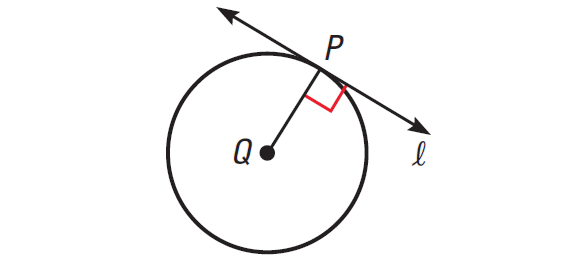
From a point in a circle's exterior, we can draw exactly two different tangents to the circle. The following theorem tells us the segments joining the external point to the two points of tangency are congruent. 

Theorem 3 :

If two segments from the same exterior points are tangent to a circle, then they are congruent. 

In the diagram shown below, if SR and ST are tangent to circle P, then SR  ≅  ST.

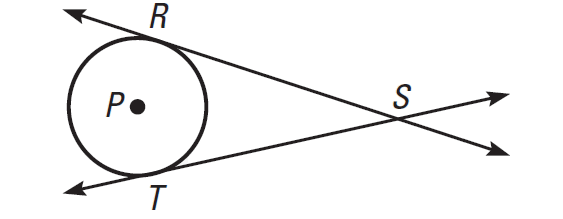
Identifying Tangent to a Circle

Example 1 :

Tell which line or segment is best described as a tangent in the diagram shown below.   

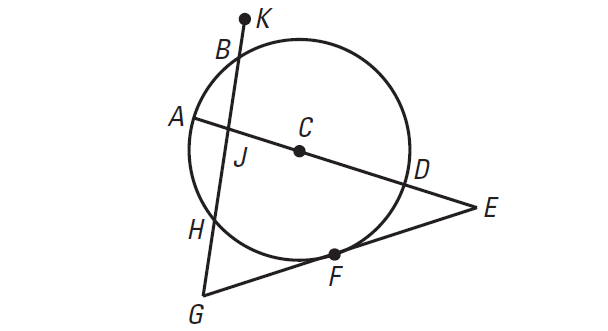
Solution :

EG is a tangent, because it intersects the circle in one point. 

Identifying Common Tangents 

Example 2 :

In the diagram shown below, tell whether the common tangents are internal or external.  

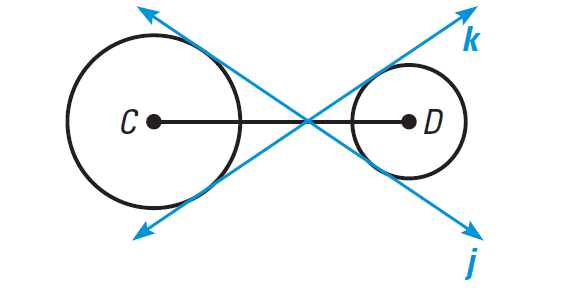
Solution :

The lines j and k intersect CD, they are common internal tangents. 

Example 3 :

In the diagram shown below, tell whether the common tangents are internal or external.  

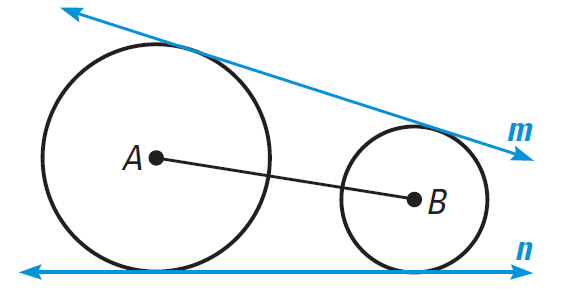
Solution :

The lines m and n do not intersect AB, so they are common external tangents. 

Tangent in Coordinate Geometry

Example 4 :

In the diagram shown below, describe all common tangent and identify the point of tangency. 

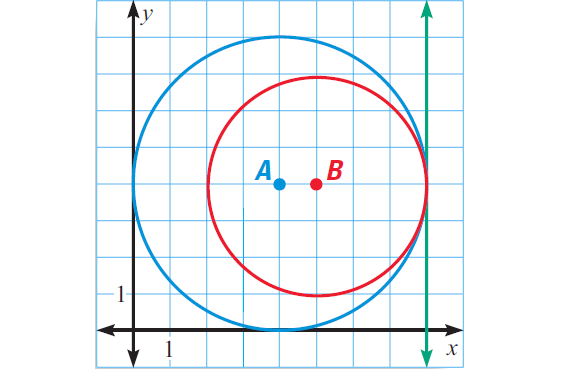
Solution :

The vertical line x  =  8 is the only common tangent of the two circles. 

The point of tangency is (8, 4). 

Note :

The point at which a tangent line intersects the circle to which it is tangent is the point of tangency. 

Verifying a Tangent to a Circle

Example 5 :

In the diagram shown below, say whether EF is tangent to the circle with center at D.  

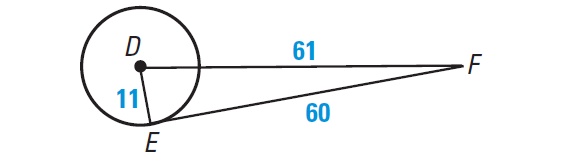
Solution :

We can use the Converse of the Pythagorean Theorem to say whether EF is tangent to circle with center at D.  

Because 112 + 602  =  612, ΔDEF is a right triangle and DE is perpendicular to EF.

So by Theorem 2 given above, EF is tangent to the circle with center at D.  

Finding the Radius of a Circle

Example 6 :

In the diagram shown below, I am standing at C, 8 feet from a grain silo. The distance from me to a point of tangency is 16 feet. What is the radius of the silo ?    

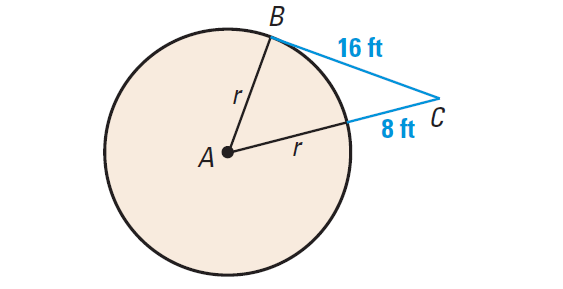
Solution :

By the Theorem 1 given above,  tangent BC is perpendicular to radius AB at B. So ΔABC is a right triangle. So we can use the Pythagorean theorem.  

Pythagorean Theorem :

(r + 8)²  =  r² + 16²

Square of binomial :

r² + 16r + 64  =  r² + 256

Subtract r² from each side : 

16r + 64  =  256

Subtract 64 from each side : 

16r  =  192

Divide each side by 16. 

r  =  12

Hence, the radius of the silo is 12 feet. 

Using Properties of Tangents

Example 7 :

In the diagram shown below, 

AB is tangent at B to the circle with center at C

AD is tangent at D to the circle with center at C

Find the value of x. 

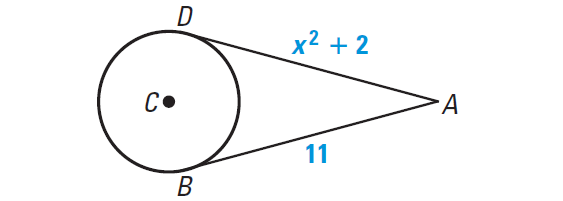
Solution :

By the Theorem 3 given above, two tangent segments from the same point are congruent.

AB  =  AD

Substitute : 

x² + 2  =  11

Subtract 2 from each side. 

x²  =  9

Take square root on each side. 

x  =  ± 3

Hence, the value of x is 3 or -3.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.