TERMS FACTORS AND COEFFICIENTS

A single variable or a constant or a combination of these as a product or quotient forms a term.

Examples :

5, -a, 3ab, 21/7, ........... etc

Terms can be added or subtracted to form an expression. In the expression 2x + 3 the term 2x is made of 2 factors and 2 and x while 3 is a single factor.

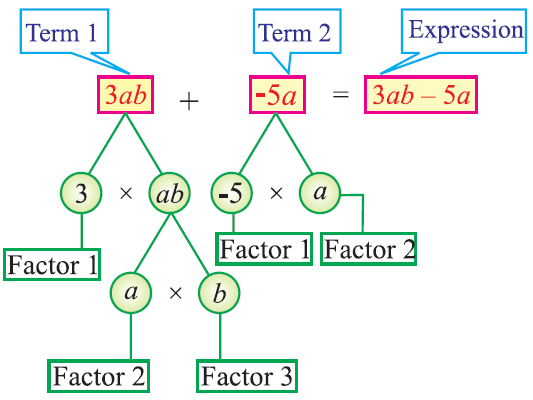
Consider the expression 3ab – 5a. It has two terms 3ab and -5a. The term 3ab is a product of factors 3, a and b. The term -5a is a product of -5 and a. The coefficient of a variable is a factor or factors.

Examples :

In the term 3ab;

(i) the coefficient of ab is 3 (ii) the coefficient of a is 3b

(iii) the coefficient of b is 3a.

In the term –5a the coefficient of a is –5

Example 1 :

Identify the number of terms and coefficient of each term in the expression.

x² y² -  5 x² y + (3/5) xy² - 11

Solution :

In the given expression, we have four terms.

Term 1  ==>  x² y²

Term 2  ==>  -  5 x² y

Term 3  ==>  (3/5) xy²

Term 4  ==>  -11

Coefficient of 1st term  =  1

Coefficient of 2nd term  =  -5

Coefficient of 3rd term  =  3/5

Since the last term is not having any variable, it is a constant term.

Example 2 :

Identify the number of terms, coefficient and factors of each term in the expression.

3abc - 5ca

Solution :

The given expression contains two terms.

Term 1  ==> 3abc

Term 2 ==>  -5ca

Example 3 :

Identify the number of terms, coefficient and factors of each term in the expression.

1 + x + y²

Solution :

The given expression contains three terms.

Term 1  ==> 1

Term 2 ==>  x

Term 2 ==>  y²

Example 4 :

Identify the number of terms, coefficient and factors of each term in the expression.

3x² y² - 3xyz + z³ 

Solution :

The given expression contains three terms.

Term 1  ==> 3x² y²

Term 2 ==>   - 3xyz 

Term 2 ==>  z³ 

Example 5 :

The coefficient of x⁴ in -5x⁷ + (3/7) x⁴ - 3x³ + 7x² - 1  

Solution :

The coefficient of x⁴ is 3/7.

Example 6 :

If A = 4x² + 7x - 1 and B = -x² - 5x + 3, then what is (3/2)A - 2B?

Solution :

A = 4x² + 7x - 1 and B = -x² - 5x + 3

To find (3/2)A, we have to multiply each coefficient of polynomial A by 3/2.

= (3/2) [4x² + 7x - 1]

= 6x² + (21/2)x - (3/2) ------(1)

To find 2B, we have to multiply each coefficient of polynomial by 2.

= 2(-x² - 5x + 3)

= -2x² + 10x + 6 -------(2)

(1) - (2)

= 6x² + (21/2)x - (3/2) - (-2x² + 10x + 6)

= 6x² + (21/2)x - (3/2) + 2x² - 10x - 6

= 8x² + (21/2)x - 10x - (3/2) - 6

= 8x² + (21 - 20/2)x + (-3 - 12)/2

= 8x² + (1/2)x - (15/2)

Example 6 :

(2x² + 3x - 4)(3x + 2) = 6x³ + ax² - 6x - 8

In the given equation, a is a constant. If the equation is true for all values of x, what is the value of a ?

a)  4     b)  9      c)  13     d)  16

Solution :

(2x² + 3x - 4)(3x + 2) = 6x³ + ax² - 6x - 8

To solve for a, we have to equate the coefficients of like terms.

(2x² + 3x - 4)(3x + 2) = 6x³ + 9x - 12x + 4x² + 6x - 8

= 6x³ + 4x² + 15x - 12x - 8

= 6x³ + 4x² + 3x - 8

6x³ + 4x² + 3x - 8 = 6x³ + ax² - 6x - 8

By comparing the coefficient of x², we get a = 4

Option a is correct.

Example 7 :

The equation

(36x² + 16x - 21)/(tx - 4) = (-9x + 5) - [1/(tx - 4)]

is true for all values of x for which x ≠ 4/t, where t is a constant. What is the value of t ?

a)  -20     b)  -4      c)  4     d)  12

Solution :

(36x² + 16x - 21)/(tx - 4)

Dividing this by long division is not possible, so by observing the given possible values in the options, let us consider option b.

t = -4

= (-9x + 5) + [1/(tx - 4)]

Applying t = -4, we get

= (-9x + 5) - [1/(-4x - 4)]

= [(-9x + 5)(-4x - 4) - 1]/(-4x - 4)

= 36x² - 20x + 36x - 20 - 1

= 36x² + 16x - 21

Since we get the same terms that we have in the left side, the required value of t is -4, which is option b.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.