THE CONVERSE OF THE PYTHAGOREAN THEOREM

The Pythagorean Theorem states that if a triangle is a right triangle, then the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the legs. The Converse of the Pythagorean Theorem is also true as stated below.

The Converse of the Pythagorean Theorem

If the square of the length of the longest side of a triangle is equal to the sum of the squares of the lengths of the other two sides, then the triangle is a right triangle.

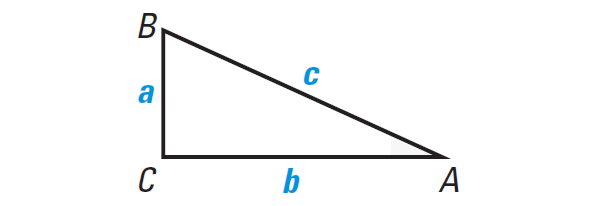
If c²  =  a² + b², then ΔABC is a right triangle.

We can use the Converse of the Pythagorean Theorem to verify that a given triangle is a right triangle.

Verifying Right Triangles

Example 1 :

The triangle shown below appears to be a right triangle. Say whether it is right a triangle.

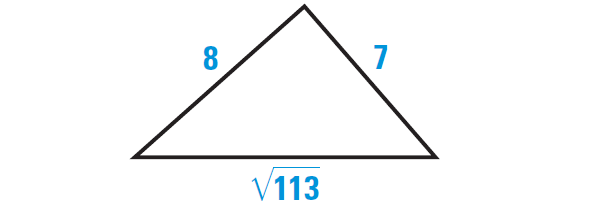
Solution :

Let c represent the length of the longest side of the triangle. Check to see whether the side lengths satisfy the equation

c²  =  a² + b²

Substitute.

 (√113)²  =  7² + 8²  ?

 113  =  49 + 64  ?

113  =  113

Because the lengths of the sides of the triangle satisfy the equation c²  =  a² + b², the triangle shown above is a right triangle. 

Example 2 :

The triangle shown below appears to be a right triangle. Say whether it is right a triangle.

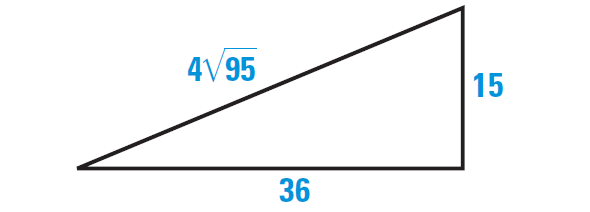
Solution :

Let c represent the length of the longest side of the triangle. Check to see whether the side lengths satisfy the equation

c²  =  a² + b²

Substitute.

 (4√95)²  =  15² + 36²  ?

 4² ⋅ (√95)²  =  15² + 36²  ?

16 ⋅ 95  =  225 + 1296  ?

 1520  ≠  1521

Because the lengths of the sides of the triangle do not satisfy the equation c²  =  a² + b², the triangle shown above is not a right triangle. 

Classifying Triangles

Sometimes it is difficult to say from looking whether a triangle is obtuse or acute or right. The theorems given below can help us to decide.

Theorems on Classifying Triangles

Theorem 1 :

If the square of the length of the longest side of a triangle is less than the sum of the squares of the lengths of the other two sides, then the triangle is acute.

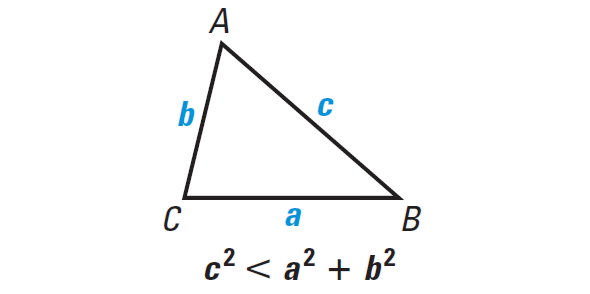
If c²  <  a² + b², then ΔABC is acute. 

Theorem 2 :

If the square of the length of the longest side of a triangle is greater than the sum of the squares of the lengths of the other two sides, then the triangle is obtuse.

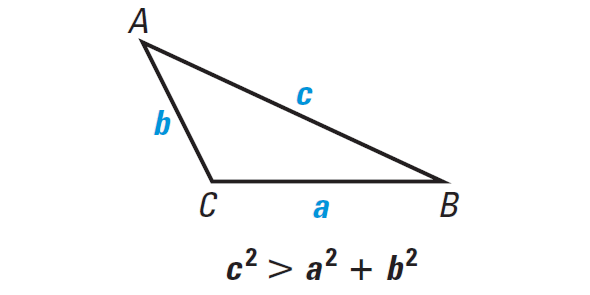
If c²  >  a² + b², then ΔABC is obtuse. 

Theorem 3 :

If the square of the length of the longest side of a triangle is equal to sum of the squares of the lengths of the other two sides, then the triangle is right.

If c²  =  a² + b², then ΔABC is right.

Solved Examples

Example 1 : 

Decide whether the set of numbers given below can represent the side lengths of a triangle. If they can, classify the triangle as right, acute, or obtuse. 

38, 77, 86

Solution :

By Triangle Inequality Theorem, the above set of numbers can represent the side lengths of a triangle.

Compare the square of the length of the longest side with the sum of the squares of the lengths of the two shorter sides.

Compare c² with a² + b².

c²  ?  a² + b²

Substitute.

86²  ?  38² + 77²

7396  ?  1444 + 5929

7396  >  1444 + 5929

c²  >  a² + b²

Because c² is greater than a² + b², the triangle is obtuse.

Example 2 : 

Decide whether the set of numbers given below can represent the side lengths of a triangle. If they can, classify the triangle as right, acute, or obtuse. 

10, 36, 37

Solution :

By Triangle Inequality Theorem, the above set of numbers can represent the side lengths of a triangle.

Compare the square of the length of the longest side with the sum of the squares of the lengths of the two shorter sides.

Compare c² with a² + b².

c²  ?  a² + b²

Substitute.

37²  ?  10² + 36²

1369  ?  100 + 1296

1369  <  1396

c²  <  a² + b²

Because c² is less than a² + b², the triangle is acute.

Example 3 : 

Decide whether the set of numbers given below can represent the side lengths of a triangle. If they can, classify the triangle as right, acute, or obtuse. 

30, 40, 50

Solution :

By Triangle Inequality Theorem, the above set of numbers can represent the side lengths of a triangle.

Compare the square of the length of the longest side with the sum of the squares of the lengths of the two shorter sides.

Compare c² with a² + b².

c²  ?  a² + b²

Substitute.

50²  ?  30² + 40²

2500  ?  900 + 1600

2500  =  2500

c²  =  a² + b²

Because c² is equal to a² + b², the triangle is right.

Building a Foundation

Example 4 :

We use four stakes and string to mark the foundation of a house. We want to make sure the foundation is rectangular.

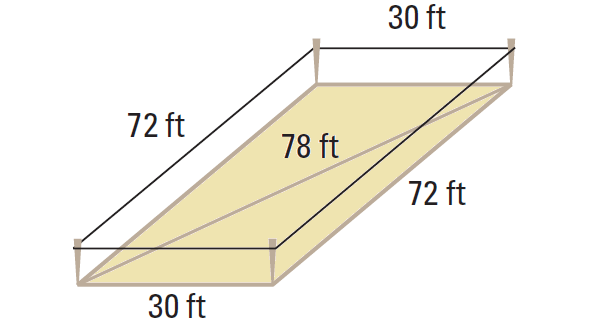
(i) John measures the four sides to be 30 feet, 30 feet, 72 feet, and 72 feet. He says these measurements prove the foundation is rectangular. Is he correct ?

(ii) David measures one of the diagonals to be 78 feet. Explain how David can use this measurement to tell whether the foundation will be rectangular.

Solution (i) :

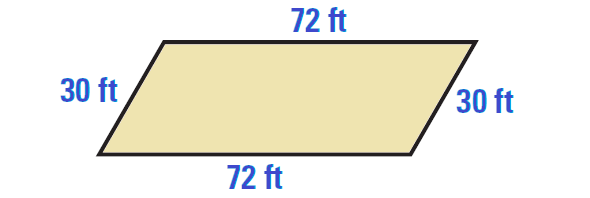
John is not correct. The foundation could be a nonrectangular parallelogram, as shown below.

Solution (ii) :

The diagonal divides the foundation into two triangles. Compare the square of the length of the longest side with the sum of the squares of the shorter sides of one of these triangles.

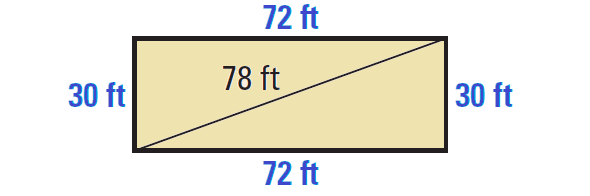
Because 30² + 72²  =  78², David can conclude that both the triangles are right triangles.

The foundation is a parallelogram with two right angles, which implies that it is rectangular.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.