TOTAL SURFACE ARE AOF COMBINED SOLID

Problem 1 :

A toy is in the form of right circular cylinder with a hemisphere at the one end and a cone at the other end. The base radius  measures 3.5 cm, height of the cylindrical portion is 10 cm. and conical part measures 12 cm. Find the total surface area of the toy(use π = 3.14)

Solution :

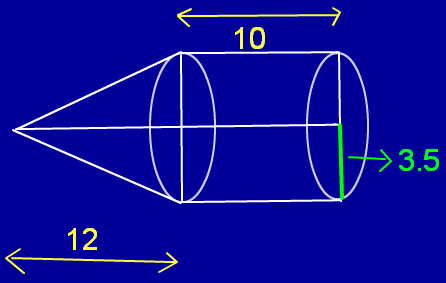
Radius of the hemisphere  =  3.5 cm

Curved Surface area (CSA) of the hemisphere

=  2πr²square units

=  2(3.5)²π

=  (24.5)π cm²

Radius of the Cylinder r = 3.5 cm

Height of the Cylinder h = 10 cm

Curved Surface Area (CSA) of the Cylinder

=  2πrh square units

=  2 π  x 3.5 x 10 

=  70π cm²

Radius of the cone r= 3.5 cm

Height of the cone h = 12 cm

Slant height of the cone l = √(r² + h²)

l  =  √(3.5² + 12²)

l = √(12.25+144)

 l = √(156.25)

l = 12.5 cm

Curved Surface-Area(CSA) of the Cone = πrl square units

=  π(3.5)(12.5)

= (43.75)π cm²

Total Surface-Area of the Toy

=  CSA of the hemisphere +   (CSA of the Cylinder) + (CSA of the Cone)

=  (24.5 π) + (70 π) + (43.75 π)

=  138.25  x 3.14

Hence, total surface area of the Toy = 434.11 cm²

Problem 2 :

Find the total surface area of cylinder whose height is 8 cm and radius is 4 cm.

Solution :

Radius of the Cylinder = 4 cm

Height of the cylinder = 8 cm

Required total surface area of the cylinder = 2πr(h+r)

=  2 π (4) (8+4)

=  2 π (4) (12)

=  96 π

Hence, total surface area of the Cylinder = 96π cm²

Problem 3 :

Find the total surface area of cylinder whose height is 16 cm and radius is 7 cm.

Solution :

Radius of the Cylinder = 7 cm

Height of the cylinder = 16 cm

Required Total surface area of the cylinder  =  2πr(h+r)

=  2 (22/7) (7) (16+7)

= 2 (22) (23)

=  1012 cm²

Hence, Total surface area of the Cylinder = 1012 cm²

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.