TRAPEZOIDS AND KITES

Trapezoids

A trapezoid is a quadrilateral with exactly one pair of parallel sides. The parallel sides are the bases. A trapezoid has two pairs of base angles.

For instance, in the trapezoid PQRS given below ∠S and ∠R.

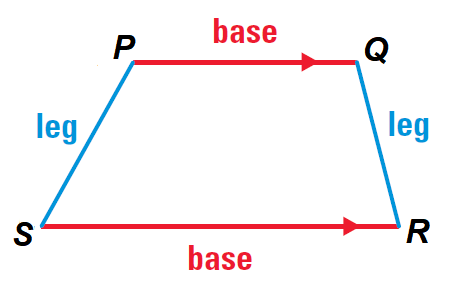
The other pair is ∠P and ∠Q. The non parallel sides are the legs of the trapezoid.

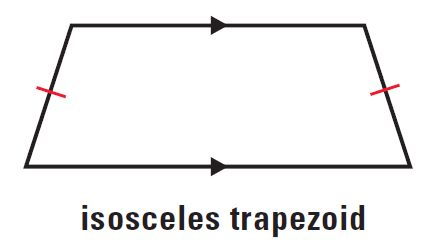
If the legs of a trapezoid are congruent, then the trapezoid is an isosceles trapezoid.

Kites

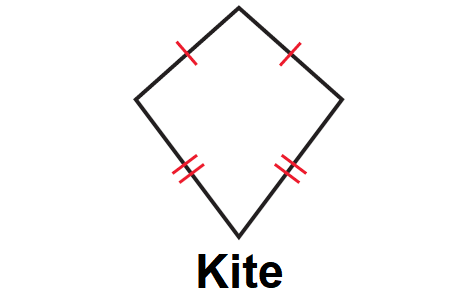
A kite is a quadrilateral that has two pairs of consecutive congruent sides, but opposite sides are not congruent.

Theorems on Trapezoids

Theorem 1 :

If a trapezoid is isosceles, then each pair of base angles is congruent.

It has been illustrated in the diagram given below.

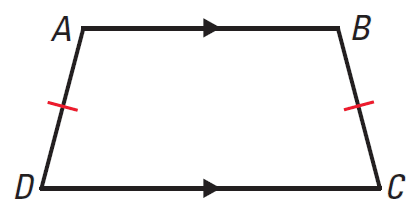
In the trapezoid ABCD above, we have

∠A ≅ ∠B,  ∠C ≅ ∠D

Theorem 2 :

If a trapezoid has a pair of congruent base angles, then it is an isosceles trapezoid.

It has been illustrated in the diagram given below.

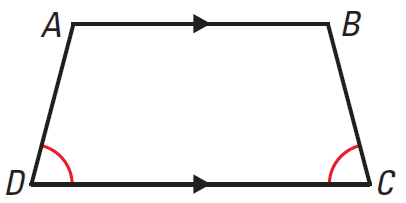
Trapezoid ABCD given above is an isosceles trapezoid. Because the base angles are congruent.

That is,

∠D ≅ ∠C

Theorem 3 :

A trapezoid is isosceles if and only if its diagonals are congruent.

It has been illustrated in the diagram given below.

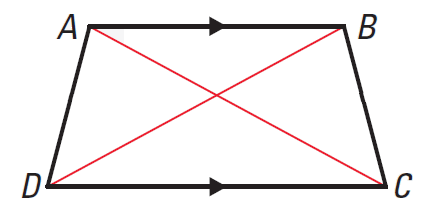
The trapezoid ABCD above can be isosceles, if and only if

AC ≅ BD

Midsegment of a Trapezoid

The midsegment of a trapezoid is the segment that connects the midpoints of its legs.

It has been illustrated in the diagram given below.

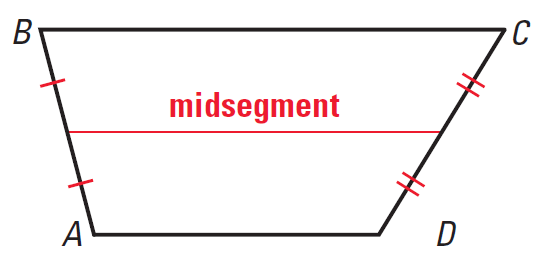
Midsegment Theorem for Trapezoids

The midsegment of a trapezoid is parallel to each base and its length is one half the sum of the lengths of the bases.

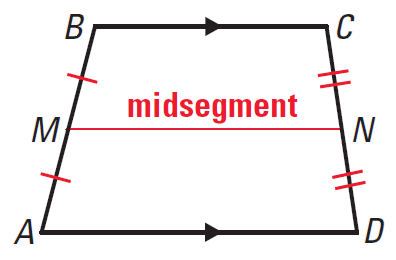
In the trapezoid ABCD given above, according to Midsegment Theorem for Trapezoids, we have

MN || AD

MN || BC

MN = 1/2 ⋅ (AD + BC)

Theorems on Kites

Theorem 1 :

If a quadrilateral is a kite, then its diagonals are perpendicular.

It has been illustrated in the diagram shown below.

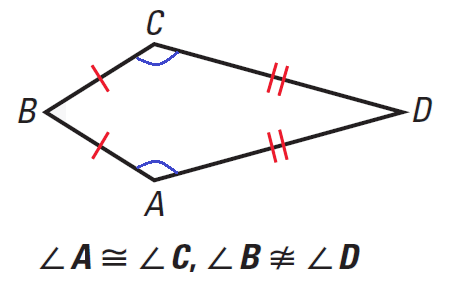
Theorem 2 :

If a quadrilateral is a kite, then exactly one pair of opposite angles are congruent.

It has been illustrated in the diagram shown below.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.