TRIANGLE CONGRUENCE AND SIMILARITY WORKSHEET

Problem 1 :

Check whether two triangles PQR and WXY are congruent.

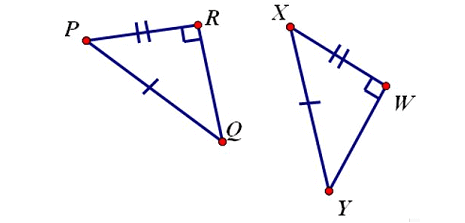
Problem 2 :

Check whether two triangles PQR and JKL are congruent.

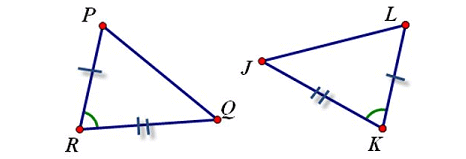
Problem 3 :

Check whether two triangles PQR and ABC are congruent.

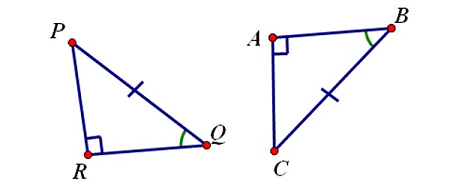
Problem 4 :

Check whether two triangles PQR and CDE are congruent.

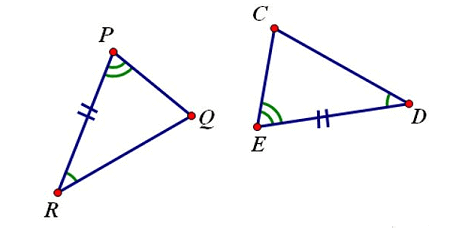
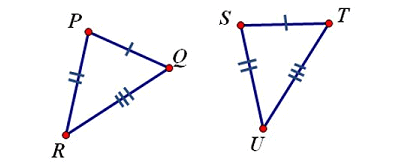
Problem 5 :

Check whether two triangles PQR and STU are congruent.

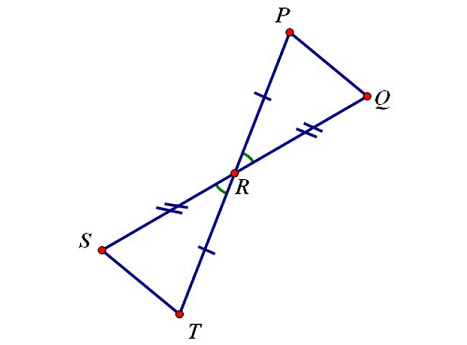
Problem 6 :

Check whether two triangles PQR and RST are congruent.

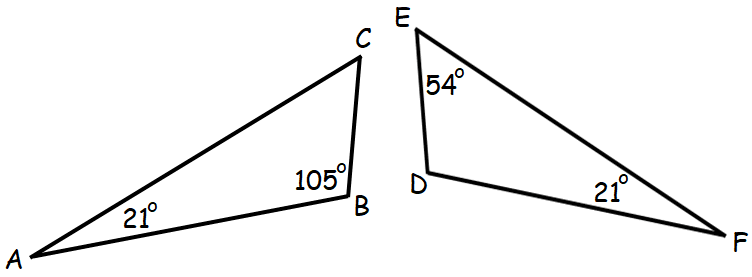
Problem 7 :

Check whether two triangles ABC and DEF are similar.

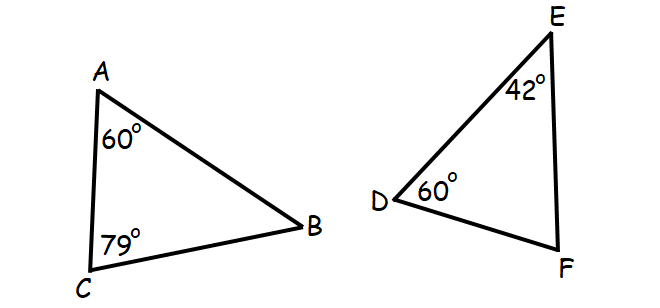
Problem 8 :

Determine whether the two triangles given below are similar. Justify your answer.

Problem 9 :

For what value of x is triangle ABC similar to triangle DEF.

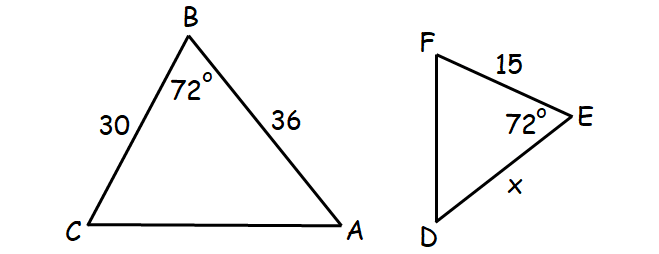
Problem 10 :

In the diagram given below, if AC and DE are parallel, find the value of h.

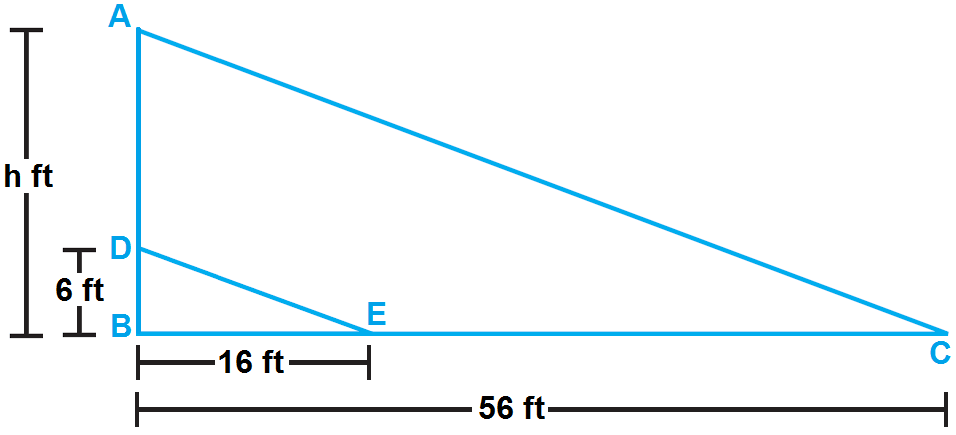
1. Answer :

(i) Triangle PQR and triangle WXY are right triangles. Because they both have a right angle.

(i) PQ = XY (Hypotenuse)

(ii) PR = WX (Leg)

Hence, the two triangles PQR and WXY are congruent by Hypotenuse-Leg theorem.

2. Answer :

(i) PR = LK (Given)

(ii) ∠R = ∠K (Given)

(i) RQ = JK (Given)

Hence, the two triangles PQR and JKL are congruent by SAS postulate.

3. Answer :

(i) PQ = BC (Hypotenuse)

(ii) ∠Q = ∠B (Acute angle)

Hence, the two triangles PQR and ABC are congruent by Hypotenuse-Acute Angle theorem.

4. Answer :

(i) ∠R = ∠D (Given)

(ii) PR = ED (Given)

(iii) ∠P = ∠E (Given)

Hence, the two triangles PQR and CDE are congruent by ASA postulate.

5. Answer :

(i) PQ = ST (Given)

(ii) PR = SU (Given)

(iii) QR = TU (Given)

Hence, the two triangles PQR and STU are congruent by SSS postulate.

6. Answer :

(i) PR = RT (Given)

(ii) ∠SRT = ∠PRQ (Vertical Angles)

(iii) QR = RS (Given)

Hence, the two triangles PQR and RST are congruent by SAS postulate.

7. Answer :

By Triangle Sum Theorem, in Δ ABC,

∠A + ∠B + ∠C = 180°

21° + 105° + ∠C = 180°

126° + ∠C = 180°

Subtract 126° from both sides.

∠C = 54°

In triangles ABC and DEF, we have

∠A = ∠F = 21°

∠E = ∠C = 54°

Two angles of one triangle are congruent to two angles of another triangle.

By Third Angle Theorem, the third pair of angles must also be congruent.

That is, 

∠B = ∠D = 105°

Hence, the triangles ABC and DEF are similar triangles.

8. Answer :

By Triangle Sum Theorem, in Δ ABC,

∠A + ∠B + ∠C = 180°

60° + ∠B + 79° = 180°

∠B + 139° = 180°

Subtract 139° from both sides.

∠B = 41°

By Triangle Sum Theorem, in Δ DEF,

∠D + ∠E + ∠F = 180°

60° + 42° + ∠F = 180°

102° + ∠F = 180°

Subtract 102° from both sides.

∠F = 78°

In triangles ABC and DEF, we have

∠A = ∠D = 60°

And no more pairs of angles are not congruent.

Hence, the triangles ABC and DEF are not similar triangles.

9. Answer :

Let us assume the given two triangles are similar.

So, the ratio of the corresponding sides must be equal.

By taking the vertices of the triangle in the order (given) ABC and DEF, we have

AB/DE = BC/EF

36/x = 30/15

Take reciprocal on both sides.

x/36 = 15/30

x/36 = 1/2

Multiply both sides by 36.

x = 1/2 ⋅ 36

x = 18

Hence the two triangles ABC and DEF are similar for the value of x is 18.

10. Answer :

In the above diagram, we can consider two triangles ABC and DBE.

Given : AC and DE are parallel.

The parallel sides AC and DE are cut by the transversal EC.

Then ∠E and ∠C are corresponding angles.

When two parallel lines cut by a transversal, the corresponding angles are congruent.

So, ∠E and ∠C are congruent.

In triangles ABC and DBE, we have

∠E = ∠C

∠B is common angle

Two angles of one triangle are congruent to two angles of another triangle.

By Third Angle Theorem, the third pair of angles must also be congruent.

So, the triangles ABC and DBE are similar triangles.

In similar triangles, the ratio of the corresponding sides are equal.

Then, we have

AB/DB = BC/BE

Substitute AB = h, DB = 6, BE = 16 and BC = 56.

h/6 = 56/16

Multiply both sides by 6.

h = (56/16) ⋅ 6

 h = 21

Hence, the value of h is 21.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.