TRIANGLE CONGRUENCE POSTULATES WORKSHEET
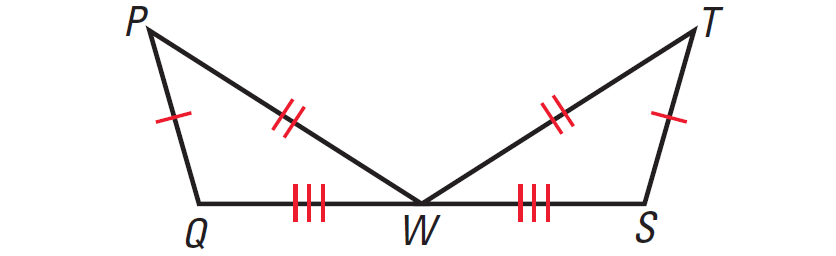
1. In the diagram below, prove that ΔPQW ≅ ΔTSW.
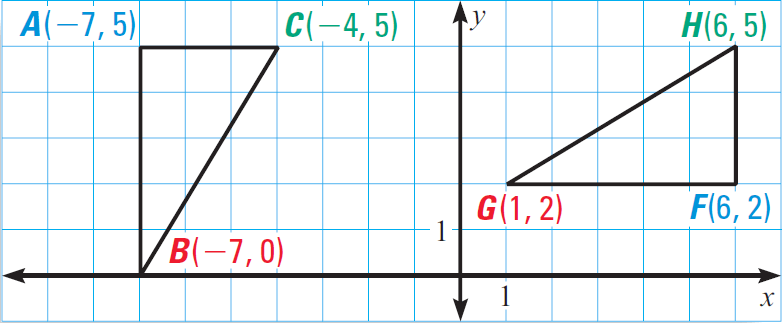
2. In the diagram below, prove that ΔABC ≅ ΔFGH.
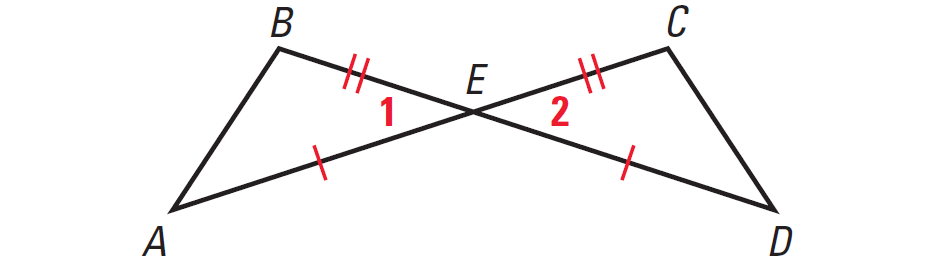
3. In the diagram below, prove that ΔAEB ≅ ΔDEC.
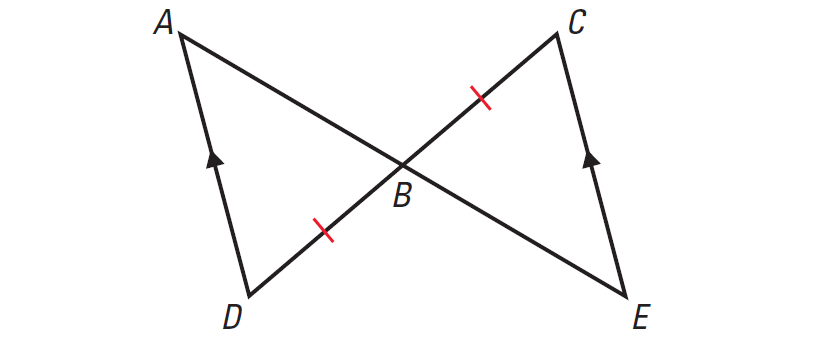
4. In the diagram below, prove that ΔABD ≅ ΔEBC.
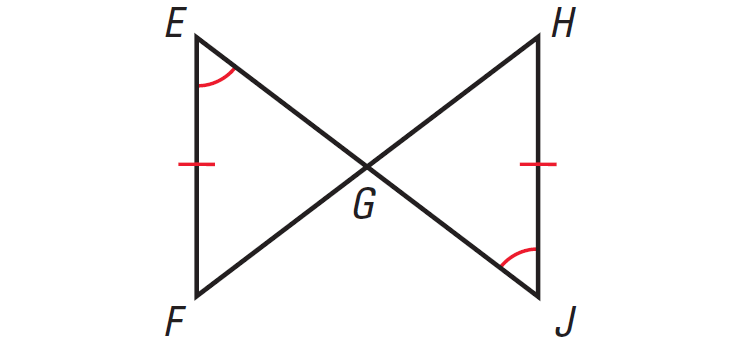
5. In the diagram below, prove that ΔEFG ≅ ΔJHG.
1. Answer :
|
Statements PQ ≅ ST PW ≅ TW QW ≅ SW ΔPQW ≅ ΔTSW |
Reasons Given Given Given SSS Congruence Postulate |
2. Answer :
Because AB = 5 in triangle ABC and FG = 5 in triangle FGH,
AB ≅ FG
Because AC = 3 in triangle ABC and FH = 3 in triangle FGH,
AC ≅ FH
Use the distance formula to find the lengths of BC and GH.
Length of BC :
BC = √[(x2 - x1)2 + (y2 - y1)2]
Substitute (x1, y1) = B(-7, 0) and (x2, y2) = C(-4, 5).
BC = √[(-4 + 7)2 + (5 - 0)2]
= √[32 + 52]
= √[9 + 25]
= √34
Length of GH :
GH = √[(x2 - x1)2 + (y2 - y1)2]
Substitute (x1, y1) = B(1, 2) and (x2, y2) = C(6, 5).
GH = √[(6 - 1)2 + (5 - 2)2]
= √[52 + 32]
= √[25 + 9]
= √34
Conclusion :
Because BC = √34 and GH = √34,
BC ≅ GH
All the three pairs of corresponding sides are congruent. By SSS congruence postulate,
ΔABC ≅ ΔFGH
3. Answer :
|
Statements AE ≅ DE, BE ≅ CE ∠1 ≅ ∠2 ΔAEB ≅ ΔDEC |
Reasons Given Vertical Angles Theorem SAS Congruence Postulate |
4. Answer :
|
Statements BD ≅ BC AD || EC ∠D ≅ ∠C ∠ABD ≅ ∠EBC ΔABD ≅ ΔEBC |
Reasons Given Given Alternate Interior Angles Theorem Vertical Angles Theorem ASA Congruence Postulate |
5. Answer :
|
Statements FE ≅ JH ∠E ≅ ∠J ∠EGF ≅ ∠JGH ΔEFG ≅ ΔJHG |
Reasons Given Given Vertical Angles Theorem AAS Congruence Postulate |
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
De Moivre's Theorem and Its Applications
Apr 19, 24 08:30 AM
De Moivre's Theorem and Its Applications -
First Fundamental Theorem of Calculus - Part 1
Apr 17, 24 11:27 PM
First Fundamental Theorem of Calculus - Part 1 -
Polar Form of a Complex Number
Apr 16, 24 09:28 AM
Polar Form of a Complex Number
